A cart moves with a constant speed along a horizontal circular path. From the cart, a particle is thrown up vertically with respect to the cart.
The particle will land somewhere on the circular path
The particle will land outside the circular path
The particle will follow an elliptical path
The particle will follow a parabolic path
The correct Answer is:BD
To solve the problem of a cart moving with constant speed along a horizontal circular path and a particle being thrown vertically from the cart, we can follow these steps:
Step 1: Understand the Motion of the Cart
The cart is moving in a circular path with constant speed. This means that while the speed is constant, the direction of the velocity is continuously changing. The velocity of the cart at any point is tangent to the circular path.
Hint: Remember that in circular motion, even though speed is constant, the direction of velocity changes, resulting in centripetal acceleration.
Step 2: Analyze the Particle's Motion
When the particle is thrown vertically upwards from the cart, it has two components of motion:
- A horizontal component (Vx), which is the same as the speed of the cart at the moment of the throw.
- A vertical component (Vy), which is the speed at which the particle is thrown upwards.
Hint: Consider the initial velocity of the particle as a vector sum of its horizontal and vertical components.
Step 3: Determine the Path of the Particle
Since the particle is thrown upwards, it will experience projectile motion. The horizontal motion will be uniform (constant velocity), while the vertical motion will be influenced by gravity, resulting in a parabolic trajectory.
Hint: Recall that projectile motion consists of two independent motions: horizontal (constant velocity) and vertical (accelerated motion due to gravity).
Step 4: Identify the Resultant Path
The resultant path of the particle will be a parabola because it is subject to gravitational acceleration while moving horizontally at the speed of the cart. As the particle moves upward and then downward, it will not follow the circular path of the cart.
Hint: Visualize the trajectory of the particle as a parabola opening downwards, starting from the point where it was thrown.
Step 5: Determine Where the Particle Lands
Since the particle is thrown from a moving cart, it will land outside the circular path traced by the cart. The horizontal distance covered by the particle will depend on the time it takes to reach the ground, which is influenced by the initial vertical velocity and the acceleration due to gravity.
Hint: Consider the time of flight and the horizontal distance traveled to conclude where the particle will land relative to the circular path.
Conclusion
Based on the analysis:
- The path of the particle will be parabolic (Option D).
- The particle will land outside the circular path (Option B).
Thus, the correct answers are Options B and D.
---
The cart is moving in a circular path with constant speed. This means that while the speed is constant, the direction of the velocity is continuously changing. The velocity of the cart at any point is tangent to the circular path.
When the particle is thrown vertically upwards from the cart, it has two components of motion:
- A horizontal component (Vx), which is the same as the speed of the cart at the moment of the throw.
- A vertical component (Vy), which is the speed at which the particle is thrown upwards.
Since the particle is thrown upwards, it will experience projectile motion. The horizontal motion will be uniform (constant velocity), while the vertical motion will be influenced by gravity, resulting in a parabolic trajectory.
The resultant path of the particle will be a parabola because it is subject to gravitational acceleration while moving horizontally at the speed of the cart. As the particle moves upward and then downward, it will not follow the circular path of the cart.
Since the particle is thrown from a moving cart, it will land outside the circular path traced by the cart. The horizontal distance covered by the particle will depend on the time it takes to reach the ground, which is influenced by the initial vertical velocity and the acceleration due to gravity.
Based on the analysis:
- The path of the particle will be parabolic (Option D).
- The particle will land outside the circular path (Option B).
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Main (Archive)19videos
Motion in Two Dimensions
- BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Advanced (Archive)19videos
Motion in Two Dimensions
- BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - Long Answer Type5videos
Motion in Two Dimensions
- BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-J10videos
Motion in Straight Line
- BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K10videos
MOVING CHARGES & MAGNETISM
Similar Questions
A particle is moving with a constant speed along a straight line path. A force is not required to
View SolutionA particle moves with constant speed v along a circular path of radius r and completes the circle in time T. The acceleration of the particle is
View SolutionA particle of mass M moves with constant speed along a circular path of radius r under the action of a force F. Its speed is
View SolutionA cart loaded with sand moves along a horizontal floor due to a constant force F coinciding in direction with cart's velocity vector. In the process, the sand spills through a hole in the bottom with a constant rate μkg/s. Find the acceleration and velocity of the cart at the moment t, if at the initial moment t=0 the cart with loaded sand had mass m0 and its velocity was equal to zero. Friction is to be neglected.
View SolutionIn the figure shown, the cart of mass 6m is initially at rest. A particle of mass in is attached to the end of the light rod which can rotate freely about A. If the rod is released from rest in a horizontal position shown, determine the velocity vrel of the particle with respect to the cart when the rod is vertical.
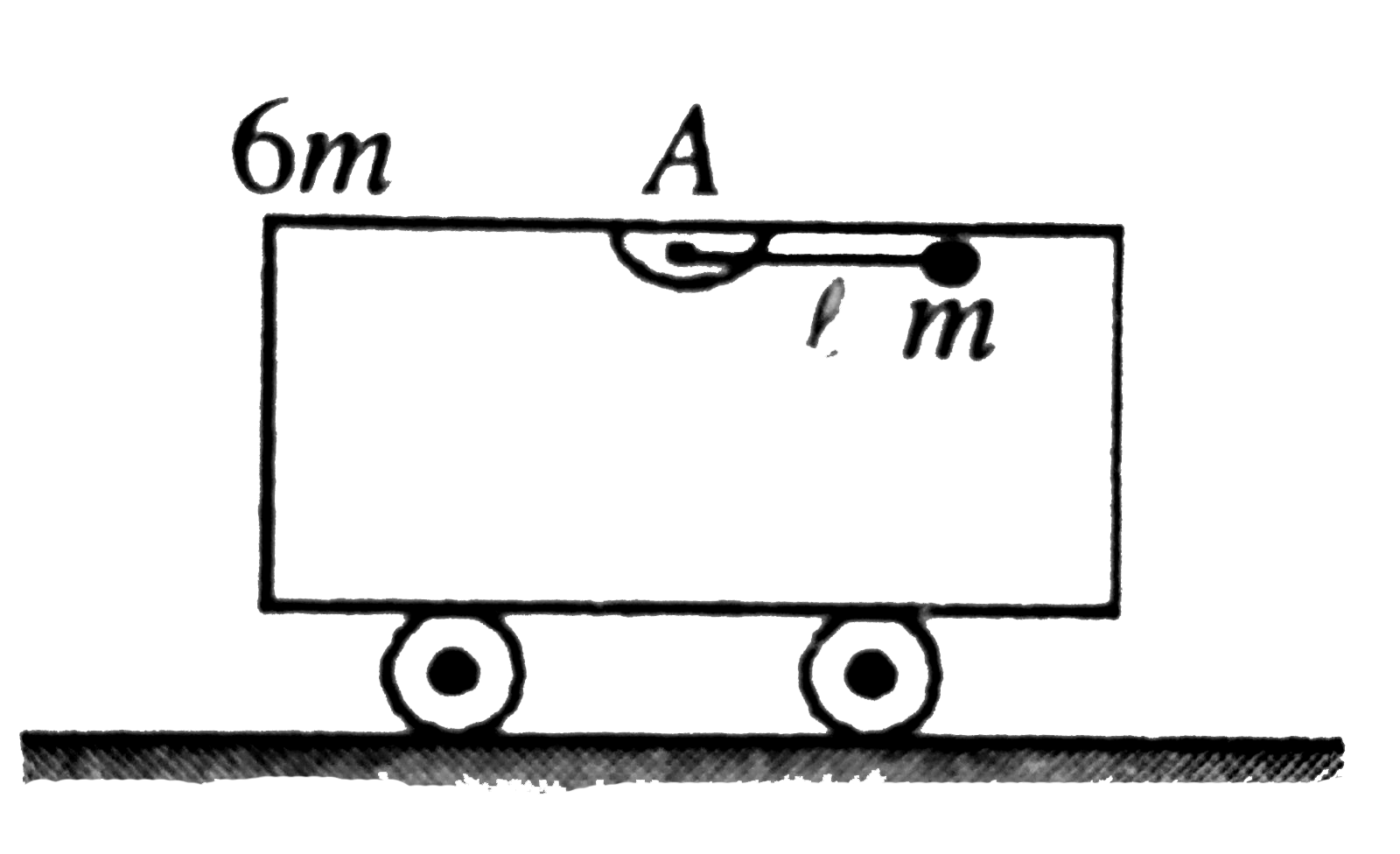 View Solution
View SolutionA heavy cart is pulled by a constant force F along a horizontal track with the help of a rope that passes over a fixed pulley, as shown in the figure. Assume the tension in the rope and the frictional forces on the cart remain constant and consider motion of the cart until it reaches vertically below the pulley. As the cart moves to the right, its acceleration
 View Solution
View SolutionAn object moving in a circular path at constant speed has constant
View SolutionSand is falling on a flat car being pulled with constant speed. The rate of mass falling on the cart is constant. Then the horizontal component of force exerted by the falling sand on the cart (sand particles sticks to the cart)
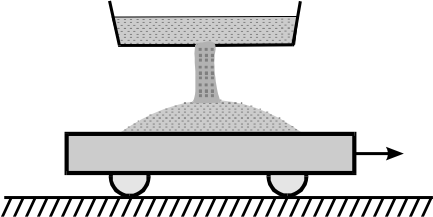 View Solution
View SolutionA cart is moving horizontally along a straight line with constant speed 30ms−1. A particle is to be fired vertically upwards from the moving cart in such a way that it returns to the cart at the same point from where it was projected after the cart has moved 80m. At what speed (relative to the cart) must the projectile be fired? (Take g=10ms−2)
View SolutionA cart is moving horizontally along a straight line with constant speed 30ms−1. A particle is to be fired vertically upwards from the moving cart in such a way that it returns to the cart at the same point from where it was projected after the cart has moved 80m. At what speed (relative to the cart) must the projectile be fired? (Take g=10ms−2)
View Solution

