Two plane mirrors are inclined at 70∘. A ray incident on one mirror at incidence angle θ after reflection falls on the second mirror and is reflected from there parallel to the first mirror, The value of θ is
50∘
45∘
30∘
55∘
The correct Answer is:A
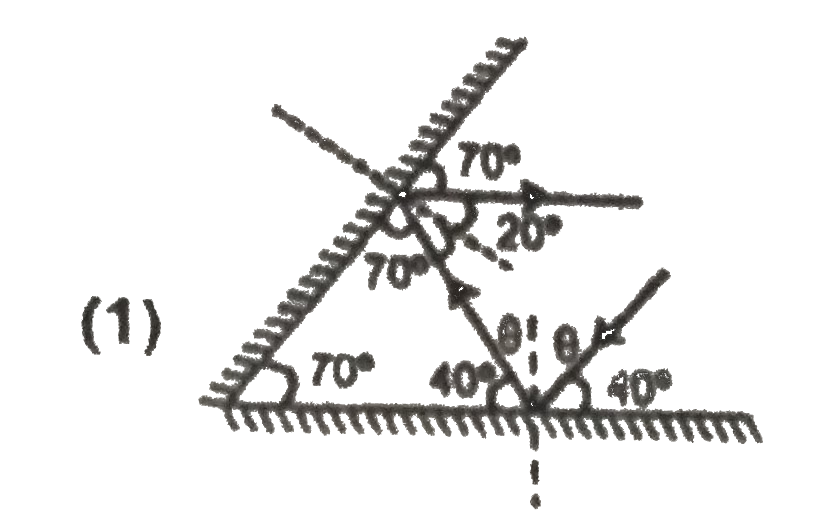
From the diagram θ=50∘
Topper's Solved these Questions
Similar Questions
Two plane mirrors are inclined to each other such that a ray of light incident on the first mirror and parallel to the second is reflected from the second mirror parallel to the first mirror. Determine the angle between the two mirrors:
View SolutionTwo plane mirror M1andM2 are inclined to each other at 70∘. A ray incident on the mirror M1 at an angle θ fallls on M2 and is then reflected parallel to M1 for
View Solution
Knowledge Check
The angle of incidence on a plane mirror is 30∘. The angle of reflection will be:
A30∘B60∘C15∘D0∘
Two plane mirrors are inclined to each other at an angle 60∘ if a ray of light incident on first mirror parallel to the second mirror, it is reflected from the second mirror
View SolutionFind the angle between two plane mirrors such that a ray of light incident on the first mirror and parallel to the second mirror is reflected from the second mirror, parallel to the first mirror.
View SolutionTwo plane mirrror are inclined to each other at 70∘. A ray of light is incident on one mirror. They ray after two reflections will undergo a total deviation of :
View SolutionTwo plane mirror are inclined to each other at 90∘. A ray of light is incident on one mirror. The ray will undergo a total deviation of
View SolutionTwo plane mirrors at an angle such that a ray incident on a mirror undergoes a total deviation of 240∘ after two reflections:
View SolutionRays of light are incident on a plane mirror at 40∘. At what angle with the first should a second mirror be placed such that the rays emerge from the second mirror parallel to the first mirror.
View SolutionTwo plane mirrors are inclined to each other at an angle θ. A ray of light is reflected at one
mirror and then at the other. Find the total deviation of the ray.
View Solution
