One mole of an ideal monoatomic gas is mixed with one mole of an equimolar mixture of monoatomic and diatomic ideal gases. Find the value of λ=(CPCv) for the final mixture
87
1912
117
85
The correct Answer is:C
To find the value of λ=CPCV for the final mixture of gases, we need to calculate the specific heat capacities CP and CV for the mixture.
Step 1: Identify the specific heat capacities for each gas type
1. Monoatomic gas:
- CP=5R2
- CV=3R2
2. Diatomic gas:
- CP=7R2
- CV=5R2
Step 2: Calculate the total CP for the mixture
We have:
- 1 mole of monoatomic gas
- 1 mole of an equimolar mixture of monoatomic and diatomic gases (which means 0.5 moles of each).
The total CP for the mixture can be calculated as follows:
CP=CP,monoatomic+CP,mixture
Where,
CP,mixture=(0.5×CP,monoatomic)+(0.5×CP,diatomic)
Substituting the values:
CP,mixture=(0.5×5R2)+(0.5×7R2)
Calculating:
CP,mixture=5R4+7R4=12R4=3R
Thus, the total CP for the mixture is:
CP=5R2+3R=5R2+6R2=11R2
Step 3: Calculate the total CV for the mixture
Similarly, we calculate CV:
CV=CV,monoatomic+CV,mixture
Where,
CV,mixture=(0.5×CV,monoatomic)+(0.5×CV,diatomic)
Substituting the values:
CV,mixture=(0.5×3R2)+(0.5×5R2)
Calculating:
CV,mixture=3R4+5R4=8R4=2R
Thus, the total CV for the mixture is:
CV=3R2+2R=3R2+4R2=7R2
Step 4: Calculate λ
Now we can find λ:
λ=CPCV=11R27R2=117
Final Answer
Thus, the value of λ for the final mixture is:
λ=117
1. Monoatomic gas:
- CP=5R2
- CV=3R2
- CP=7R2
- CV=5R2
We have:
- 1 mole of monoatomic gas
- 1 mole of an equimolar mixture of monoatomic and diatomic gases (which means 0.5 moles of each).
CP=CP,monoatomic+CP,mixture
Where,
CP,mixture=(0.5×CP,monoatomic)+(0.5×CP,diatomic)
Substituting the values:
CP,mixture=(0.5×5R2)+(0.5×7R2)
Calculating:
CP,mixture=5R4+7R4=12R4=3R
Thus, the total CP for the mixture is:
CP=5R2+3R=5R2+6R2=11R2
Similarly, we calculate CV:
CV=CV,monoatomic+CV,mixture
Where,
CV,mixture=(0.5×CV,monoatomic)+(0.5×CV,diatomic)
Substituting the values:
CV,mixture=(0.5×3R2)+(0.5×5R2)
Calculating:
CV,mixture=3R4+5R4=8R4=2R
Thus, the total CV for the mixture is:
CV=3R2+2R=3R2+4R2=7R2
Now we can find λ:
λ=CPCV=11R27R2=117
Thus, the value of λ for the final mixture is:
λ=117
Topper's Solved these Questions
Similar Questions
One mole of an ideal monoatomic gas is mixed with one mole of an ideal diatomic gas. The molar specific heat of the mixture at constant volume is (in Calories )
View SolutionIf one mole each of a monoatomic and diatomic gases are mixed at low temperature then Cp/Cv ratio for the mixture is :
View SolutionWhen 1 mole of monoatomic gas is mixed with 2 moles of diatomic gas, then find gamma for the mixture of gases.
View SolutionFor one mole of a monoatomic gas match the following.
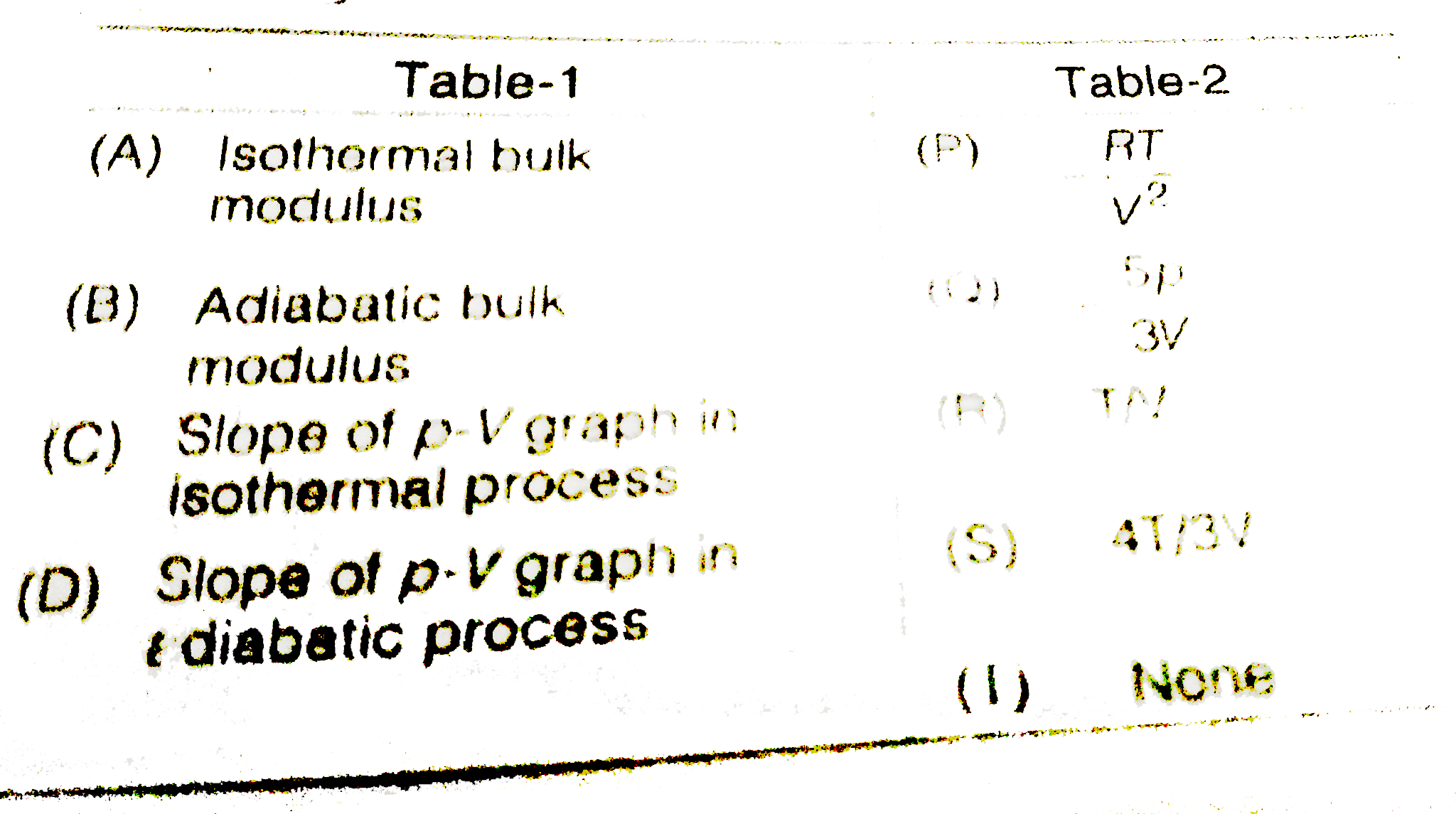 View Solution
View SolutionOne mole of a mono-atomic ideal gas is mixed with one mole of a diatomic ideal gas. The molar specific heat of the mixture at constant volume is ……
View SolutionOne moles of a monatomic gas is mixed with three moles of a diatomic gas. The molar specific heat of the mixture at constant volume is
View SolutionOne gram mole of a monoatomic gas occupies 22.4 L at S.T.P
View SolutionIf one mole of a monatomic gas (γ=53) is mixed with one mole of a diatomic gas (γ=75), the value of gamma for mixture is
View SolutionIf one mole of a monatomic gas (γ=53) is mixed with one mole of a diatomic gas (γ=75), the value of gamma for mixture is
View SolutionIf α moles of a monoatomic gas are mixed with β moles of a polyatomic gas and mixture behaves like diatomic gas, then [neglect the vibration mode of freedom]
View Solution

