A and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. The ratio AXBX is
2√2−12√2+1
√3−1√3+1
3√2−23√2+2
13
The correct Answer is:A
To solve the problem, we need to find the ratio AXBX where A and B are points on the path of a particle executing Simple Harmonic Motion (SHM) and the velocity is zero at these points. Given that the particle crosses point X at intervals of 2 seconds and 4 seconds with a speed of 5 m/s, we can proceed as follows:
1. Understanding the Motion:
- Points A and B are the extreme positions of the SHM where the velocity is zero.
- The particle crosses point X at intervals of 2 seconds and 4 seconds, indicating its motion back and forth.
2. Determine the Time Period:
- The total time taken to go from X back to X after reaching point A and then B is 2+4=6 seconds.
- Therefore, the time period T of the SHM is 6 seconds.
3. Calculate Half the Time Period:
- The time taken to move from the mean position (equilibrium) to point A or B is half of the time period:
T2=62=3 seconds
4. Determine Time to Reach Point X:
- Let t0 be the time taken to go from the mean position to point X. The total time to go from the mean position to A and back to X is:
t0+t0+3=4 seconds
- Simplifying gives:
2t0+3=4⟹2t0=1⟹t0=12 seconds
5. Calculate the Angular Frequency:
- The angular frequency ω is given by:
ω=2πT=2π6=π3 rad/s
6. Calculate the Displacement from Mean Position:
- The displacement x from the mean position after t0 seconds is:
x=Asin(ωt0)=Asin(π3⋅12)=Asin(π6)=A⋅12
7. Express AX and BX:
- The distances from the mean position to points A and B are:
AX=A−xandBX=A+x
- Substituting for x:
AX=A−A2=A2andBX=A+A2=3A2
8. Calculate the Ratio:
- Now, we can find the ratio AXBX:
AXBX=A23A2=13
Final Answer:
The ratio AXBX is 13.
- Points A and B are the extreme positions of the SHM where the velocity is zero.
- The particle crosses point X at intervals of 2 seconds and 4 seconds, indicating its motion back and forth.
- The total time taken to go from X back to X after reaching point A and then B is 2+4=6 seconds.
- Therefore, the time period T of the SHM is 6 seconds.
- The time taken to move from the mean position (equilibrium) to point A or B is half of the time period:
T2=62=3 seconds
- Let t0 be the time taken to go from the mean position to point X. The total time to go from the mean position to A and back to X is:
t0+t0+3=4 seconds
- Simplifying gives:
2t0+3=4⟹2t0=1⟹t0=12 seconds
- The angular frequency ω is given by:
ω=2πT=2π6=π3 rad/s
- The displacement x from the mean position after t0 seconds is:
x=Asin(ωt0)=Asin(π3⋅12)=Asin(π6)=A⋅12
- The distances from the mean position to points A and B are:
AX=A−xandBX=A+x
- Substituting for x:
AX=A−A2=A2andBX=A+A2=3A2
- Now, we can find the ratio AXBX:
AXBX=A23A2=13
The ratio AXBX is 13.
Similar Questions
A and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. Amplitude of oscillation isView SolutionX1 and X2 are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X(X1X<X2X) then particle crosses this point again at successive intervals of 2s and 4s with a speed of 5m/s.
The time period of SHM isView SolutionA particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P(APltBP) at successive intervals of 0.5s and 1.5 s with a speed of 3m/s.
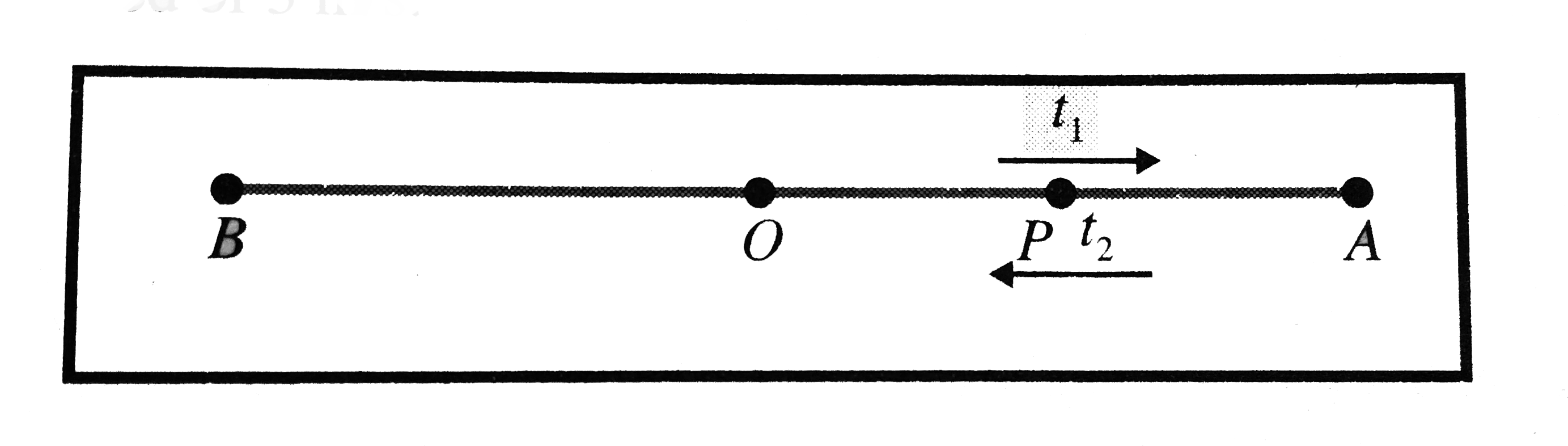
View SolutionA particle starts SHM from mean position O executing SHM A and B are the two point at which its velocity is zero . It passes through a certain point P at time t1=0.5andt2=1.5s with a speed of 3m/s.
i. The maximum speed .......
ii. ratio AP/PB ....
 .View Solution
.View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionIf the velocity v of a particle moving along a straight line and its distance s from a fixed point on the line are related by v2=a2+s2, then its acceleration equals
View SolutionThe period of a particle executing SHM is T. There is a point P at a distance 'x' from the mean position 'O'. When the particle passes P towards OP, it has speed v. Find the time in which it returns to P again.
View SolutionVelocity time equation of a particle moving in a straight line is v=2t−4 for t≤2s and v=4−2t for t>2.The distance travelled by the particle in the time interval from t=0 to t=4s is (Here t is in second and v in m/s)
View SolutionA particle is moving in a straight line such that the distance covered by it in t seconds from a point is (t33−t) cm. find its speed at t=3 seconds.
View Solution

