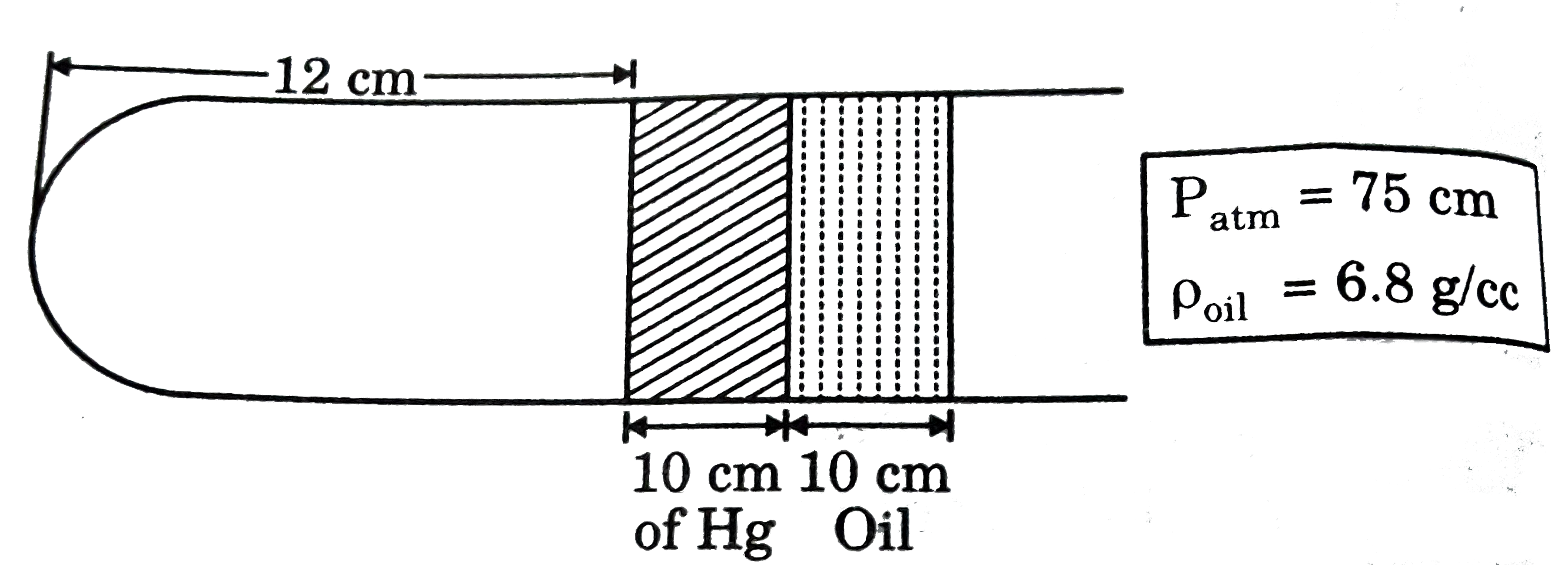
A column of mercury is in the middle of horizontal tube of uniform cross section closed at both ends. The pressure of the enclosed air column on either side of the mercury is 75 cm of mercury column. When the tuve is placed vertical the length air below the mercury column is 35 th of that above it. if length of mercury column is 10n cm. find n.
The correct Answer is:4
To solve the problem, we will follow these steps:
Step 1: Understand the Setup
We have a horizontal tube with a mercury column in the middle. The pressure of the air on both sides of the mercury column is given as 75 cm of mercury. When the tube is placed vertically, the lengths of the air columns below and above the mercury are in the ratio 35.
Step 2: Define Variables
Let:
- x = length of the mercury column (in cm)
- L = total length of the air column (in cm)
- The length of the air column below the mercury = L−x2−y
- The length of the air column above the mercury = L−x2+y
Step 3: Set Up the Ratio
According to the problem, the length of air below the mercury is 35 of the length above it. Thus, we can write:
L−x2−y=35(L−x2+y)
Step 4: Simplify the Equation
Cross-multiplying gives:
5(L−x2−y)=3(L−x2+y)
Expanding both sides:
5⋅L−x2−5y=3⋅L−x2+3y
Rearranging gives:
5⋅L−x2−3⋅L−x2=5y+3y
2(L−x)2=8y
L−x=8y
Step 5: Express y in Terms of L and x
From the equation L−x=8y, we can express y:
y=L−x8
Step 6: Relate Pressures
Using the ideal gas law for both sides of the mercury column, we can write:
- For the air column above the mercury:
P0⋅(L−x2+y)=P1⋅(L−x2−y)
Step 7: Substitute y
Substituting y=L−x8 into the pressure equation:
P0⋅(L−x2+L−x8)=P1⋅(L−x2−L−x8)
Step 8: Solve for P0 and P1
After simplifying, we can find a relationship between P0 and P1.
Step 9: Use Weight of Mercury
The weight of the mercury column provides another equation:
P1A−P0A=ρgx
Where ρ is the density of mercury and g is the acceleration due to gravity.
Step 10: Solve for x
Using the relationships derived from pressures and the weight of the mercury, we can solve for x in terms of known quantities.
Step 11: Substitute Known Values
Given that P0=75 cm of mercury, we can substitute this value into our equations to find x.
Step 12: Find n
Finally, since x=10n, we can solve for n.
Final Calculation
After performing the calculations, we find that n=4.
We have a horizontal tube with a mercury column in the middle. The pressure of the air on both sides of the mercury column is given as 75 cm of mercury. When the tube is placed vertically, the lengths of the air columns below and above the mercury are in the ratio 35.
Let:
- x = length of the mercury column (in cm)
- L = total length of the air column (in cm)
- The length of the air column below the mercury = L−x2−y
- The length of the air column above the mercury = L−x2+y
According to the problem, the length of air below the mercury is 35 of the length above it. Thus, we can write:
L−x2−y=35(L−x2+y)
Cross-multiplying gives:
5(L−x2−y)=3(L−x2+y)
Expanding both sides:
5⋅L−x2−5y=3⋅L−x2+3y
Rearranging gives:
5⋅L−x2−3⋅L−x2=5y+3y
2(L−x)2=8y
L−x=8y
From the equation L−x=8y, we can express y:
y=L−x8
Using the ideal gas law for both sides of the mercury column, we can write:
- For the air column above the mercury:
P0⋅(L−x2+y)=P1⋅(L−x2−y)
Substituting y=L−x8 into the pressure equation:
P0⋅(L−x2+L−x8)=P1⋅(L−x2−L−x8)
After simplifying, we can find a relationship between P0 and P1.
The weight of the mercury column provides another equation:
P1A−P0A=ρgx
Where ρ is the density of mercury and g is the acceleration due to gravity.
Using the relationships derived from pressures and the weight of the mercury, we can solve for x in terms of known quantities.
Given that P0=75 cm of mercury, we can substitute this value into our equations to find x.
Finally, since x=10n, we can solve for n.
After performing the calculations, we find that n=4.
Similar Questions
Knowledge Check
A thin tube of uniform cross-section is sealed at both ends. It lies horizontally, the middle 5 cm containing mercury and the two equal end containing air at the same pressure P. When the tube is held at an angle of 60∘ with the vetical direction, the length of the air column above and below the mercury column are 46 cm and 44.5 cm respectively. Calculate the pressure P in centimeters of mercury. (The temperature of the system is kept at 30∘C).
A75.4B45.8C67.5D89.3
A barometer tube reads 76 cm of mercury. If the tube is gradually inclined at an angle of 60 with vertical, keeping the open end immersed in the mercury reservoir, the length of the mercury column will be
View SolutionA barometer tube reads 76 cm of mercury. If the tube is gradually inclined keeping the open end immersed in the mercury reservoir, will the length of mercury column be 76 cm, more than 76 cm or less than 76 cm?
View SolutionThe length of an air column trapped in a capillary tube by means of a mercury pellet is 5 cm at 30∘C. If the tube is heated uniformly to 80∘C, the new length of air column is :
View SolutionExplain how is the height of mercury column in the tube of a simple barometer, a measure of the atmospheric pressure.
View SolutionA thin tube of uniform cross-section is sealed at both ends. It lies horizontally, the middle 5 cm containing mercury and the two equal end containing air at the same pressure P. When the tube is held at an angle of 60∘ with the vetical direction, the length of the air column above and below the mercury column are 46 cm and 44.5 cm respectively. Calculate the pressure P in centimeters of mercury. (The temperature of the system is kept at 30∘C).
View SolutionThe readings of a thermometer at 0∘C and 100∘C are 50cm and 75cm of mercury column respectively. Find the temperature at which its reading is 80cm of mercury column ?
View SolutionThe readings of a thermometer at 0∘C and 100∘C are 50cm and 75cm of mercury column respectively. Find the temperature at which its reading is 80cm of mercury column ?
View Solution