A complex containing K+,Pt(IV) and Cl−− is 100% ionised giving i=3. Thus, complex is:
K2[PtCl4]
K2[PtCl6]
K43[PtCl5]
K[PtCl3]
The correct Answer is:B
To solve the problem, we need to identify the complex that contains K+, Pt(IV), and Cl−− and results in 100% ionization with i=3.
1. Understanding Ionization:
- The ionization factor i indicates the number of particles (ions) produced when the complex dissociates in solution. Given i=3, the complex must yield 3 ions upon dissociation.
2. Identifying Possible Complexes:
- We have the following complexes to consider:
1. K2(PtCl4)
2. K2(PtCl6)
3. K2(PtCl5)
4. K(PtCl3)
3. Calculating Ionization for Each Complex:
- For K2(PtCl4):
- Dissociates into 2K++(PtCl4)2− → Total ions = 3
- For K2(PtCl6):
- Dissociates into 2K++(PtCl6)2− → Total ions = 3
- For K2(PtCl5):
- Dissociates into 2K++(PtCl5)− → Total ions = 3
- For K(PtCl3):
- Dissociates into K++(PtCl3)− → Total ions = 2
4. Determining the Oxidation State of Platinum:
- For K2(PtCl4):
- Let the oxidation state of Pt be x.
- Equation: x+4(−1)=−2 → x=+2
- For K2(PtCl6):
- Equation: x+6(−1)=−2 → x=+4
- For K2(PtCl5):
- Equation: x+5(−1)=−2 → x=+3
- For K(PtCl3):
- Equation: x+3(−1)=0 → x=+3
5. Identifying the Correct Complex:
- The only complex that has Pt(IV) (i.e., +4 oxidation state) and gives i=3 is K2(PtCl6).
Conclusion:
The complex is K2(PtCl6).
- The ionization factor i indicates the number of particles (ions) produced when the complex dissociates in solution. Given i=3, the complex must yield 3 ions upon dissociation.
- We have the following complexes to consider:
1. K2(PtCl4)
2. K2(PtCl6)
3. K2(PtCl5)
4. K(PtCl3)
- For K2(PtCl4):
- Dissociates into 2K++(PtCl4)2− → Total ions = 3
- For K2(PtCl6):
- Dissociates into 2K++(PtCl6)2− → Total ions = 3
- For K2(PtCl5):
- Dissociates into 2K++(PtCl5)− → Total ions = 3
- For K(PtCl3):
- Dissociates into K++(PtCl3)− → Total ions = 2
- For K2(PtCl4):
- Let the oxidation state of Pt be x.
- Equation: x+4(−1)=−2 → x=+2
- For K2(PtCl6):
- Equation: x+6(−1)=−2 → x=+4
- For K2(PtCl5):
- Equation: x+5(−1)=−2 → x=+3
- For K(PtCl3):
- Equation: x+3(−1)=0 → x=+3
- The only complex that has Pt(IV) (i.e., +4 oxidation state) and gives i=3 is K2(PtCl6).
The complex is K2(PtCl6).
Topper's Solved these Questions
- SOLUTIONSBOOK - RESONANCE ENGLISHCHAPTER - SOLUTIONSEXERCISE - Advabced Level Problems (PART-2)
35 Videos
- SOLUTIONSBOOK - RESONANCE ENGLISHCHAPTER - SOLUTIONSEXERCISE - EXERCISE-3(PART-3)
30 Videos
- SOLUTION AND COLLIGATIVE PROPERTIESBOOK - RESONANCE ENGLISHCHAPTER - SOLUTION AND COLLIGATIVE PROPERTIESEXERCISE - PHYSICAL CHEMITRY (SOLUTION & COLLIGATIVE PROPERTIES)
52 Videos
- STEREOISOMERISMBOOK - RESONANCE ENGLISHCHAPTER - STEREOISOMERISMEXERCISE - EXERCISE (PART III : PRACTICE TEST-2 (IIT-JEE (ADVANCED PATTERN))
23 Videos
Similar Questions
Knowledge Check
Assertion : The complex K3[Cr(C2O4)3] when present in aqueous solution , will give test for K+ , Cr3+ and oxalate ions .
Reason : The complex K3[Cr(C2O4)3] will dissociate completely in solution .AIf both Assertion and reason are true and reason is the correct explanation of assertionBIf both assertion and reason are true but reason is not the correct explanation of assertionCIf assertion is true but reason is falseDif both assertion and reason are false
A 0.001 molal solution of a complex represented as Pt(NH3)4Cl4 in water had freezing point depression of 0.0054∘C. Given Kf for H2O=1.86Km−1. Assuming 100% ionization of the complex, write the ionization nature and formula or complex.
View Solution17.4% (wt./vol.) K2(SO4) solution at 27∘C isotonic to 5.85% (wt./vol.) NaCl solution at 27∘C. IF NaCl is 100% ionised, what is % ionisation of K2SO4 in aq. solution?
View SolutionConsider following figure and answer the questions at the end of it. Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
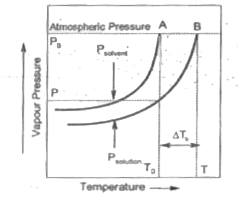
Variation of vapour pressure with temperature and showing elevation in boiling point.
A complex of iron and cyanide ions is 100% ionized at 1m (molal). If elevation in boiling point is 2.08∘(Kb=0.52∘mol−1kg) then the complex is:
View SolutionPtCl4.6H2Ocan exist as hydrated complex 1 molal aq.solution has depression in freezing point of 3.72∘CAssume 100% ionisation and Kf(H2O=1.86∘Cmol−1Kg) then complex is
View SolutionA hexa-coordinated complex of formula CoCl3.6H2O undergoes 100% ioisation is aqueous solutin to give van't Hoff factor equal to 3. Which of the following is/are correct regarding the given complex?
View SolutionAn aqueous solution of a solute AB has b.p. of 101.08∘C(AB is 100% ionised at boiling point of the solution ) and freezes at −1.80∘C. Hence, AB(Kb/Kf=0.3)
View SolutionA complex is represented as CoCl3.XNH3. Its 0.1 molal solution in aqueous solution shows ΔTf=0.558∘.(Kf for H2O is 1.86Kmolality−1) Assuming 100% ionisation of complex and co-ordination number of Co as six, calculate formula of complex.
View Solution
