If the range of the function y=x−1x+1 on the interval 0≤x≤4 be [a,b] then the value of 5b−2a=_________ .
To solve the problem, we need to find the range of the function y=x−1x+1 on the interval (0,4) and then compute 5b−2a where (a,b) is the range of the function.
1. Rewrite the Function:
We start with the function:
y=x−1x+1
We can manipulate this expression for easier analysis. By adding and subtracting 1 in the numerator, we can rewrite it as:
y=x+1−2x+1=x+1x+1−2x+1=1−2x+1
2. Determine the Interval for x:
The given interval for x is (0,4). Therefore, we need to evaluate y at the endpoints of this interval.
3. Calculate y at the Endpoints:
- For x=0:
y(0)=1−20+1=1−2=−1
- For x=4:
y(4)=1−24+1=1−25=1−0.4=0.6=35
4. Determine the Range:
Now we have the values of y at the endpoints:
- At x=0, y=−1
- At x=4, y=35
Since y is a continuous function and decreases as x increases (as seen from the derivative or the form of the function), the range of y on the interval (0,4) is:
Range=(−1,35)
Thus, a=−1 and b=35.
5. Calculate 5b−2a:
Now we substitute a and b into the expression 5b−2a:
5b−2a=5(35)−2(−1)=3+2=5
Final Answer:
The value of 5b−2a is:
5
We start with the function:
y=x−1x+1
We can manipulate this expression for easier analysis. By adding and subtracting 1 in the numerator, we can rewrite it as:
y=x+1−2x+1=x+1x+1−2x+1=1−2x+1
The given interval for x is (0,4). Therefore, we need to evaluate y at the endpoints of this interval.
- For x=0:
y(0)=1−20+1=1−2=−1
- For x=4:
y(4)=1−24+1=1−25=1−0.4=0.6=35
Now we have the values of y at the endpoints:
- At x=0, y=−1
- At x=4, y=35
Range=(−1,35)
Thus, a=−1 and b=35.
Now we substitute a and b into the expression 5b−2a:
5b−2a=5(35)−2(−1)=3+2=5
The value of 5b−2a is:
5
Topper's Solved these Questions
Similar Questions
The range of the function sin−1(x2+2x),x>0 is
View Solution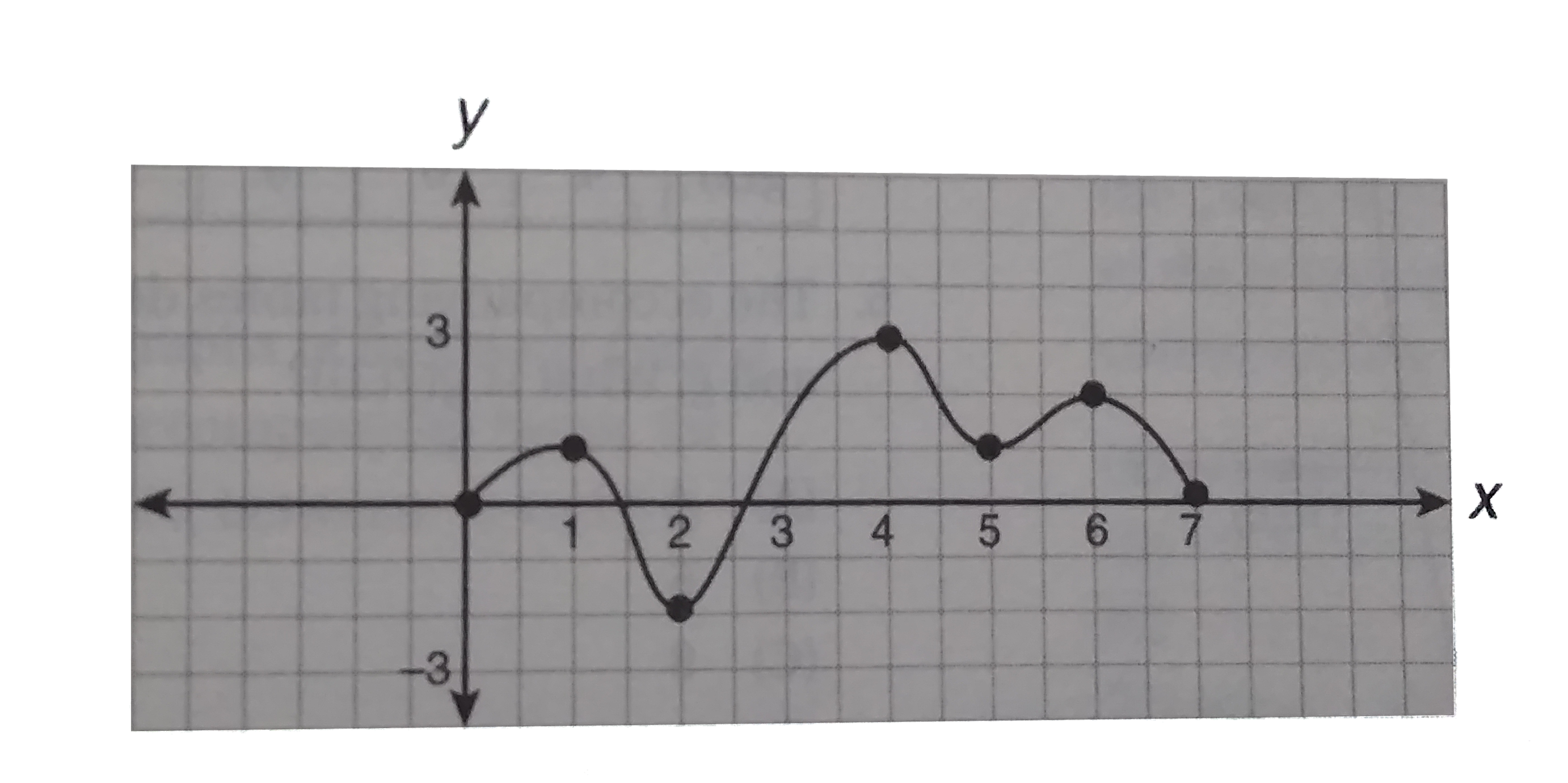
The graph above shows the function y=f(x) over the interval 0≤x≤7. What is the value of f(f(6))?
View SolutionIf the range of function f(x)=x+1k+x2 contains the interval
[-0,1] , then values of k can be equal to
View SolutionIf the range of the function f(x)=cot−1(x2x2+1) is (a, b), find the value of (ba+2).
View SolutionFind the range of the following functions.
y=x21+x2
View SolutionThe range of the function f(x)=x2ln(x) for x∈[1,e] is [a,b], where a+b is equal to
View SolutionThe function f(x)=2x2−1x4,x>0decreases in the interval
View SolutionIf the largest interval of x in which the function f(x)=x3−3x+1 is decreasing is (a, b), then the value of a+2b is equal to
View SolutionThe function y=ax+bx−1(x−4) has turning point at P(2,-1) Then find the values of a and b.
View SolutionDraw the graph of function. y=∣∣4−x2∣∣,−3≤x≤3.
View Solution
