A circular loop of rope of length L rotates with uniform angular velocity ω about an axis through its centre on a horizontal smooth platform. Velocity of pulse (with resppect to rope ) produce due to slight radiul displacement is given by ωL2(π) ,if both are in opposite direction. Then the velocity of the pulse w.r.t. to groun will be:
ωL
ωL2π
ωLπ
0
The correct Answer is:D
To solve the problem, we need to find the velocity of the pulse with respect to the ground when a circular loop of rope of length L rotates with uniform angular velocity ω and the pulse moves in the opposite direction to the rotation of the rope.
1. Understanding the Setup:
- We have a circular loop of rope of length L rotating about its center with angular velocity ω.
- The velocity of the pulse with respect to the rope is given as ωL2π.
2. Identifying the Directions:
- Let's assume the pulse moves in one direction (say clockwise) and the rope rotates in the opposite direction (counterclockwise).
3. Velocity of the Rope:
- The linear velocity v of a point on the rope at radius r is given by:
v=r⋅ω
- The radius r of the circular loop can be calculated from its circumference:
2πr=L⟹r=L2π
- Therefore, the velocity of the rope at this radius is:
vrope=r⋅ω=(L2π)⋅ω=ωL2π
4. Velocity of the Pulse with Respect to the Ground:
- The velocity of the pulse with respect to the ground vpulse, ground can be expressed as:
vpulse, ground=vpulse, rope+vrope
- Here, vpulse, rope=ωL2π (in the clockwise direction) and vrope=ωL2π (in the counterclockwise direction). Since they are in opposite directions, we subtract the rope's velocity from the pulse's velocity:
vpulse, ground=ωL2π−ωL2π=0
5. Conclusion:
- The velocity of the pulse with respect to the ground is 0.
Final Answer:
The velocity of the pulse with respect to the ground is 0.
---
- We have a circular loop of rope of length L rotating about its center with angular velocity ω.
- The velocity of the pulse with respect to the rope is given as ωL2π.
- Let's assume the pulse moves in one direction (say clockwise) and the rope rotates in the opposite direction (counterclockwise).
- The linear velocity v of a point on the rope at radius r is given by:
v=r⋅ω
- The radius r of the circular loop can be calculated from its circumference:
2πr=L⟹r=L2π
- Therefore, the velocity of the rope at this radius is:
vrope=r⋅ω=(L2π)⋅ω=ωL2π
- The velocity of the pulse with respect to the ground vpulse, ground can be expressed as:
vpulse, ground=vpulse, rope+vrope
- Here, vpulse, rope=ωL2π (in the clockwise direction) and vrope=ωL2π (in the counterclockwise direction). Since they are in opposite directions, we subtract the rope's velocity from the pulse's velocity:
vpulse, ground=ωL2π−ωL2π=0
- The velocity of the pulse with respect to the ground is 0.
The velocity of the pulse with respect to the ground is 0.
Topper's Solved these Questions
- WAVE ON STRING Book:RESONANCE ENGLISHChapter:WAVE ON STRING Exercise:Exercise- 2 PART II
Explore 19 Videos
- WAVE ON STRING Book:RESONANCE ENGLISHChapter:WAVE ON STRING Exercise:Exercise- 2 PART III
Explore 15 Videos
- WAVE ON STRING Book:RESONANCE ENGLISHChapter:WAVE ON STRING Exercise:Exercise- 1 PART II
Explore 31 Videos
- TRAVELLING WAVESBook:RESONANCE ENGLISHChapter:TRAVELLING WAVESExercise:Exercise- 3 PART I
Explore 19 Videos
- WAVE OPTICSBook:RESONANCE ENGLISHChapter:WAVE OPTICSExercise:Advanced Level Problems
Explore 8 Videos
Similar Questions
A circular loop of rope of length L rotates with uniform angular velocity ω about an axis through its centre on a horizontal smooth platform. Velocity of pulse (with resppect to rope ) produce due to slight radiul displacement is given by ωL2π , if the motion of the pulse and rotation of the loop, both are in same direction then the velocity of the pulse w.r.t. to ground will be:
View SolutionIn the above question,if both are in opposite direction. Then the velocity of the pulse w.r.t. to groun will be:
View Solution
Knowledge Check
A rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the lrod is
ABl2ω2BzeroC(Bl2ω8)D2Bl2ω
A circular disc is rotating about its own axis at an angular velocity ω. If P is exact midpoint of dise between axis and rim of disc then angular velocity of Pis
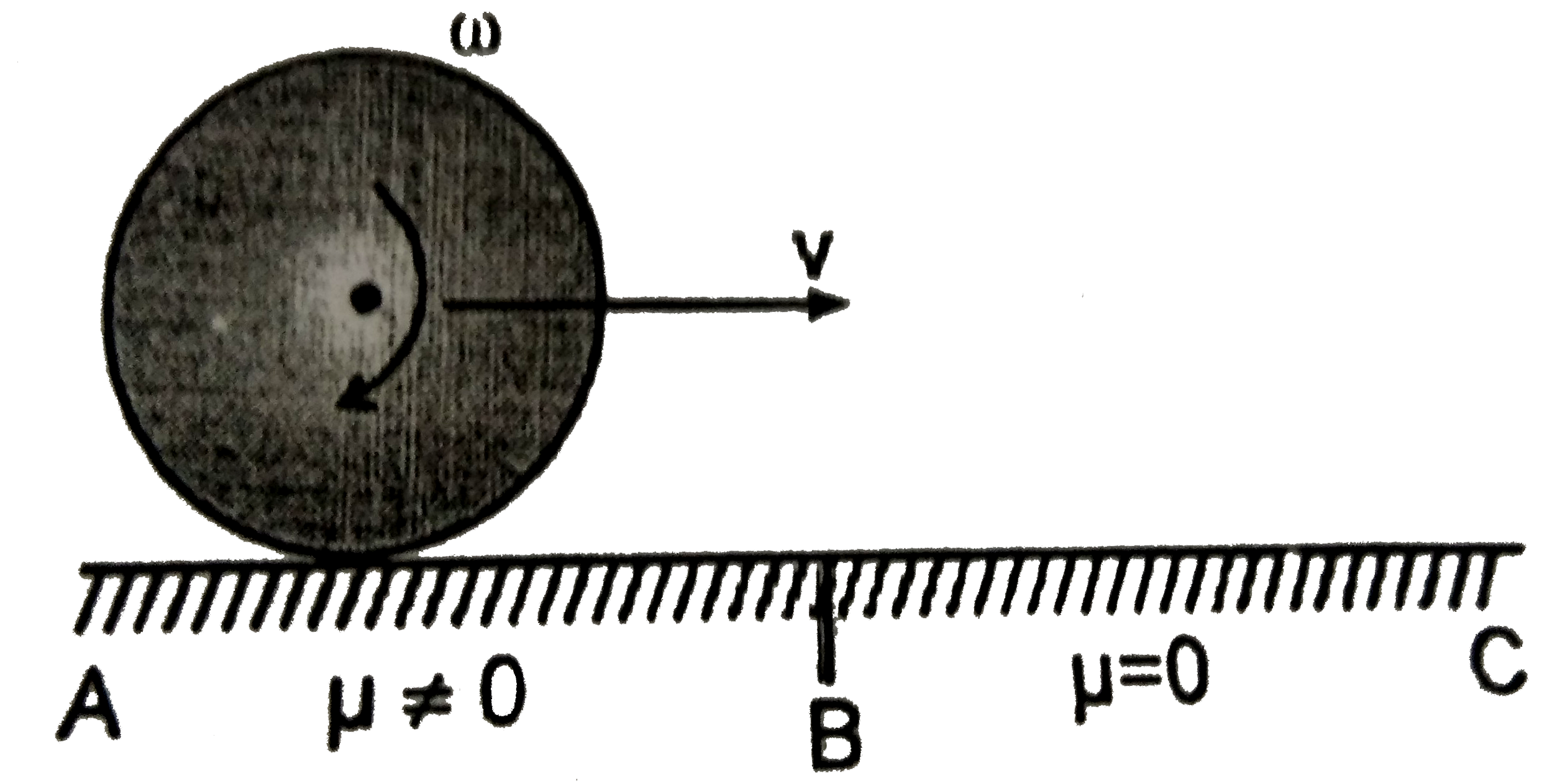
View SolutionAs shown in the figure, a disc of mass m is rolling without slipping a angular velocity ω. When it crosses point B disc will be in :
View SolutionA rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the lrod is
View SolutionA uniform rod of length l is spinning with an angular velocity ω=2vl while its centre of mass moves with a velocity v. Find the velocity of the end of the rod.
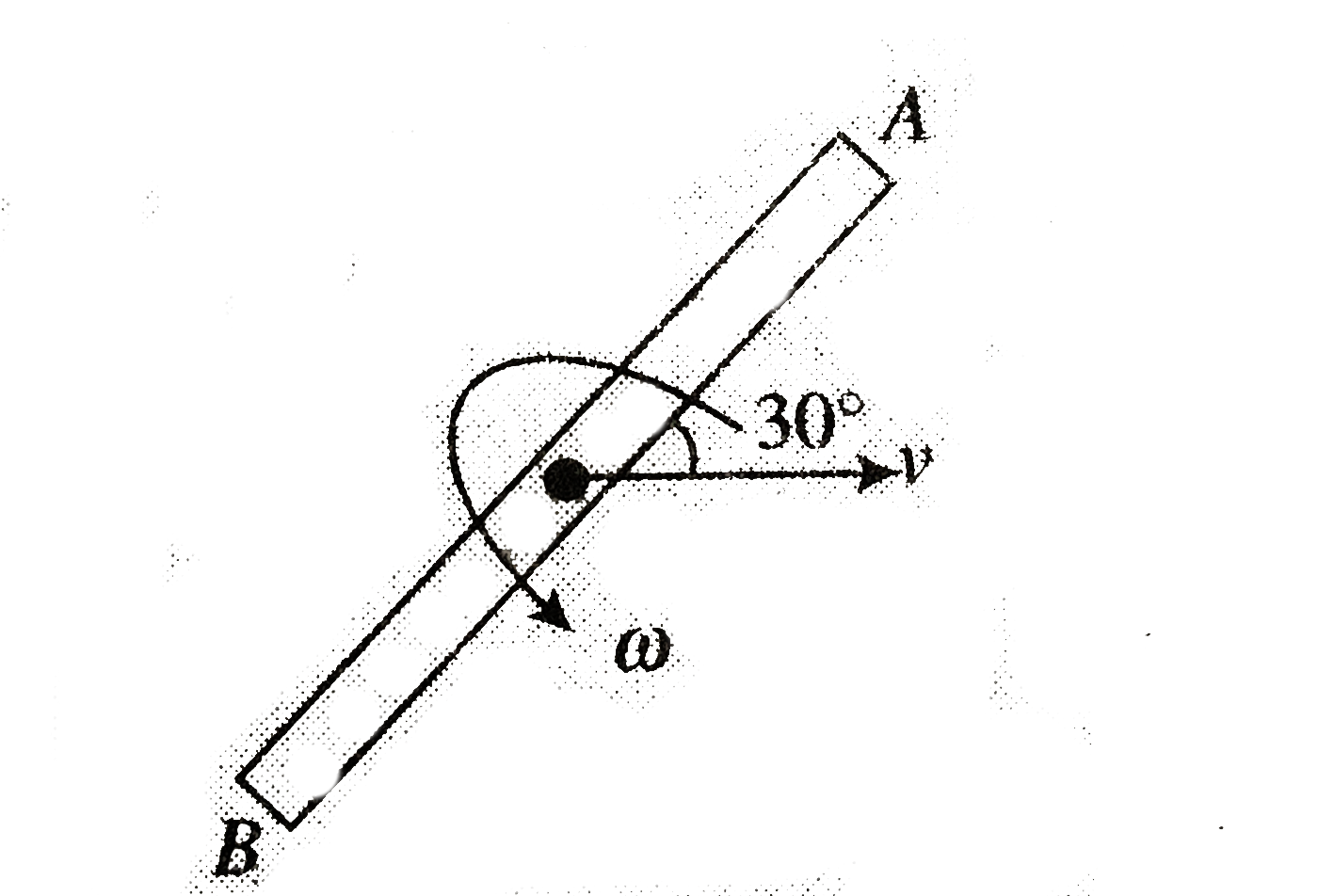
View SolutionA square loop of edge a having n turns Is rotated with a uniform angular velocity ω about one of its diagonals which is kept fixed in a horizontal position . A uniform magnetic field B exists in the vertical direction. Find the emf induced in the coil.

View SolutionA horizontal disk is rotating with angular velocity ω about a vertical axis passing through its centre. A ball is placed at the centre of groove and pushed slightly. The velocity of the ball when it comes out of the groove -

View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View Solution
