In the above question,if both are in opposite direction. Then the velocity of the pulse w.r.t. to groun will be:
0
The correct Answer is:d
To solve the problem of finding the velocity of the pulse with respect to the ground when two waves are moving in opposite directions, we can follow these steps:
1. Understanding the Problem: We have two waves (let's call them Wave A and Wave B) moving in opposite directions. We need to find the velocity of the pulse created by these waves with respect to the ground.
2. Define the Velocities: Let the velocity of Wave A be vA and the velocity of Wave B be vB. Since they are moving in opposite directions, we can denote their velocities as positive and negative respectively. For example, if Wave A moves to the right, we can write:
vA=+v
vB=−v
3. Relative Velocity Concept: The velocity of the pulse with respect to the ground is the vector sum of the velocities of the two waves. Since they are moving in opposite directions, we can express the velocity of the pulse vpulse as:
vpulse=vA+vB
4. Substituting the Values: Substituting the values of vA and vB into the equation:
vpulse=v+(−v)=0
5. Conclusion: The velocity of the pulse with respect to the ground is:
vpulse=0
This means that the pulse does not move with respect to the ground when both waves are moving in opposite directions at the same speed.
Final Answer:
The velocity of the pulse with respect to the ground is 0.
vA=+v
vB=−v
vpulse=vA+vB
vpulse=v+(−v)=0
vpulse=0
This means that the pulse does not move with respect to the ground when both waves are moving in opposite directions at the same speed.
The velocity of the pulse with respect to the ground is 0.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer1videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Multiple Correct25videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Subjective16videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A circular loop of rope of length L rotates with uniform angular velocity ω about an axis through its centre on a horizontal smooth platform. Velocity of pulse (with resppect to rope ) produce due to slight radiul displacement is given by ωL2(π) ,if both are in opposite direction. Then the velocity of the pulse w.r.t. to groun will be:
View SolutionA circular loop of rope of length L rotates with uniform angular velocity ω about an axis through its centre on a horizontal smooth platform. Velocity of pulse (with resppect to rope ) produce due to slight radiul displacement is given by ωL2π , if the motion of the pulse and rotation of the loop, both are in same direction then the velocity of the pulse w.r.t. to ground will be:
View SolutionIn the above question, the angular velocity will:
View SolutionFig. 144.9 shops to wave pulses travelling along a string in the opposite directions. The velocity of the wave is 3 m/s and the pulses are 9cm apart. Find what has hap-pened to the energy at t=1.5s.
View SolutionIn the above question if the coil rotates with a constant angular velocity ω, the EMF induced in it
View Solutionf A and B persons are moving with VAandVB. velocities in opposite directions. Magnitude of relative velocity of B w.r.t. A is x and magni-tude of relative velocity of A w.r.t B is y. Then
View SolutionTwo cars are moving is one a same line dimension in opposite direction with the same speed v. The relative velocity of two cars w.r.t. each other is
View SolutionIn the above question what will be the displacement of particle in x-direction when its velocity makes 60∘ angle with initial velocity?
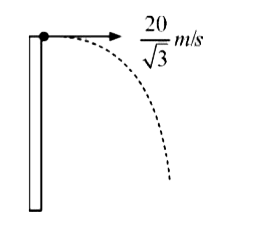 View Solution
View SolutionWe know that when a boat travels in water, its net velocity w.r.t. ground is the vector sum of two velocities. First is the velocity of boat itself in river and other is the velocity of water w.r.t. ground. Mathematically:
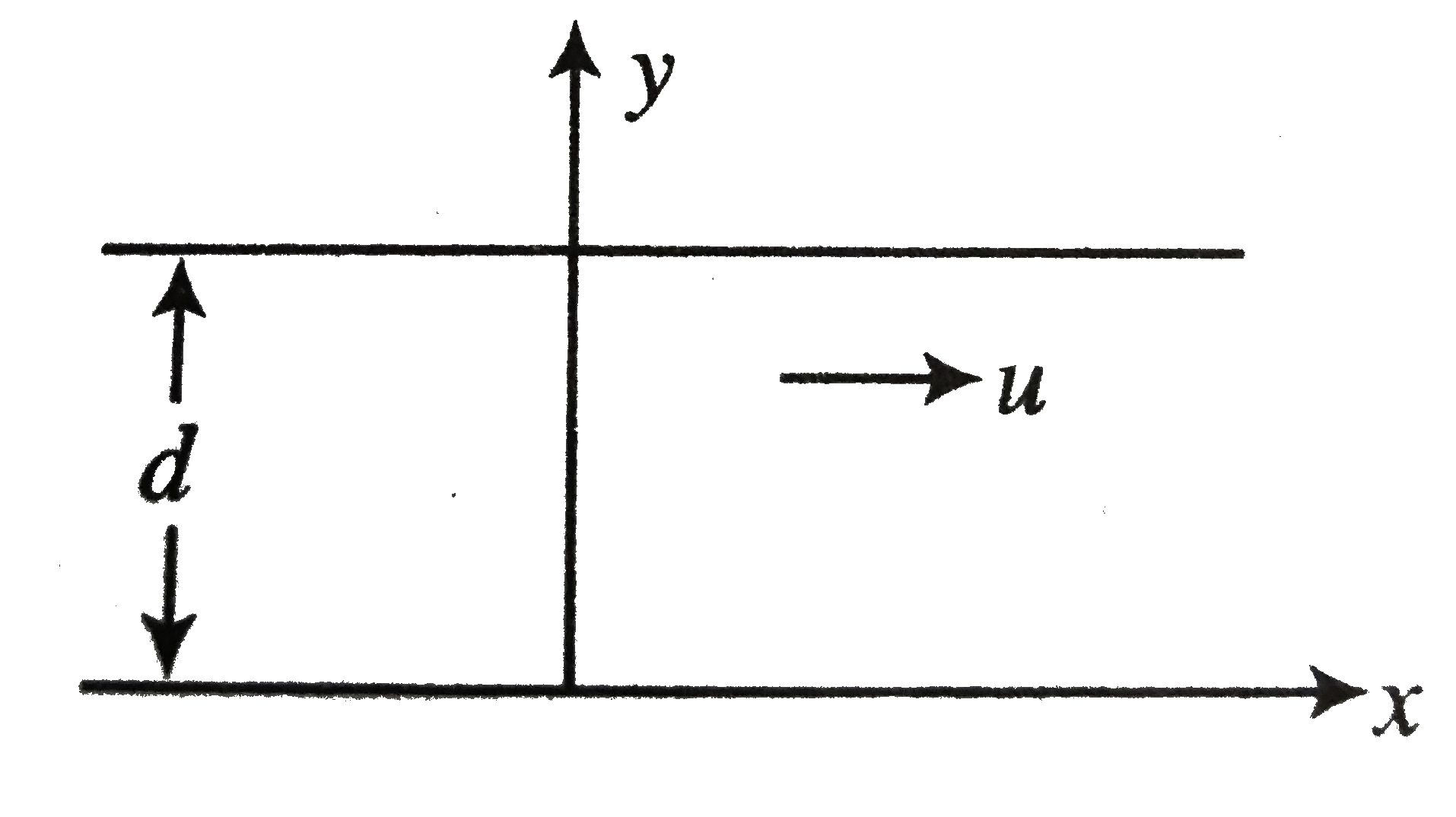
→vboat=→vboat,water+→vwater.
Now given that velocity of water w.r.t. ground in a river is u. Width of the river is d.
A boat starting from rest aims perpendicular to the river with an acceleration of a = 5t, where t is time. The boat starts from point (1,0) of the coordinate system as shown in figure. Assume SI units.View SolutionWe know that when a boat travels in water, its net velocity w.r.t. ground is the vector sum of two velocities. First is the velocity of boat itself in river and other is the velocity of water w.r.t. ground. Mathematically:
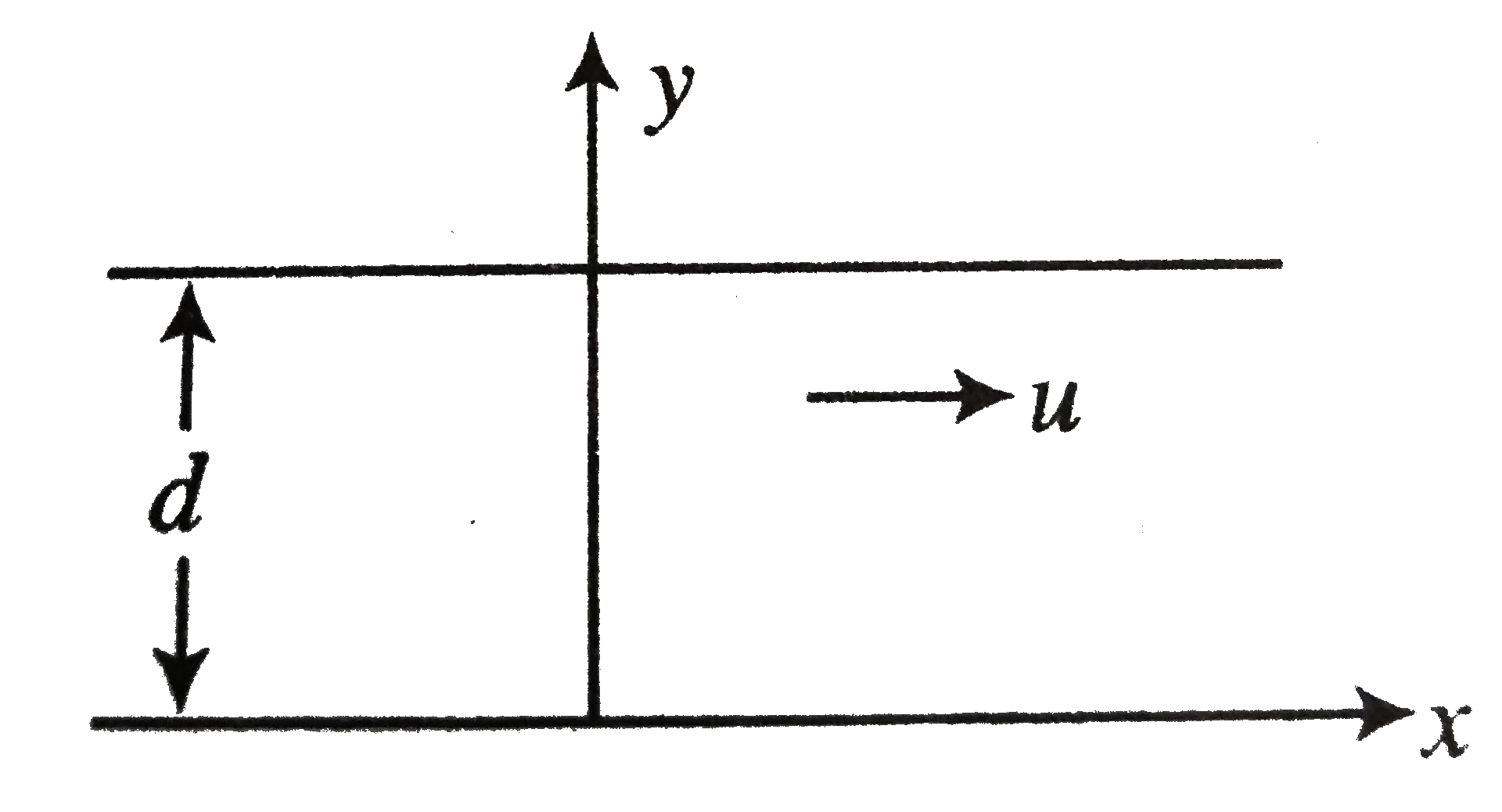
→vboat=→vboat,water+→vwater.
Now given that velocity of water w.r.t. ground in a river is u. Width of the river is d.
A boat starting from rest aims perpendicular to the river with an acceleration of a = 5t, where t is time. The boat starts from point (1,0) of the coordinate system as shown in figure. Assume SI units. Find time taken by him to across the river.View Solution

