STATEMENT-1 The formula connecting u,v and f for a spherical mirrors whose sizes are very small compared to their radii of curvature.
STATEMENT-2 Laws of reflection are strictly valid for plane surfaces, but not for large spherical surfaces.
Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement -1
Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement -1
Statement-1 is True, Statement-2 is False
Statement-1 is False, Statement-2 is True.
The correct Answer is:C
To solve the given question, we will analyze both statements separately and determine their validity based on the principles of geometrical optics.
Step 1: Analyze Statement 1
- Statement: The formula connecting u,v, and f for a spherical mirror whose sizes are very small compared to their radii of curvature.
- Explanation: The formula for spherical mirrors is given by:
1f=1v+1u
where f is the focal length, v is the image distance, and u is the object distance. This formula is derived under the assumption that the size of the mirror is small compared to its radius of curvature. This approximation allows us to treat the rays as if they are parallel and simplifies the geometry involved in deriving the mirror formula.
- Conclusion: Statement 1 is True.
Step 2: Analyze Statement 2
- Statement: Laws of reflection are strictly valid for plane surfaces, but not for large spherical surfaces.
- Explanation: The law of reflection states that the angle of incidence is equal to the angle of reflection, and this principle applies to all reflective surfaces, including spherical mirrors, regardless of their size. While the approximation used in deriving the mirror formula may break down for very large spherical mirrors, the law of reflection itself remains valid.
- Conclusion: Statement 2 is False.
Final Conclusion:
- Statement 1 is True.
- Statement 2 is False.
Thus, the correct answer is that Statement 1 is true and Statement 2 is false.
- Statement: The formula connecting u,v, and f for a spherical mirror whose sizes are very small compared to their radii of curvature.
- Explanation: The formula for spherical mirrors is given by:
1f=1v+1u
where f is the focal length, v is the image distance, and u is the object distance. This formula is derived under the assumption that the size of the mirror is small compared to its radius of curvature. This approximation allows us to treat the rays as if they are parallel and simplifies the geometry involved in deriving the mirror formula.
- Conclusion: Statement 1 is True.
- Statement: Laws of reflection are strictly valid for plane surfaces, but not for large spherical surfaces.
- Explanation: The law of reflection states that the angle of incidence is equal to the angle of reflection, and this principle applies to all reflective surfaces, including spherical mirrors, regardless of their size. While the approximation used in deriving the mirror formula may break down for very large spherical mirrors, the law of reflection itself remains valid.
- Conclusion: Statement 2 is False.
- Statement 1 is True.
- Statement 2 is False.
Topper's Solved these Questions
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Advance level Problems
35 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-2
62 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS
16 Videos
Similar Questions
In each of the questions, assertion(A) is given by corresponding statement of reason (R) of the statemens. Mark the correct answer.
Q. Statement I: The formula connecting u,v and f for a spherical mirror is valid only for mirrors whose sizes are very small compared to their radii of curvature.
Statement II: Laws of reflection are strictly valid for plane surfaces, but not for large spherical surfaces.
View SolutionAssertion The formula connecting u, v and f for a spherical mirror is valid only for mirrors sizes which are very small compared to their radii of curvature.
Reason Laws of reflection are stricity valid for plane surface, but not for large spherical.
View SolutionA thin copper rod of uniform cross section A square metres and of length L metres has a spherical metal sphere of radius r metre at tis one end symmetrically attached to the copper rod. The thermal conductivity of copper is K and the emissivity of the spherical surface of the sphere is ε.The free end of the copper rod is maintained at the temperature T kelving by supplying thermal energy from a P watt source. Steady state conditions are allowed ot be established while the rod is properly insulated aginst heat loss from its lateral surface. Surroundings are at 0∘C Stefan's constant=σW/m2K4.
If the metal sphere attached at the end of the copper rod is made of brass, whose thermal conductivity is Kb<K, then which of the following statements is true?
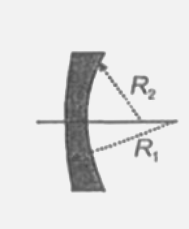
View SolutionIf R1andR2 are the radii of curvature of the spherical surfaces of a thin lens and R1>R2, then this lens can
View SolutionA thin bi-convex lens made up of glass of refractive index 3/2 is placed is front of a plane mirror. The space between the lens and the mirror is filled with water of refractive index 4/3 . The radii of curvatures of the lens are R1=15cm and R2=25cm . A point object is placed at distance x from the surface whose curvature is R1. The distance x is greater than the separation between the lens and the mirror. Find the value of x so that image must coincide with the object.
View SolutionTrace the rays of light showing the formation of an image due to a point object placed on the axis of a spherical surface separating the two media of refractive indices n1andn2. Establish the relation between the distance of the object, the image and the radius of curvature from the central point of the spherical surface.
Hence derive the expression of the lens maker's formula
View SolutionStatement-1:All colloidal dispersions give very low osmotic pressure and show very small freezing point depression or boiling point elevation.
Statement-2:Tyndall effect is due to scattering of light from the surface of colloidal particles.
View SolutionA small conducting spherical shell with inner radius a and outer radius b is concentric with a larger conducting sphereical shell with inner radius c and outer radius d. The inner shell has total charge +2q and the outer shell has charge +4q.
a. Make a plot of the magnitude of the electric field versus r.
b. Calculate the electric field (magnitude and direction in terms of q) and the distance r from the common centre of the two shell for (i)r<a,(ii)a<r<b,(iii)bltrltc,(iv)cltrltd,(v)rgtd.Showuourrest–∈agraphwithradialcompo≠ntofvecEasfunctionofr.
c. What is the total charge on the
i. inner surface of the small shell,
ii. outer surface of the small shell,
iii. inner surface of the large shell,
iv. outer surface of the large shell?
View Solution(a) define electrie flux. Write its, S.I unit.
" Gauses's law in electrostatis is true for any closed surface, no matter what its shape or size is.
Justify this statement with the help of a suitable example.
(b) Use Gauss's law to prove that the electric field inside a uniformly charged spherical shell is zero.
View SolutionWhen two concentric shells are connected by a thin conducting wire, whole of the charge of inner shell transfers to the outer shell and potential difference between them becomes zero. Surface charge densities of two thin concentric spherical shells are σ and −σ respectively. Their radii are R and 2R. Now they are connected by a thin wire.
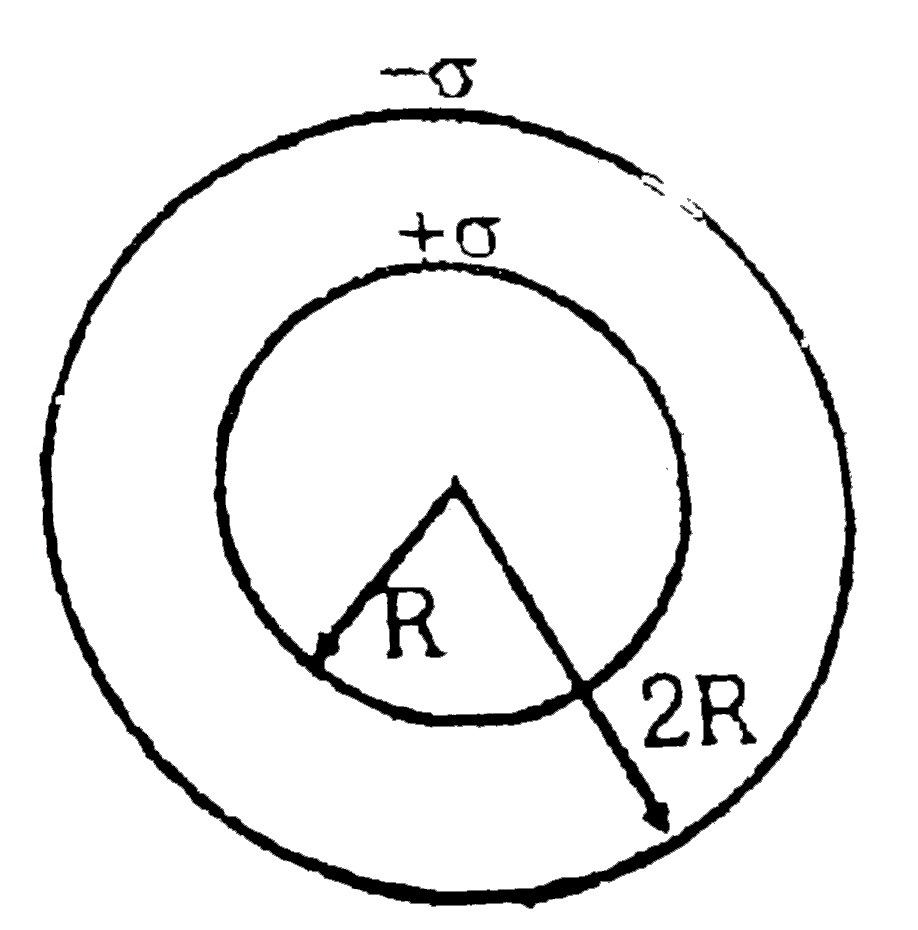
Suppose electric field at a distance r(>2R) was E1 before connecting the two shells and E2 after connecting the two shells, then ∣∣∣E2E1∣∣∣ is :-
View Solution
