Two short magnet and of magnetic dipole mement and respectively are placed as shown.The axis of and the equatorial line of are the same.Find the magnetic force on one magnet due to other.
The correct Answer is: , upwards on
down wards on
To find the magnetic force on one magnet due to the other, we can follow these steps:
Step 1: Understand the Configuration
We have two short magnets, A and B, with magnetic dipole moments M1 and M2 respectively. The axis of magnet A coincides with the equatorial line of magnet B.
Step 2: Magnetic Field at the Equatorial Line
The magnetic field B at the equatorial line of a magnetic dipole can be expressed as:
B=μ04π⋅2Mr3
where M is the magnetic moment of the magnet, r is the distance from the magnet, and μ0 is the permeability of free space.
Step 3: Magnetic Field Due to Magnet B at the Location of Magnet A
Since we are interested in the magnetic field at the location of magnet A due to magnet B, we can write:
BB=μ04π⋅2M2r3
where M2 is the magnetic moment of magnet B and r is the distance between the two magnets.
Step 4: Force on Magnet A Due to Magnet B
The force F on a magnetic dipole in a magnetic field is given by:
F=M⋅dBdr
For magnet A, we need to calculate the derivative of the magnetic field BB with respect to r:
dBBdr=ddr(μ04π⋅2M2r3)=−3μ04π⋅2M2r4
Step 5: Substitute into the Force Equation
Now we can substitute this derivative back into the force equation for magnet A:
FA=M1⋅(−3μ04π⋅2M2r4)
This simplifies to:
FA=−3μ04π⋅2M1M2r4
Step 6: Conclusion
The magnetic force on magnet A due to magnet B is:
FA=−3μ04π⋅2M1M2r4
This indicates that the force is attractive since it is negative.
---
We have two short magnets, A and B, with magnetic dipole moments M1 and M2 respectively. The axis of magnet A coincides with the equatorial line of magnet B.
The magnetic field B at the equatorial line of a magnetic dipole can be expressed as:
B=μ04π⋅2Mr3
where M is the magnetic moment of the magnet, r is the distance from the magnet, and μ0 is the permeability of free space.
Since we are interested in the magnetic field at the location of magnet A due to magnet B, we can write:
BB=μ04π⋅2M2r3
where M2 is the magnetic moment of magnet B and r is the distance between the two magnets.
The force F on a magnetic dipole in a magnetic field is given by:
F=M⋅dBdr
For magnet A, we need to calculate the derivative of the magnetic field BB with respect to r:
dBBdr=ddr(μ04π⋅2M2r3)=−3μ04π⋅2M2r4
Now we can substitute this derivative back into the force equation for magnet A:
FA=M1⋅(−3μ04π⋅2M2r4)
This simplifies to:
FA=−3μ04π⋅2M1M2r4
The magnetic force on magnet A due to magnet B is:
FA=−3μ04π⋅2M1M2r4
This indicates that the force is attractive since it is negative.
Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-1 PART-1
83 Videos
- ELECTRODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-1 PART-2
54 Videos
- ELECTRODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
Similar Questions
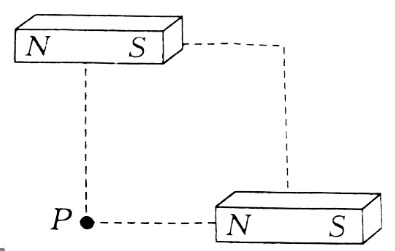
Two short magnets of magnetic moment 1000Am2 are placed as shown at the corners of a square of side 10cm. The net magnetic induction at P is
View SolutionTwo short magnets of equal dipole moments M are fastened perpendicularly at their centres (figure). The magnitude of the magnetic field at a distance d from the centre on the bisector of the right angle is
(##HCV_VOL2_C36_E01_006_Q01##)
View Solution
Knowledge Check
Two identical magnetic dipoles of magnetic moments 1⋅0Am2 each are placed at a separation of 2m with their axes perpendicular to each other. What is the resultant magnetic field at a point midway between the dipoles?
A√5×10−7TB5×10−7TC√2×10−7TD10−7T
A bar magnetic dipole moment M is cut into two equal pieces as shown, magnetic moment of each piece is
View SolutionTwo lines of force due to a bar magnet
View SolutionOrientation of two identical small bar magnet of magnetic moment 'M' is as shown in figure. The magnitude of magnetic field at the point which lies on equatorial line of one magnet and axial line of another magnet is (r >> size of magnet)
View SolutionFigure shows two identical magnetic dipoles aandb of magnetic moments M each, placed at a separation d, with their axes perpendicular to each other. Find the magnetic field at the point P midway between the dipoles.
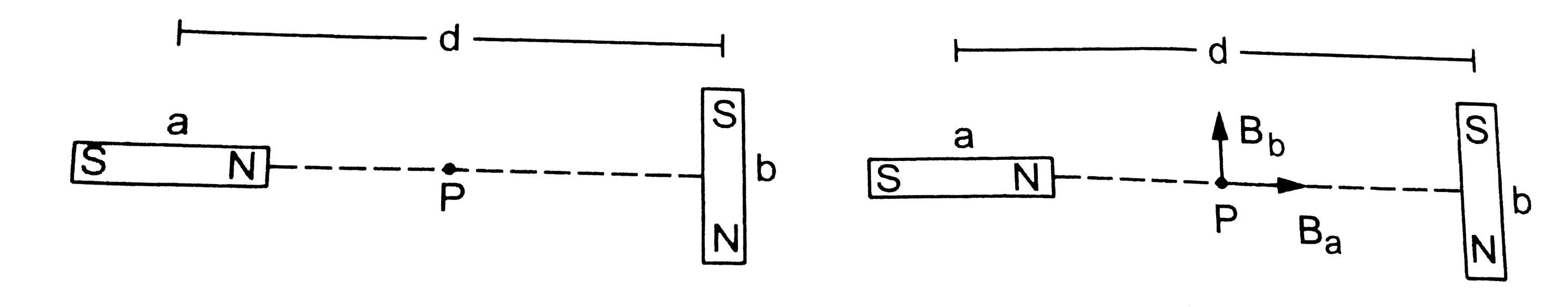
View SolutionTwo identical magnetic dipoles of magnetic moments 1⋅0Am2 each are placed at a separation of 2m with their axes perpendicular to each other. What is the resultant magnetic field at a point midway between the dipoles?
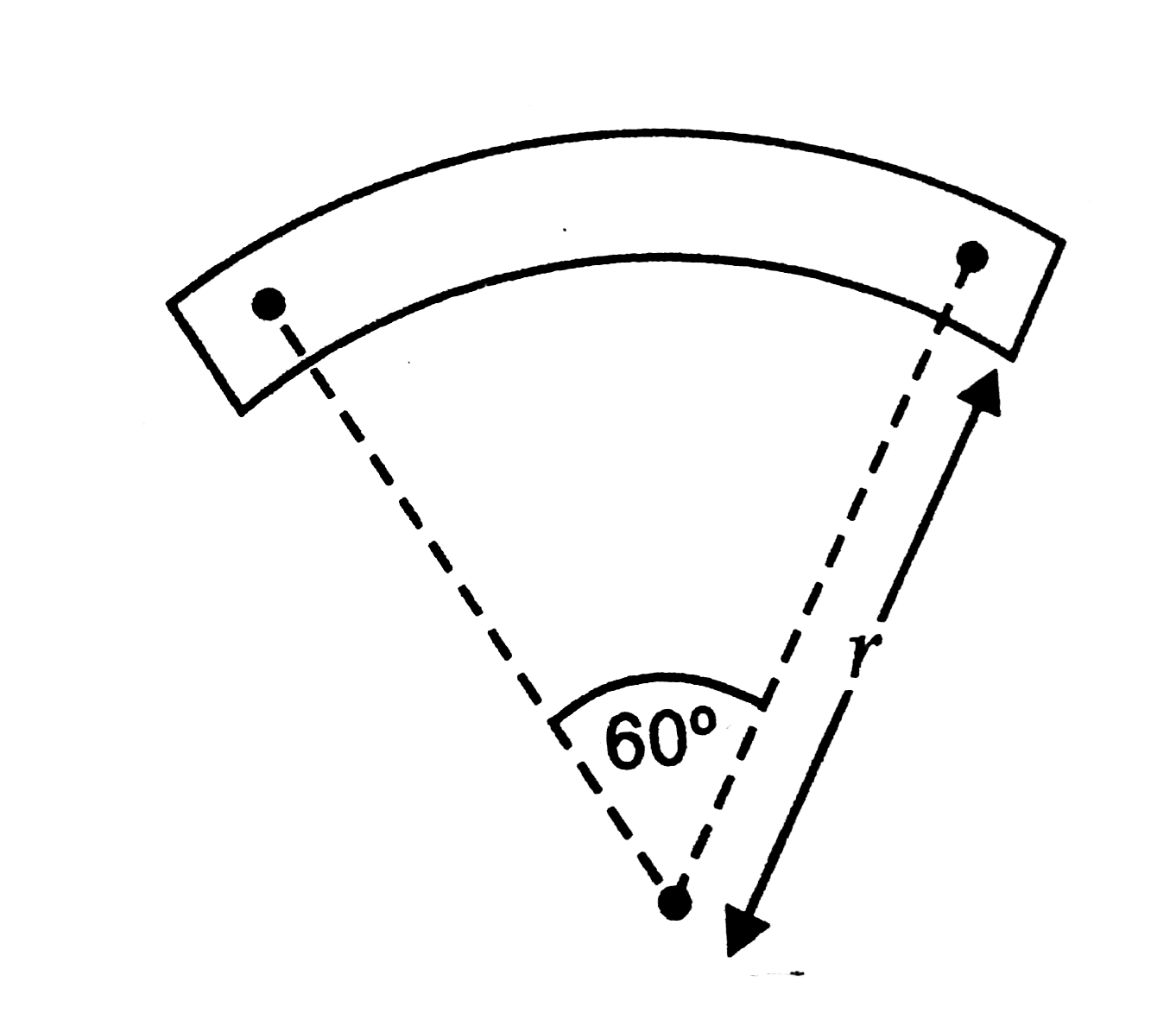
View SolutionA bar magnet of lenth l and magnetic dipole moment 'M' is bent in the form of an arc as shown in figure. The new magnetic dipole moment will be
View SolutionTwo identical short bar magnets, each having magnetic moment M, are placed a distance of 2d apart with axes perpendicular to each other in a horizontal plane. The magnetic induction at a point midway between them is
View Solution
