In the figure shown a conducting rod of length l, resistnace R and mass m is moved with a constant velocity v.The magnetic field B varies with time t as B=5t, where t is time in second.At t=0 the area of the loop containing capacitor and the rod is zero and the capacitor is uncharged.The rod started moving at t=0 on the fixed smooth conducting rails which have negligible resistance.Find (i)The current in the circuit as a function of time t.
(ii)If the above system is kept in vertical plane such that the rod can move vertically downward due of gravity and other parts are kept fixed and B=constant =B0.then find the maximum current in the circuit.
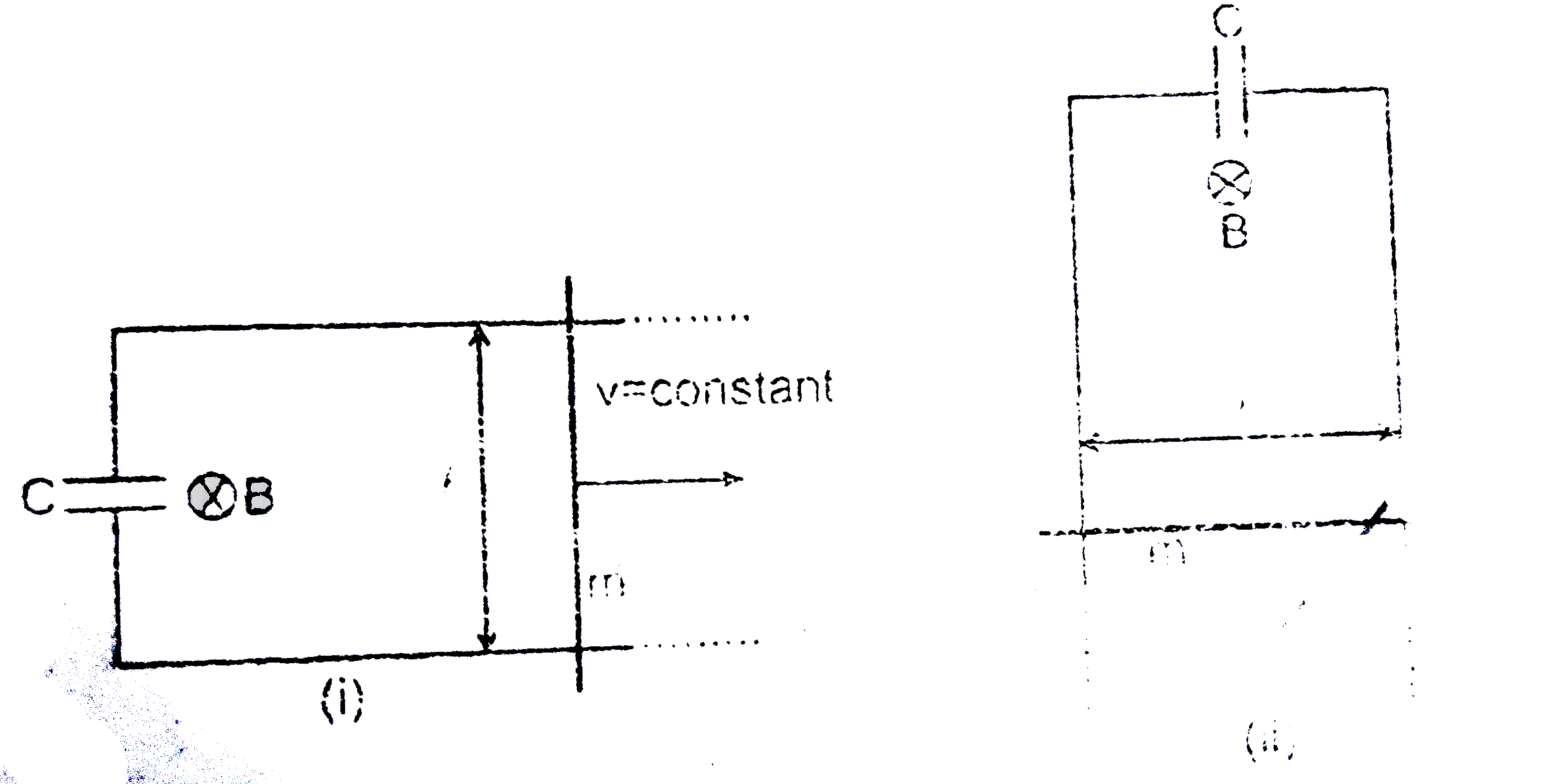
The correct Answer is:A, B, C
(i)ϕ=flux at time t
=BA=(5t)(lvt)=5lvt2
∴ε=∣∣∣dϕdt∣∣∣=10lvt
qc=ε−iR⇒qc=10lvt−iR
Differentiating both sides w.r.t t
10lv−didtR=ic
i=10lVc(1−e−t/cR)
(ii)q/c=ε−iR=BVl−IR,
for maximum value of i,di/dt=0
i/c=Bal−di/dt×R
l/c=B(mg−Bilm)l−0
∴i=imax=mgBlcm+B2l2c

Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-3 PART 3
16 Videos
- ELECTRODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - HLP
40 Videos
Similar Questions
Knowledge Check
A conducting metal circular-wire-loop of radius r is placed perpendicular to a magnetic field which varies with time as B=B0e−t/τ, where B0andτ are constants, at time = 0. If the resistance of the loop is R then the heat generated in the loop after a long time (t→∞) is :
Aπ2r4B402τRBπ2r4B202τRCπ2r4B20RτDπ2r4B20τR
A conducting rod of length l is moving in a transverse magnetic field of strength B with veocity v. The resistance of the rod is R. The current in the rod is
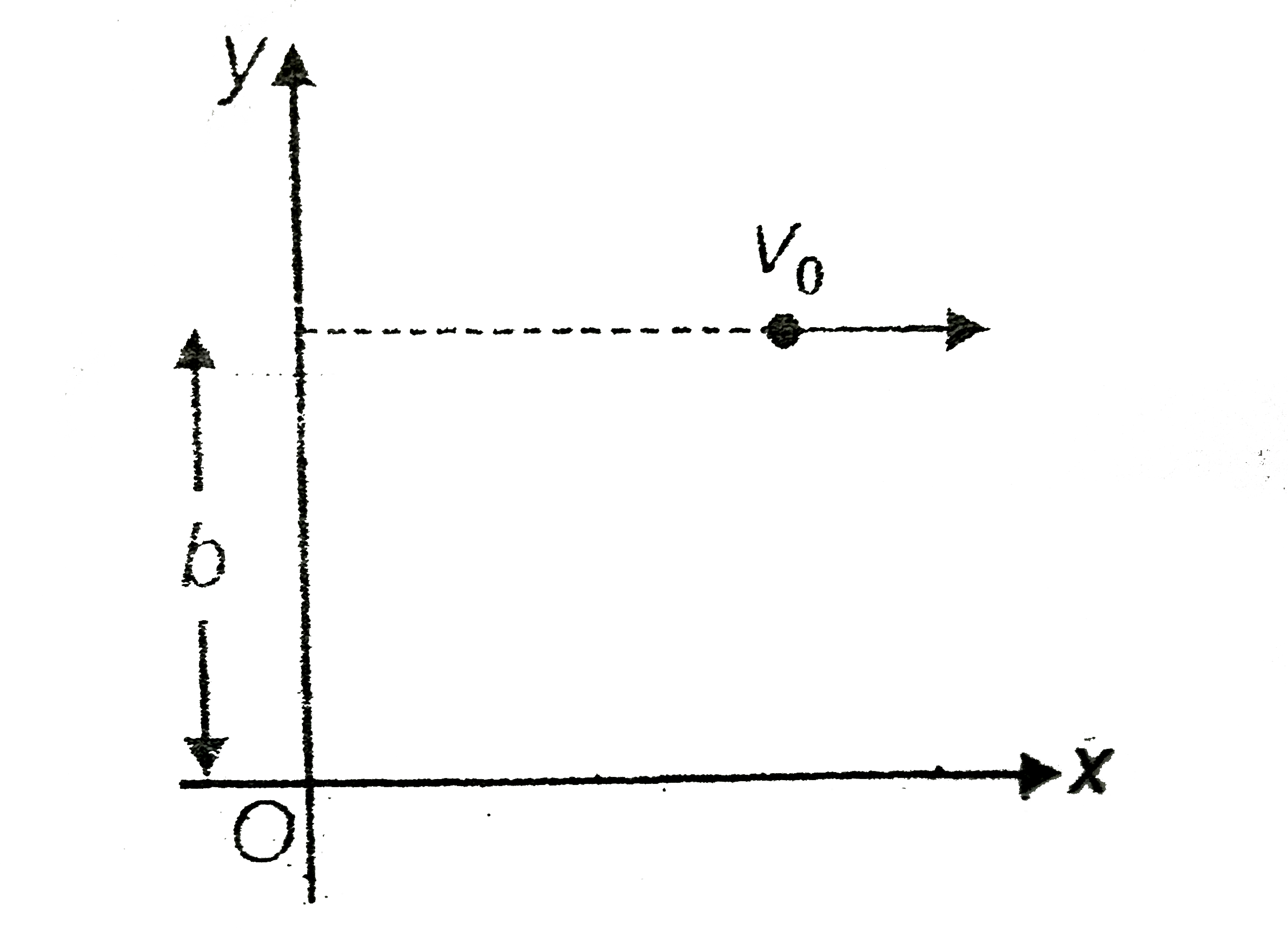
View SolutionA particle of mass m is moving with constant velocity v0 along the line y=b. At time t=0 it was at the point (0,b). At time t=bv0, the
View SolutionThe velocity v of a body moving along a straight line varies with time t as v=2t2e−t, where v is in m/s and t is in second. The acceleration of body is zero at t =
View SolutionA conducting circular loop of radius a and resistance R is kept on a horizontal plane. A vertical time varying magnetic field B=2t is switched on at time t=0. Then
View SolutionA conducting rod of resistance r moves uniformly with a constant speed v in a uniform magnetic field. If the rod keeps moving uniformly, then the amount of force required is
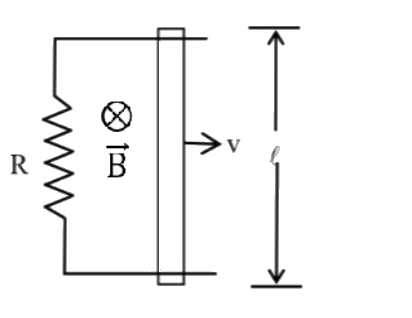
View SolutionA small conducting rod of length l, moves with a uniform velocity v in a uniform magnetic field B as shown in fig __

A. Then the end X of the rod become positively charged
B. The end Y of the rod become positively charged
C. The entire rod is unevenly charged
D. The rod become hot due to joule heating
View SolutionA conducting metal circular-wire-loop of radius r is placed perpendicular to a magnetic field which varies with time as B=B0e−t/τ, where B0andτ are constants, at time = 0. If the resistance of the loop is R then the heat generated in the loop after a long time (t→∞) is :
View Solution
