The wavelength of a moving body of mass 0.1mg is 3.31×10−29m. The kinetic energy of the body in J would be :
The correct Answer is:A
To find the kinetic energy of a moving body given its wavelength, we can follow these steps:
Step 1: Convert the mass to kilograms
The mass of the body is given as 0.1 mg. We need to convert this to kilograms:
Mass(m)=0.1mg=0.1×10−3g=0.1×10−6kg=10−7kg
Step 2: Use the de Broglie wavelength formula
The de Broglie wavelength (λ) is related to the momentum (p) of the body by the formula:
λ=hp
where h is Planck's constant (6.626×10−34Js). Rearranging this gives us:
p=hλ
Step 3: Substitute the values to find momentum
Now we can substitute the values of h and λ:
p=6.626×10−343.31×10−29
Calculating this gives:
p=2.00×10−5kg m/s
Step 4: Calculate the kinetic energy
The kinetic energy (KE) is given by the formula:
KE=p22m
Substituting the values of p and m:
KE=(2.00×10−5)22×(10−7)
Calculating p2:
(2.00×10−5)2=4.00×10−10
Now substituting this into the kinetic energy formula:
KE=4.00×10−102×10−7=4.00×10−102.00×10−7=2.00×10−3J
Final Answer
The kinetic energy of the body is:
KE=2.00×10−3J
---
The mass of the body is given as 0.1 mg. We need to convert this to kilograms:
Mass(m)=0.1mg=0.1×10−3g=0.1×10−6kg=10−7kg
The de Broglie wavelength (λ) is related to the momentum (p) of the body by the formula:
λ=hp
where h is Planck's constant (6.626×10−34Js). Rearranging this gives us:
p=hλ
Now we can substitute the values of h and λ:
p=6.626×10−343.31×10−29
Calculating this gives:
p=2.00×10−5kg m/s
The kinetic energy (KE) is given by the formula:
KE=p22m
Substituting the values of p and m:
KE=(2.00×10−5)22×(10−7)
Calculating p2:
(2.00×10−5)2=4.00×10−10
Now substituting this into the kinetic energy formula:
KE=4.00×10−102×10−7=4.00×10−102.00×10−7=2.00×10−3J
The kinetic energy of the body is:
KE=2.00×10−3J
Similar Questions
A body of mass 50 kg has a momentum of 3000 kg ms−1. Calculate:
The kinetic energy of the body.
View SolutionThe only force acting on a 2.0−kg body as it moves along the x-axis varies as shown in figure. The velocity of the body at x=0 is 4.0ms−1.
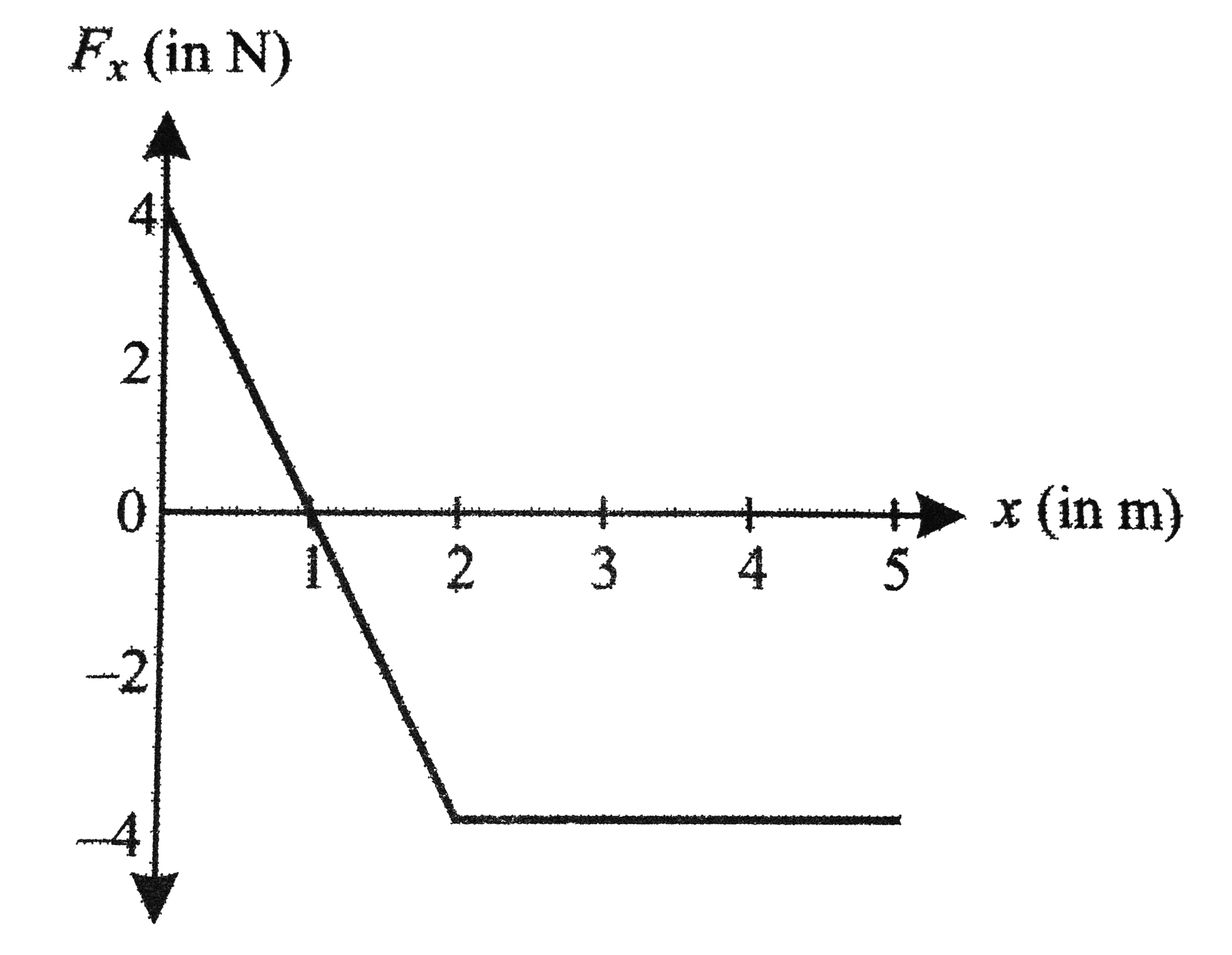
a. What is the kinetic energy of the body at x=3.0m?
b. At what value of x will the body have a kinetic energy of 8.0J?
c. What is the maximum kinetic energy attained by the body between x=0 and x=5.0m?
View SolutionA uniform force of 4 N acts on a body of mass 10 kg for a distance of 2.0 m . The kinetic energy acquired by the body is
View SolutionCalculate the wavelength a particle of mass m=6.6×10−27kg moving with kinetic energy 7.425×10−13J(h=6.6×10−34kgm2s−1
View SolutionA moving electron has 5×10−25Jof kinetic energy .What is the de Broglie wavelength ?
View SolutionA particle of mass m1=4kg moving at 6ˆims−1 collides perfectly elastically with a particle of mass m2=2 moving at 3ˆims−1
View SolutionCalculate de Broglie wavelenth of a neutron
(mass, m=1.6×10−27 kg) moving with kinetic energy of 0.04 eV.
View SolutionTwo bodies of masses m1andm2 have same kinetic energy. The ratio of their momentum is
View SolutionA body of mass 2 m moving with velocity v makes a head - on elastic collision with another body of mass m which is initially at rest. Loss of kinetic energy of the colliding body (mass 2 m) is
View SolutionA body of mass m moving with a velocity v is approaching a second object of same mass but at rest. The kinetic energy of the two objects as viewed from the centre of mass is
View Solution
