The instantaneous voltages at three terminals marked X, Y and Z are given by
VX=V0sinωt,VY=V0sin(ωt=2π3)andVz=V0sin(ωt=4π3)
An ideal voltmeter is configured to read runs value of the potential difference between its terminals. is connected between points X and Y and then between Y and Z. The reading the voltmeter will be
VX=V0sinωt,Vγ=V0sin(ωt+2π3)andVZ=V0sin(ωt+4π3)
VrmsXY=V0√32
VrmsYZ=V0√12
indpependent of the choice of the two terminals
The correct Answer is:A, C
To solve the problem, we need to calculate the potential difference readings of an ideal voltmeter connected between terminals X and Y, and then between terminals Y and Z. The instantaneous voltages at the terminals are given as follows:
1. VX=V0sin(ωt)
2. VY=V0sin(ωt+2π3)
3. VZ=V0sin(ωt+4π3)
Step 1: Calculate the potential difference VXY
The potential difference between terminals X and Y is given by:
VXY=VX−VY
Substituting the values of VX and VY:
VXY=V0sin(ωt)−V0sin(ωt+2π3)
Factoring out V0:
VXY=V0(sin(ωt)−sin(ωt+2π3))
Step 2: Apply the sine subtraction formula
Using the sine subtraction formula sinA−sinB=2cos(A+B2)sin(A−B2):
Let A=ωt and B=ωt+2π3:
VXY=V0⋅2cos⎛⎝(ωt)+(ωt+2π3)2⎞⎠sin⎛⎝(ωt)−(ωt+2π3)2⎞⎠
Calculating the averages:
A+B2=ωt+π3
A−B2=−π3
Thus,
VXY=V0⋅2cos(ωt+π3)sin(−π3)
Since sin(−π3)=−√32:
VXY=−V0⋅√3cos(ωt+π3)
Step 3: Calculate RMS value for VXY
The peak value of VXY is √3V0. The RMS value is given by:
VRMS,XY=Vpeak√2=√3V0√2=V0√3√2
Step 4: Calculate the potential difference VYZ
Now, we calculate the potential difference between terminals Y and Z:
VYZ=VY−VZ
Substituting the values of VY and VZ:
VYZ=V0sin(ωt+2π3)−V0sin(ωt+4π3)
Factoring out V0:
VYZ=V0(sin(ωt+2π3)−sin(ωt+4π3))
Step 5: Apply the sine subtraction formula again
Using the same sine subtraction formula:
Let A=ωt+2π3 and B=ωt+4π3:
VYZ=V0⋅2cos⎛⎝(ωt+2π3)+(ωt+4π3)2⎞⎠sin⎛⎝(ωt+2π3)−(ωt+4π3)2⎞⎠
Calculating the averages:
A+B2=ωt+π
A−B2=−π3
Thus,
VYZ=V0⋅2cos(ωt+π)sin(−π3)
Since cos(ωt+π)=−cos(ωt) and sin(−π3)=−√32:
VYZ=V0⋅2(−cos(ωt))(−√32)=V0√3cos(ωt)
Step 6: Calculate RMS value for VYZ
The peak value of VYZ is √3V0. The RMS value is given by:
VRMS,YZ=Vpeak√2=√3V0√2=V0√3√2
Conclusion
The readings of the voltmeter when connected between terminals X and Y, and then Y and Z, are both:
VRMS,XY=V0√3√2,VRMS,YZ=V0√3√2
2. VY=V0sin(ωt+2π3)
3. VZ=V0sin(ωt+4π3)
A−B2=−π3
A−B2=−π3
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - RESONANCE ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - PART-II
6 Videos
- ALTERNATING CURRENTBOOK - RESONANCE ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - HIGH LEVEL PROBLEMS
11 Videos
- ALTERNATING CURRENTBOOK - RESONANCE ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - PART-IV Comprehension
11 Videos
- ATOMIC PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Advanved level problems
17 Videos
Similar Questions
A,B and C are voltmeters of resistances R,1.5R and 3R respectively. When some potential difference is applied between x and y the voltmeter readings are VA,VBandVC, then
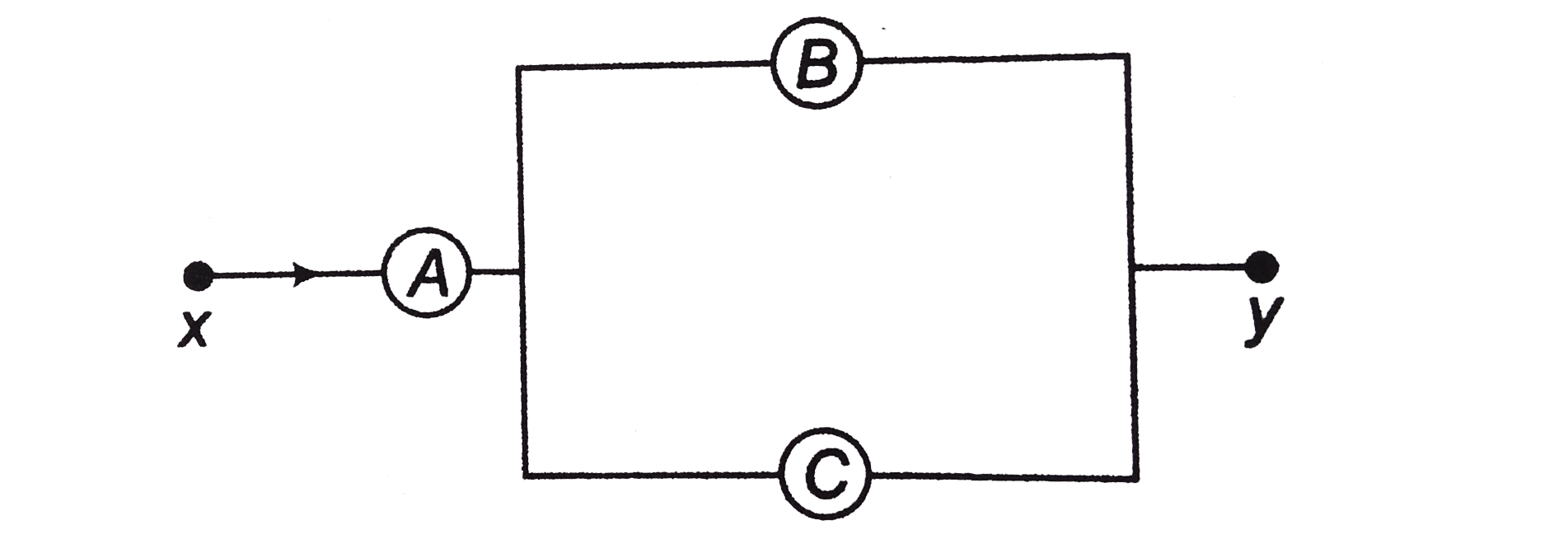
View SolutionA,B and C are voltmeters of resistances R,1.5R and 3R respectively. When some potential difference is applied between x and y the voltmeter readings are VA,V_BandV_C, then

View Solution
Knowledge Check
Three travelling waves are superimposed. The equations of the wave are
y!=A0sin(kx−ωt),y2=3√2A0sin(kx−ωt+ϕ) and y3=4A0cos(kx−ωt)
find the value of ϕ(given 0≤ϕ≤π/2) if the phase difference between the resultant wave and first wave is π/4Aπ6Bπ3Cπ12DNone of theseThe voltage over a cycle varies as
V=V0sinωt for 0≤t≤πω
=−V0sinωt for πω≤t≤2πω
The average value of the voltage one cycle isAV0√2BV02CzeroD2V0π
A,B and C are voltmeters of resistances R,1.5R and 3R respectively. When some potential difference is applied between x and y the voltmeter readings are VA,V_BandV_C, then

View SolutionA,B and C are voltmeters of resistances R,1.5R and 3R respectively. When some potential difference is applied between x and y the voltmeter readings are VA,V_BandV_C, then

View SolutionA voltmeter reads the potential difference across the terminals of an old battery as 1.40V, while a potentiometer reads its voltage to be 1.55V. The voltmeter resistance is 280Ω.
View SolutionAn Ac voltage V=V0sin100t is applied to the circuit, the phase difference between current and voltage is found to be π4, then
 .
.
View SolutionAn Ac voltage V=V0sin100t is applied to the circuit, the phase difference between current and voltage is found to be π4, then
 .
.
View SolutionThe voltage over a cycle varies as
V=V0sinωt for 0≤t≤πω
=−V0sinωt for πω≤t≤2πω
The average value of the voltage one cycle is
View Solution
