Two inductors L1 (inductance 1 mH, internal resistance 3Ω)and L2 (inductance 2 mH, internal resistance 4Ω), and a resistance R (resistance 12Ω)are all connected in parallel across a 5V battery . The circuit is switched on at time t=0. The ratio of the maximum to the minimum current (Imax/Imin) drawn from the battery is :
The correct Answer is:8
To solve the problem, we need to find the ratio of the maximum to the minimum current drawn from the battery when two inductors and a resistor are connected in parallel. Let's go through the solution step by step.
Step 1: Identify the Components
We have:
- Inductor L1 with inductance 1mH and internal resistance 3Ω
- Inductor L2 with inductance 2mH and internal resistance 4Ω
- Resistor R with resistance 12Ω
- Voltage of the battery E=5V
Step 2: Determine the Equivalent Resistance at t=0
At time t=0, the inductors behave like open circuits because they have not yet built up any current. Therefore, the only resistance in the circuit is the resistor R.
Req, max=R=12Ω
Step 3: Determine the Equivalent Resistance as t→∞
As time approaches infinity, the inductors behave like short circuits (i.e., they have zero resistance). We need to find the equivalent resistance of the circuit when both inductors are shorted.
The equivalent resistance Req, min for the parallel combination of the resistances is given by:
1Req, min=1R1+1R2+1R
Where:
- R1=3Ω (internal resistance of L1)
- R2=4Ω (internal resistance of L2)
- R=12Ω (external resistance)
Substituting the values:
1Req, min=13+14+112
Finding a common denominator (which is 12):
1Req, min=412+312+112=812
Thus,
Req, min=128=1.5Ω
Step 4: Calculate Maximum and Minimum Currents
Using Ohm's Law, the current drawn from the battery can be calculated as:
- Maximum current Imax when Req=Req, max:
Imax=EReq, max=5V12Ω=512A
- Minimum current Imin when Req=Req, min:
Imin=EReq, min=5V1.5Ω=51.5=103A
Step 5: Calculate the Ratio of Maximum to Minimum Current
Now, we can find the ratio of maximum to minimum current:
ImaxImin=512103=512×310=15120=18
Thus, the ratio of maximum to minimum current is:
ImaxImin=18
Final Answer
The ratio of the maximum to the minimum current drawn from the battery is 18.
---
We have:
- Inductor L1 with inductance 1mH and internal resistance 3Ω
- Inductor L2 with inductance 2mH and internal resistance 4Ω
- Resistor R with resistance 12Ω
- Voltage of the battery E=5V
At time t=0, the inductors behave like open circuits because they have not yet built up any current. Therefore, the only resistance in the circuit is the resistor R.
As time approaches infinity, the inductors behave like short circuits (i.e., they have zero resistance). We need to find the equivalent resistance of the circuit when both inductors are shorted.
- R1=3Ω (internal resistance of L1)
- R2=4Ω (internal resistance of L2)
- R=12Ω (external resistance)
Using Ohm's Law, the current drawn from the battery can be calculated as:
Now, we can find the ratio of maximum to minimum current:
The ratio of the maximum to the minimum current drawn from the battery is 18.
Similar Questions
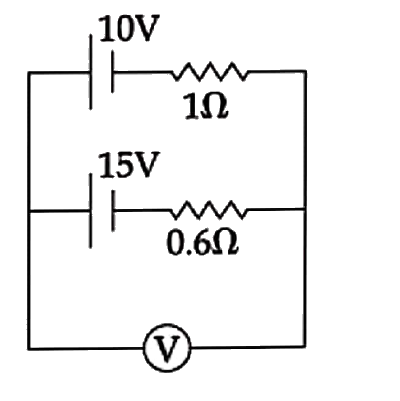
A 10 V battery with internal resistance 1Ω and a 15 V battery with internal resistance 0.6Ω are connected in parallel to a voltmeter (see figure). The reading in the voltmeter will be close to:
View SolutionA coil of inductance 8.4mH and resistance 6 Ω is connected to a 12V battery. The current in the coil is 1.0A at approximately the time.
View Solution
Knowledge Check
7 V battery with internal resistance 2Ωand3V battery with internal resistance 1Ω are connected to a 10Ω resistors as shown in figure, the current in 10Ω resistor is
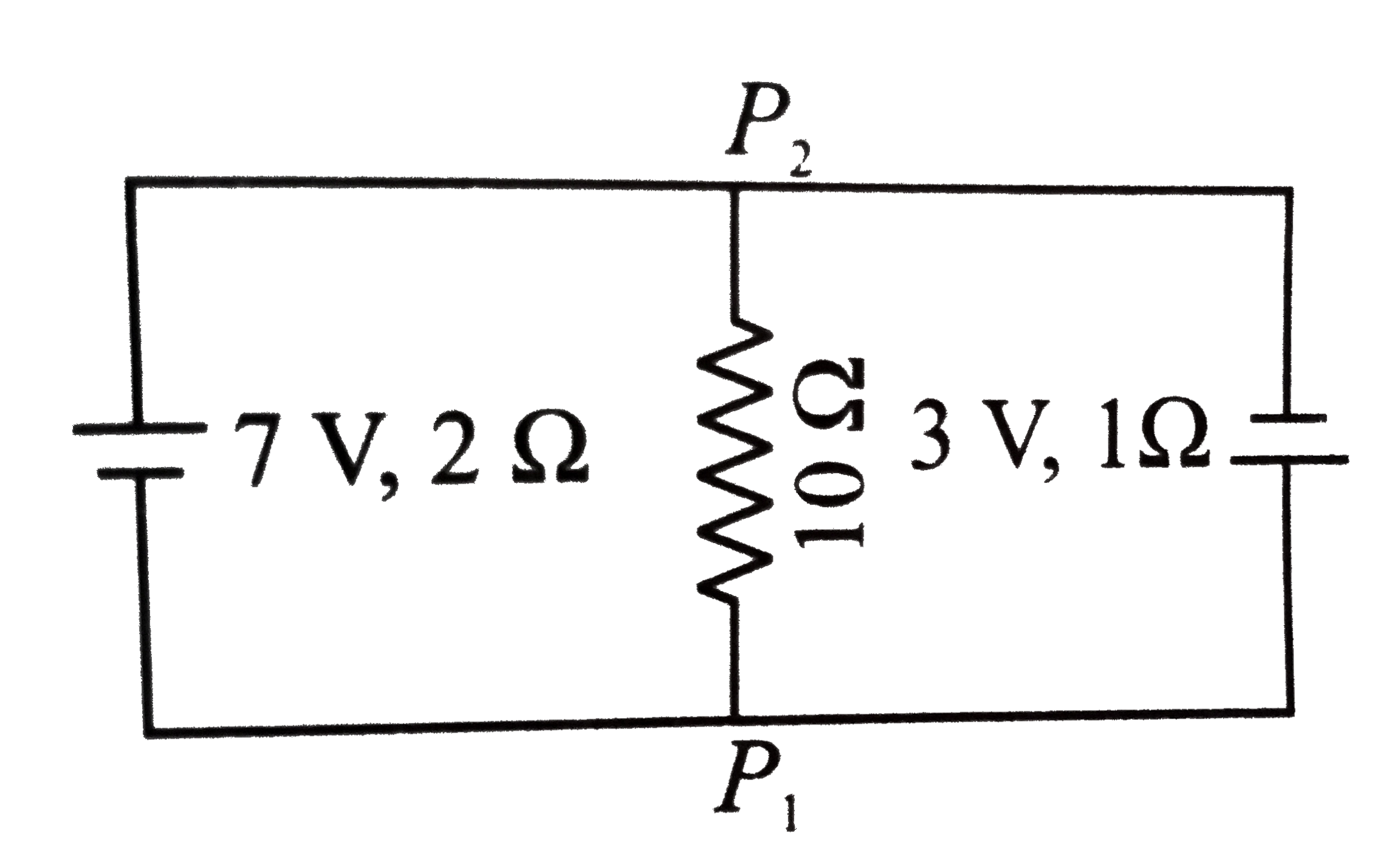 A0.27AB0.31AC0.031AD0.53
A0.27AB0.31AC0.031AD0.53
A coil of inductance 8.4 mH and resistance 6(Ω) is connected to a 12 V battery. The current in the coil is 1.0 A at approximately the time
View SolutionA 6 V cell with 0.5Ω internal resistance , a 10 v cell with 1Ω internal resistance and a 12Ω external resistance are connected in parallel . The current (in amper) through the 10 V cell is
View SolutionA 5V battery with internal resistance 2Ω and a 2V battery with internal resistance1Ω are connected to a 10Ω resistor as shown in the figure.
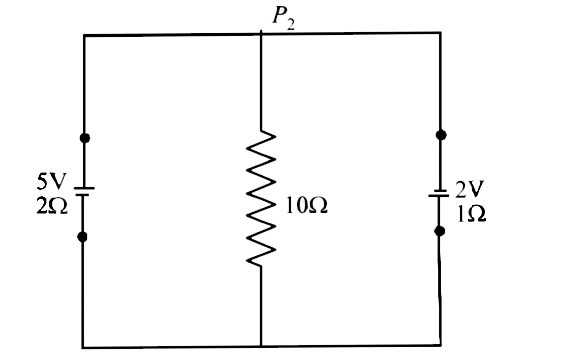
The current in the 10Ω resistor is
View SolutionA 5 V battery with internal resistance 1Ω and a 2 V battery with internal resistance 0.5Ω are connected to a 3Ω resistor as shown in the figure.The current in the 3Ω resistor is
View SolutionA uniformly wound solenoid coil of slf inductnace 4 mH and resistance 12Ω is broken int two parts. These two coil are connected in parallel across is 12V battery. The steady current through battery is
View SolutionTwo resistors of resistances 2Ω and 6Ω are connected in parallel. This combination is then connected to a battery of emf 2 V and internal resistance 0.5Ω. What is the current flowing through the battery ?
View Solution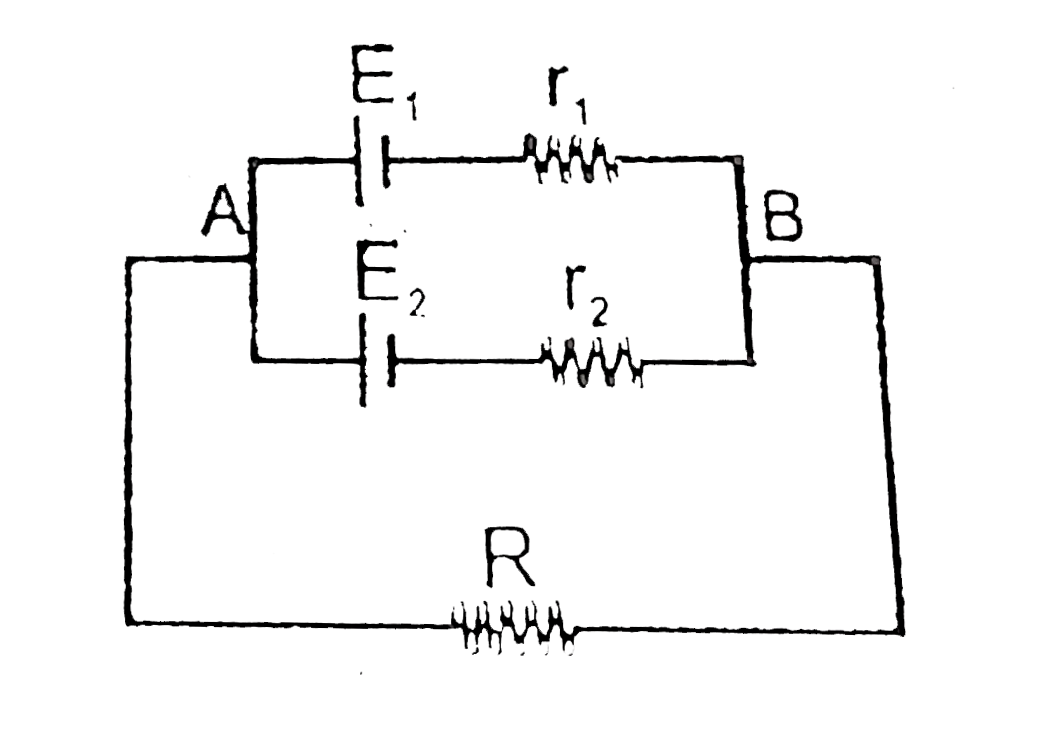
Two batteries of E1 internal resistance r1 & emf E2 internal resistance r2 are connected in parallel and makes a battery system. An external resistance R is connected across this system as shown in the figure.
View Solution

