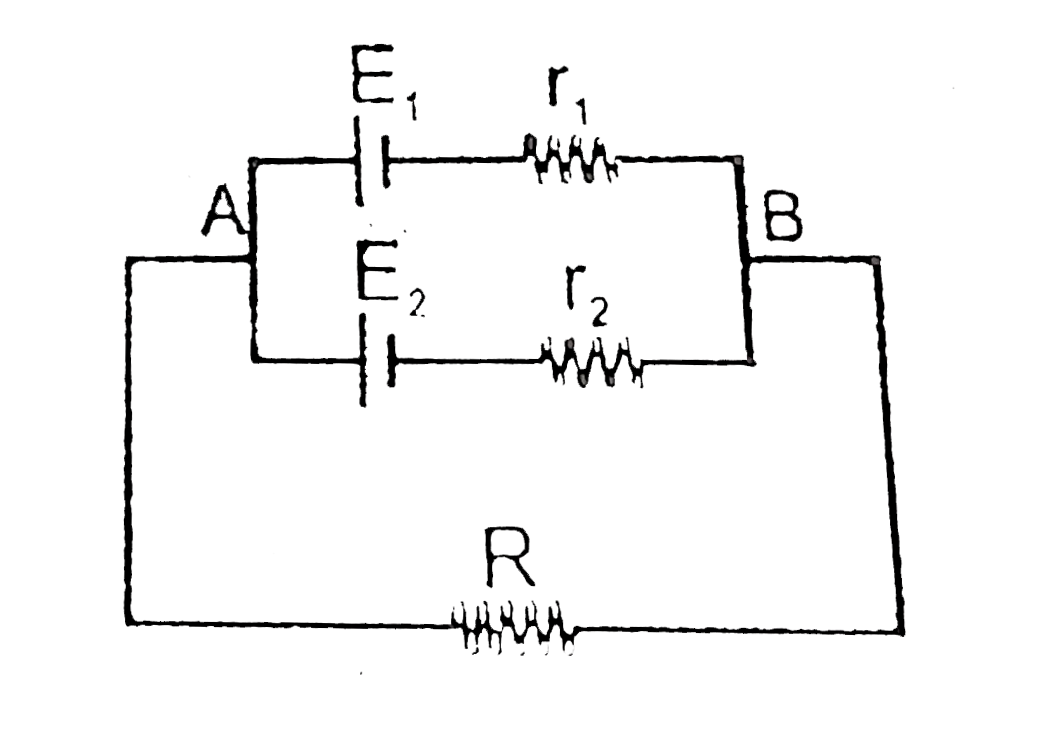
Two batteries of E1 internal resistance r1 & emf E2 internal resistance r2 are connected in parallel and makes a battery system. An external resistance R is connected across this system as shown in the figure.
if R is infinite the equivalent emf of system across A and B is E1r1+E2r2r1+r2
if R is infinite the equivalent emf of system across A and B is E1r2+E2r1r1+r2
The current in external circuit is given as E1r2+E2r2r1+r2R+r2r1r2+r1
The current in external circuit is given as E1r1+E2r21+Rr1+Rr2
Step by step video & image solution for Two batteries of E_(1) internal resistance r_(1) & emf E_(2) internal resistance r_(2) are connected in parallel and makes a battery system. An external resistance R is connected across this system as shown in the figure. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TEST PAPERSBook:RESONANCEChapter:TEST PAPERSExercise:PT-01
Explore 30 Videos
- TEST PAPERSBook:RESONANCEChapter:TEST PAPERSExercise:Pt-02
Explore 30 Videos
- TEST PAPERSBook:RESONANCEChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 107 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCEChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCEChapter:TEST SERIESExercise:PHYSICS
Explore 127 Videos
Similar Questions
Knowledge Check
Two batteris of emf ε1 and ε2 wit respective internal resistance r1 and r2 are connected in parallel. Now
AThe effective emf of this parallel combination is (ε1r1+ε2r2)(r1+r2)BThe effective internal resistance of this combination is 2r1r2(r1+r2)Cthe effective emf of this parallel combination is (ε1r2+ε2r1)(r1+r2)DThe effective internal resistance of this combination is r1r2(r1+r2)Two batteries of difference emf's and internal resistance are connected in parallel to one another.
AEquivalent emf will be grater than either of the emf'sBEquivalent emf will be smaller than either of the emfCEquivalent internal resistance will be grater than either of the two internal resistancesDEquivalent internal resistance will be smaller than either of the two internal resistances.N batteries each of emf E and internal resistance r are first connected in series and then in parallel to an external resistance R. if current through R is same in both cases then.
AR=r2/NBR−Nr/2CR=NrDR=r
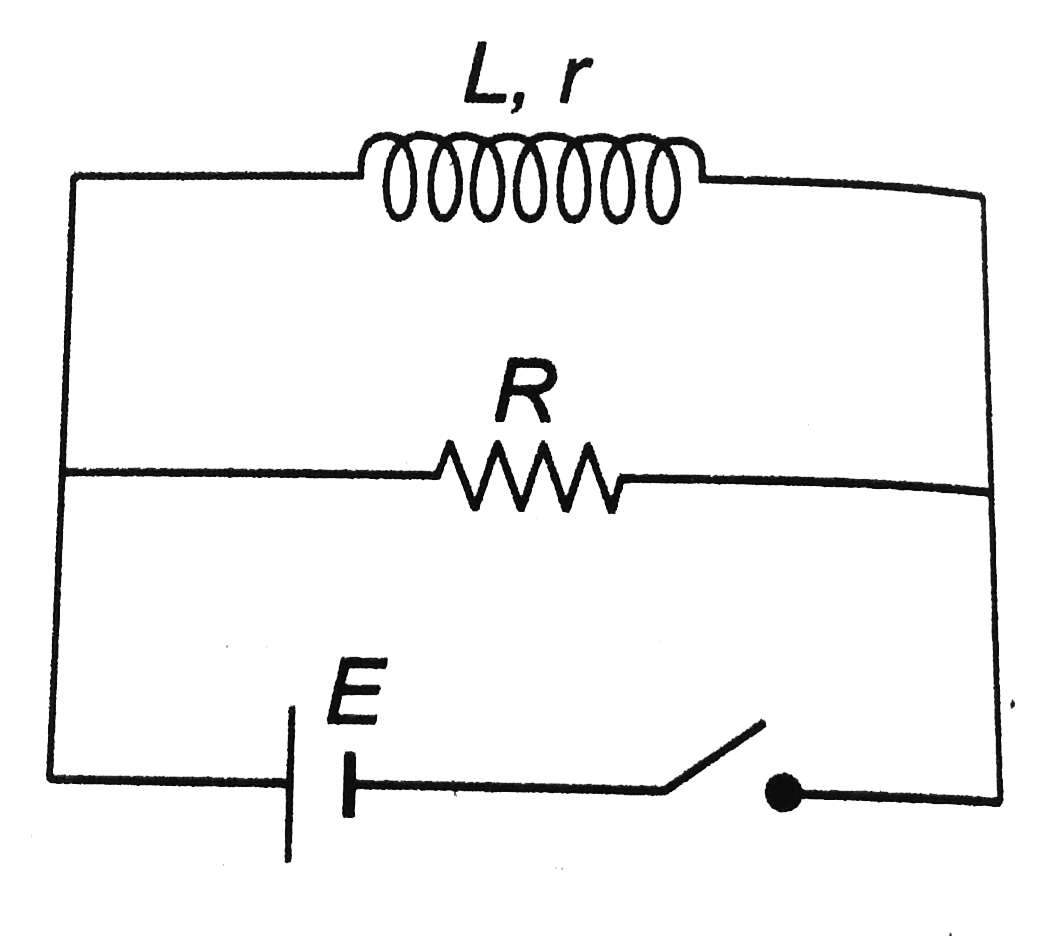
A solenoid of inductance L with resistance r is connected in parallel to a resistance R. A battery of emf E and of negligible internal resistance is connected across the parallel combination as shown in the figure. At time t=0, switch S is opened, calculate
(a) current through the solenoid after the switch is opened.
(b) amount of heat generated in the solenoid.
View SolutionTwo cells of emf E1andE2 having internal resistance r1andr2 are connected in parallel. Calculate Eeq and req for the combination.
View SolutionTwo cells of equal e.m.f. E b ut of difference internal resistance r1 and r2 are connected in parallel and the combination is connected with an external resistance R to obtain maximum power in R. find the value of R

View Solution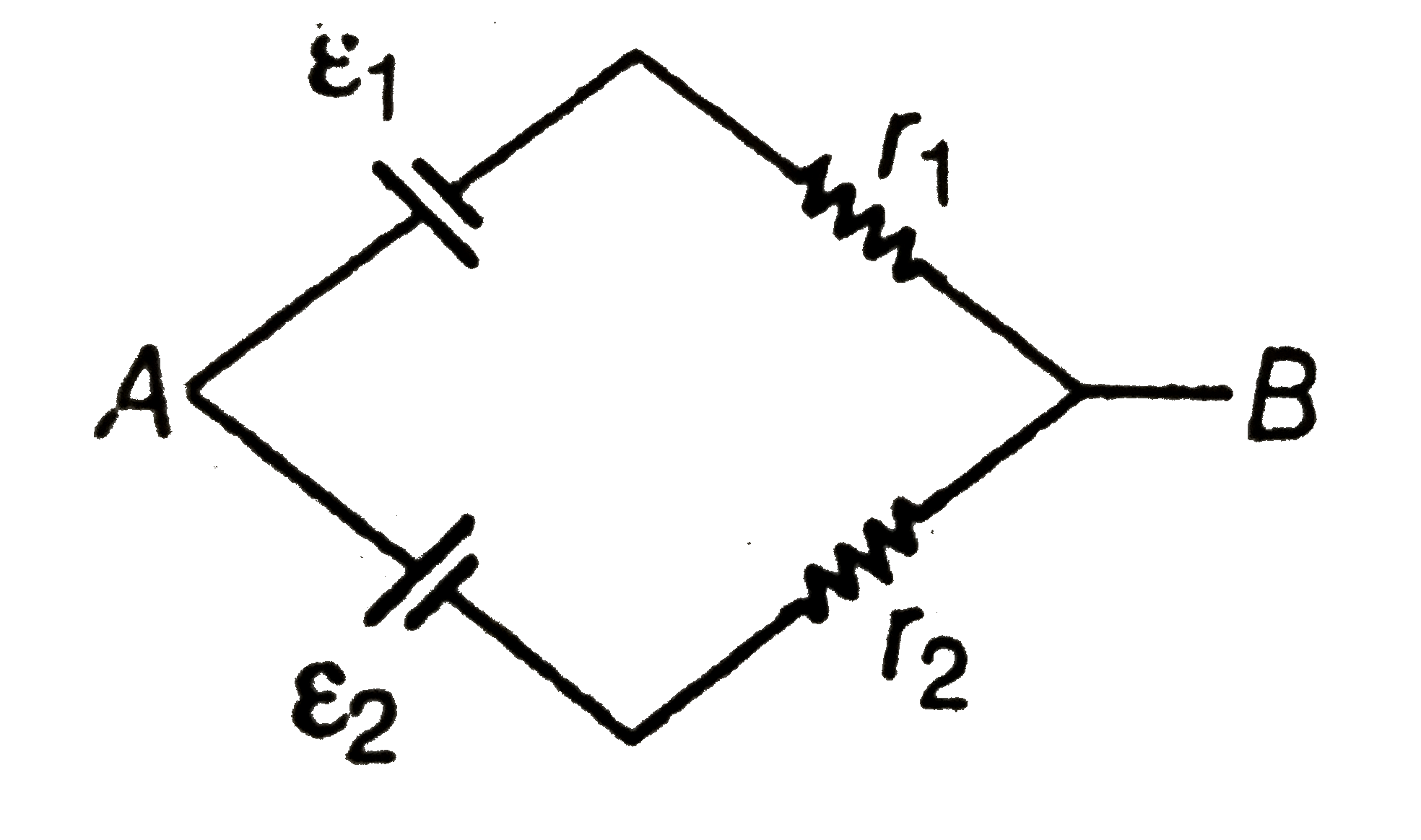
Two batteries of emf ε1andε2(ε2>ε1) and internal resistance r1andr2 respectively are connected in parallel as shown in figure.
View SolutionFour cells, each of emf E and internal resistance r, are connected in series across an external resistance reverse. Then, the current in the external circuit is
View Solution