A semicircular ring of radius 0.5 m is uniformly charged with a total charge of 1.5×10−9 coul. The electric potential at the centre of this ring is :
27 V
13.5 V
54 V
45.5 V
The correct Answer is:A
To find the electric potential at the center of a uniformly charged semicircular ring, we can follow these steps:
1. Identify Given Values:
- Radius of the semicircular ring, r=0.5m
- Total charge on the ring, Q=1.5×10−9C
2. Understanding Electric Potential:
- The electric potential V at a point due to a charge distribution is given by the formula:
V=k∫dqr
- Here, k is Coulomb's constant, k=9×109N m2/C2, and r is the distance from the charge element dq to the point where the potential is being calculated.
3. Linear Charge Density:
- The linear charge density λ for the semicircular ring is given by:
λ=QL
- The length L of the semicircular ring is πr (since it is half of a full circle).
- Therefore, L=π×0.5=1.57m.
- Thus, the linear charge density is:
λ=1.5×10−9π×0.5=1.5×10−91.57≈9.55×10−10C/m
4. Electric Potential Calculation:
- The potential V at the center due to the entire semicircular ring can be calculated by integrating the contributions from each infinitesimal charge element dq:
V=k∫π0λrdθ
- Since r (the distance from the charge element to the center) is constant and equal to the radius of the ring, we can factor it out:
V=kλr∫π0dθ=kλr(θ)π0=kλrπ
5. Substituting Values:
- Substitute λ and r into the equation:
V=k(Qπr)rπ=kQ/r
- Now substituting the values:
V=(9×109)×(1.5×10−9)0.5
6. Calculating the Final Value:
- Simplifying:
V=(9×1.5)0.5=13.50.5=27V
Final Answer:
The electric potential at the center of the semicircular ring is 27 V.
---
- Radius of the semicircular ring, r=0.5m
- Total charge on the ring, Q=1.5×10−9C
- The electric potential V at a point due to a charge distribution is given by the formula:
V=k∫dqr
- Here, k is Coulomb's constant, k=9×109N m2/C2, and r is the distance from the charge element dq to the point where the potential is being calculated.
- The linear charge density λ for the semicircular ring is given by:
λ=QL
- The length L of the semicircular ring is πr (since it is half of a full circle).
- Therefore, L=π×0.5=1.57m.
- Thus, the linear charge density is:
λ=1.5×10−9π×0.5=1.5×10−91.57≈9.55×10−10C/m
- The potential V at the center due to the entire semicircular ring can be calculated by integrating the contributions from each infinitesimal charge element dq:
V=k∫π0λrdθ
- Since r (the distance from the charge element to the center) is constant and equal to the radius of the ring, we can factor it out:
V=kλr∫π0dθ=kλr(θ)π0=kλrπ
- Substitute λ and r into the equation:
V=k(Qπr)rπ=kQ/r
- Now substituting the values:
V=(9×109)×(1.5×10−9)0.5
- Simplifying:
V=(9×1.5)0.5=13.50.5=27V
The electric potential at the center of the semicircular ring is 27 V.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Part - II Section (D)
2 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Part - II Section (E)
2 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Part - II Section (B)
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
Charge q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
View SolutionA ring of charge with radius 0.5m has 0.002π m gap. If the ribg carries a charge of +1C the electric field at the center is

View SolutionAssertion : For a non-uniformly charged thin circular ring with net charge zero, the electric field at any point on axis of the ring is zero.
Reason : For a non-uniformly charged thin circular ring with net charge zero, the electric potential at each point on axis of the ring is zero.
View SolutionA half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
View SolutionA hollow metal sphere of radius 5cm is charged so that the potential on its surface is 10V. The potential at the centre of the sphere is
View SolutionA circular ring carries a uniformly distributed positive charge .The electric field(E) and potential (V) varies with distance (r ) from the centre of thr ring along its axis as
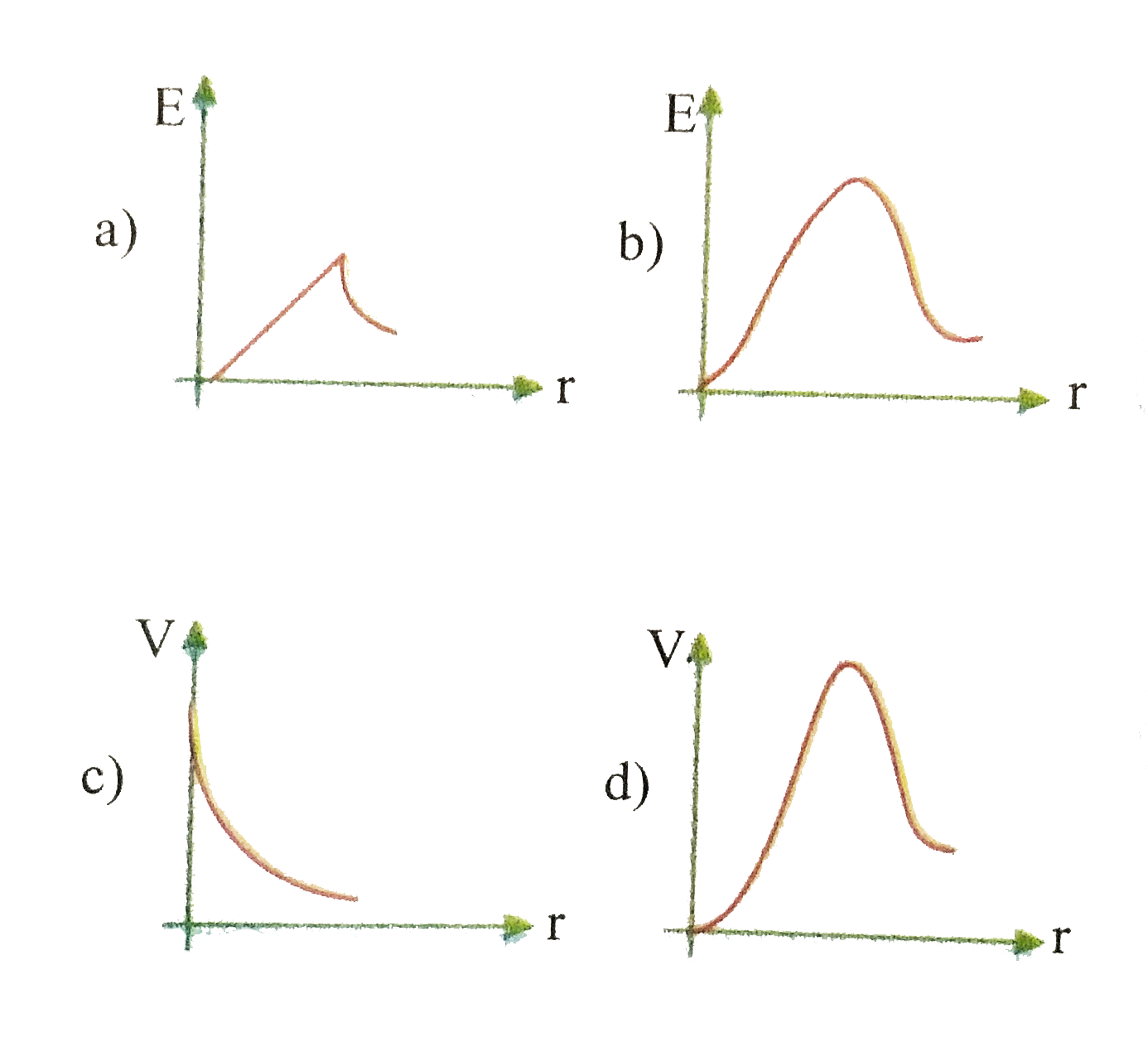
View SolutionA charge q is uniformly distributed over a quarter circular ring of radius r. The magnitude of electric field strength at the centre of the ring will be
View SolutionA ring of radius R carries a uniformly distributed charge +Q. A point charge −q is placed on the axis of the ring at a distance 2R from the centre of the ring and released from rest. The particle executes a simple harmonic motion along the axis of the ring. State True or False.
View SolutionA hollow metal sphere of radius 10 cm is charged such that the potential on its surface is 5 V. What is the potential at the centre of the sphere?
View SolutionA hollow metal sphere of radius 10 cm is charged such that the potential on its surface is 5 V. What is the potential at the centre of the sphere?
View Solution
