A charge q is uniformly distributed over a quarter circular ring of radius r. The magnitude of electric field strength at the centre of the ring will be
√2qπɛ0r2
q4πɛ0r2
q√2π2ɛ0r2
qπ2ɛ0r2
To find the magnitude of the electric field strength at the center of a quarter circular ring of radius r with a uniformly distributed charge q, we can follow these steps:
Step 1: Understand the Geometry
The quarter circular ring has a radius r and carries a total charge q. The center of the ring is the point where we want to calculate the electric field.
Step 2: Electric Field Contribution from a Small Element
Consider a small charge element dq on the ring. The electric field dE produced by this charge at the center of the ring can be resolved into two components: one along the x-axis (dEx) and one along the y-axis (dEy).
Step 3: Symmetry of the Problem
Due to the symmetry of the quarter ring, the horizontal components (dEx) from opposite sides will cancel out, while the vertical components (dEy) will add up.
Step 4: Expression for Electric Field from a Small Charge
The electric field dE due to the small charge dq at a distance r from the center is given by:
dE=14πϵ0dqr2
Here, r is the radius of the ring.
Step 5: Total Charge on the Quarter Ring
The total charge q is uniformly distributed over the quarter ring. The linear charge density λ can be expressed as:
λ=qL
where L is the length of the quarter ring, which is 14×2πr=πr2. Thus, we have:
λ=qπr2=2qπr
Step 6: Expressing dq
The small charge element dq can be expressed in terms of the linear charge density:
dq=λds
where ds is the infinitesimal arc length. For a quarter ring, ds=rdθ, where θ is the angle in radians.
Step 7: Total Electric Field Components
The vertical component dEy can be expressed as:
dEy=dEsin(θ)
The total vertical electric field Ey is obtained by integrating dEy over the quarter ring:
Ey=∫π20dEsin(θ)
Step 8: Integrating Over the Quarter Ring
Substituting dE and dq into the integral:
Ey=∫π2014πϵ0dqr2sin(θ)
Substituting dq=λrdθ:
Ey=∫π2014πϵ0λrdθr2sin(θ)
This simplifies to:
Ey=14πϵ0λr∫π20sin(θ)dθ
Step 9: Evaluate the Integral
The integral ∫π20sin(θ)dθ=1. Thus:
Ey=14πϵ0λr
Step 10: Substitute λ
Substituting λ=2qπr:
Ey=14πϵ02qπr2
This gives:
Ey=q2π2ϵ0r2
Step 11: Total Electric Field
Since Ex and Ey are equal due to symmetry, the total electric field E at the center is:
E=√E2x+E2y=√2E2y=√2Ey
Substituting Ey:
E=√2⋅q2π2ϵ0r2=q√22π2ϵ0r2
Final Result
Thus, the magnitude of the electric field strength at the center of the quarter circular ring is:
E=q2π2ϵ0r2
The quarter circular ring has a radius r and carries a total charge q. The center of the ring is the point where we want to calculate the electric field.
Consider a small charge element dq on the ring. The electric field dE produced by this charge at the center of the ring can be resolved into two components: one along the x-axis (dEx) and one along the y-axis (dEy).
Due to the symmetry of the quarter ring, the horizontal components (dEx) from opposite sides will cancel out, while the vertical components (dEy) will add up.
The electric field dE due to the small charge dq at a distance r from the center is given by:
dE=14πϵ0dqr2
Here, r is the radius of the ring.
The total charge q is uniformly distributed over the quarter ring. The linear charge density λ can be expressed as:
λ=qL
where L is the length of the quarter ring, which is 14×2πr=πr2. Thus, we have:
λ=qπr2=2qπr
The small charge element dq can be expressed in terms of the linear charge density:
dq=λds
where ds is the infinitesimal arc length. For a quarter ring, ds=rdθ, where θ is the angle in radians.
The vertical component dEy can be expressed as:
dEy=dEsin(θ)
The total vertical electric field Ey is obtained by integrating dEy over the quarter ring:
Ey=∫π20dEsin(θ)
Substituting dE and dq into the integral:
Ey=∫π2014πϵ0dqr2sin(θ)
Substituting dq=λrdθ:
Ey=∫π2014πϵ0λrdθr2sin(θ)
This simplifies to:
Ey=14πϵ0λr∫π20sin(θ)dθ
The integral ∫π20sin(θ)dθ=1. Thus:
Ey=14πϵ0λr
Substituting λ=2qπr:
Ey=14πϵ02qπr2
This gives:
Ey=q2π2ϵ0r2
Since Ex and Ey are equal due to symmetry, the total electric field E at the center is:
E=√E2x+E2y=√2E2y=√2Ey
Substituting Ey:
E=√2⋅q2π2ϵ0r2=q√22π2ϵ0r2
Thus, the magnitude of the electric field strength at the center of the quarter circular ring is:
E=q2π2ϵ0r2
Similar Questions
Charge q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
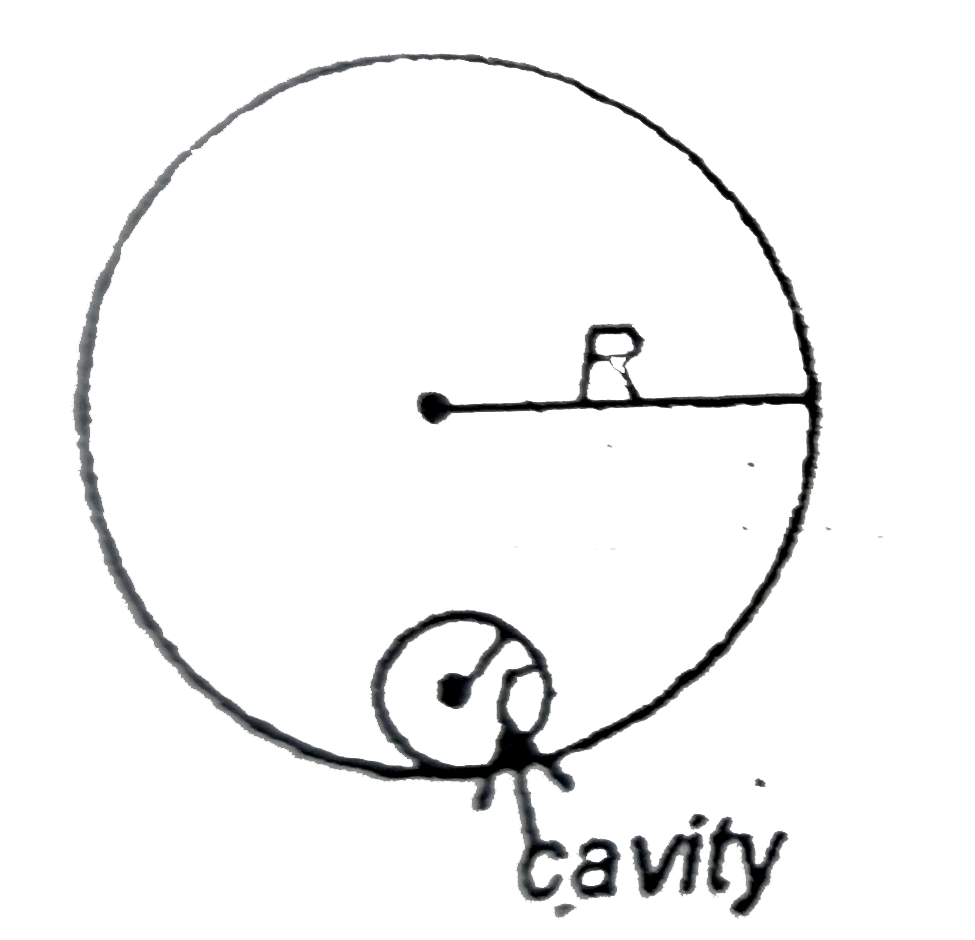
View SolutionA solid sphere of radius R has a spherical cavity of radius r as shown in the figure. If the volume charge density σ is uniformly distributed over the whole volume of the sphere, then electric field strength at the centre of the sphere will be
View SolutionA charge +Q is uniformly distributed over a thin ring of the radius R. The velocity of an electron at the moment when it passes through the centre O of the ring, if the electron was initially at far away on the axis of the ring is (m=mass of electron, K=14πε0)
View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring .The

View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring.

View SolutionA point charge q is located at the centre fo a thin ring of radius R with uniformly distributed charge −q, find the magnitude of the electric field strength vectro at the point lying on the axis of the ring at a distance x from its centre, if x>>R.
View SolutionA nonconducting ring of radius R has uniformly distributed positive charge Q. A small part of the ring.of length d, is removed (d<<R). The electric field at the centre of the ring will now be
View SolutionA charge q is unifomly distrybuted over a nonconducting ring of radius R. The ring is rotated about an axis passing through its centre and perpendicular to the plane of the ring with frequency f. The ratio of electric potential to the magnetic field at the centre of the ring depends on.
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionA non - conducting ring of mass m = 4 kg and radius R = 10 cm has charge Q = 2 C uniformly distributed over its circumference. The ring is placed on a rough horizontal surface such that the plane of the ring is parallel to the surface. A vertical magnetic field B=4t3T is switched on at t = 0. At t = 5 s ring starts to rotate about the vertical axis through the centre. The coefficient of friction between the ring and the surface is found to be k24. Then the value of k is
View Solution
