Two uniformly charged non-conducting hemispherical shells each having uniform charge density σ and radius R from a complete sphere (not stuck together) and surround a concentric spherical conducting shell of radius R/2. If hemispherical parts are in equilibrium then minimum surface charge density of inner conducting shell is :
−2σ
−σ/2
−σ
2σ
The correct Answer is:A
To solve the problem, we need to find the minimum surface charge density of the inner conducting shell when the two hemispherical shells are in equilibrium. Here’s a step-by-step solution:
Step 1: Understand the Configuration
We have two non-conducting hemispherical shells with uniform charge density σ and radius R. They form a complete sphere around a concentric conducting shell of radius R2.
Step 2: Calculate the Electric Field due to the Hemispherical Shells
The electric field E due to a uniformly charged hemispherical shell at its flat surface can be derived from the formula for electric field due to a charged surface. The electric field at the center of a hemispherical shell is given by:
E=σ2ϵ0
However, since we have two hemispherical shells, the net electric field at the center (due to both shells) will be:
Enet=E1+E2=σ2ϵ0+σ2ϵ0=σϵ0
Step 3: Calculate the Electric Pressure
The electric pressure P on the surface of the hemispherical shell can be expressed as:
P=σ22ϵ0
Step 4: Relate the Forces
For the hemispherical shells to be in equilibrium, the force of repulsion between the two hemispherical shells must be balanced by the attractive force from the inner conducting shell. The force F on one hemispherical shell due to the electric pressure can be calculated as:
F=P⋅A
where A is the area of the flat circular face of the hemisphere, which is πR2. Thus:
F=σ22ϵ0⋅πR2
Step 5: Calculate the Force from the Inner Conducting Shell
The inner conducting shell will have a surface charge density σ′. The electric field E′ due to the conducting shell at its surface is given by:
E′=σ′ϵ0
The force F′ on the hemispherical shell due to this electric field is:
F′=E′⋅A=σ′ϵ0⋅πR2
Step 6: Set Forces Equal for Equilibrium
For equilibrium, we set the repulsive force equal to the attractive force:
σ22ϵ0⋅πR2=σ′ϵ0⋅π(R2)2
Simplifying this gives:
σ22=σ′4
Thus,
σ′=2σ2
Conclusion
The minimum surface charge density of the inner conducting shell is:
σ′=2σ2
We have two non-conducting hemispherical shells with uniform charge density σ and radius R. They form a complete sphere around a concentric conducting shell of radius R2.
The electric field E due to a uniformly charged hemispherical shell at its flat surface can be derived from the formula for electric field due to a charged surface. The electric field at the center of a hemispherical shell is given by:
E=σ2ϵ0
However, since we have two hemispherical shells, the net electric field at the center (due to both shells) will be:
Enet=E1+E2=σ2ϵ0+σ2ϵ0=σϵ0
The electric pressure P on the surface of the hemispherical shell can be expressed as:
P=σ22ϵ0
For the hemispherical shells to be in equilibrium, the force of repulsion between the two hemispherical shells must be balanced by the attractive force from the inner conducting shell. The force F on one hemispherical shell due to the electric pressure can be calculated as:
F=P⋅A
where A is the area of the flat circular face of the hemisphere, which is πR2. Thus:
F=σ22ϵ0⋅πR2
The inner conducting shell will have a surface charge density σ′. The electric field E′ due to the conducting shell at its surface is given by:
E′=σ′ϵ0
The force F′ on the hemispherical shell due to this electric field is:
F′=E′⋅A=σ′ϵ0⋅πR2
For equilibrium, we set the repulsive force equal to the attractive force:
σ22ϵ0⋅πR2=σ′ϵ0⋅π(R2)2
Simplifying this gives:
σ22=σ′4
Thus,
σ′=2σ2
The minimum surface charge density of the inner conducting shell is:
σ′=2σ2
Topper's Solved these Questions
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Part- II
Explore 20 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Part - IV
Explore 9 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Part - III
Explore 20 Videos
- ELECTROMAGNETIC INDUCTIONBook:RESONANCE ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:A.l.P
Explore 19 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCE ENGLISHChapter:EXPERIMENTAL PHYSICSExercise:PART -II
Explore 10 Videos
Similar Questions
Knowledge Check
Consider a thin spherical shell of radius R consisting of uniform surface charge density σ. The electric field at a point of distance x from its centre and outside the shell is
Ainversely proportional to σBdirectly proportional to x2Cdirectlr proportional to RDinversely proportional to x2
The electrostatic potential of a uniformly charged thin spherical shell of charge Q and radius R at a distance r from the centre
View SolutionThere are two thin concentric conducting shells of radii a and b(a<b) . Equal charges Q given to each shell. Find the charge distribution on the inner and outer shell.
View SolutionThere are two concentric conducting shells of radius R,2R respectively. Charge on each surface is as shown in the figure.
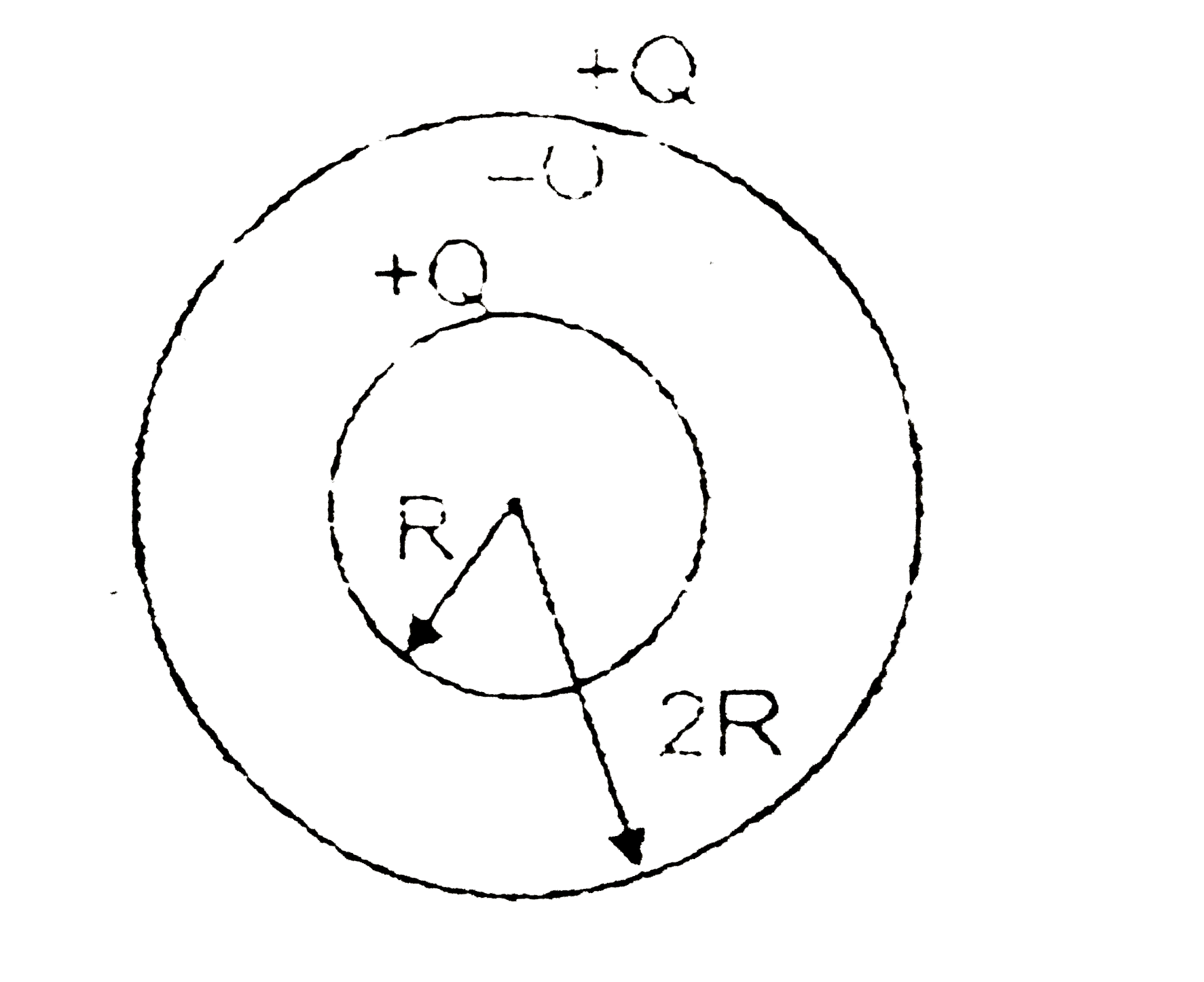
Equivalent capacitance of this system is:
View SolutionTwo concentric conducting shells of radii R and 2R are having charges Q and -2Q respectively.In a region r
View SolutionA charge q is placed at a distance of 2R from the centre of a spherical conducting shell of radius R. What is electrical potential, due to charges induced on the shell, at a point which is farthest from the point charge ?
View SolutionA conducting sphere of radius R, carrying charge Q, lies inside uncharged conducting shell of radius 2R. If they are joined by a metal wire,
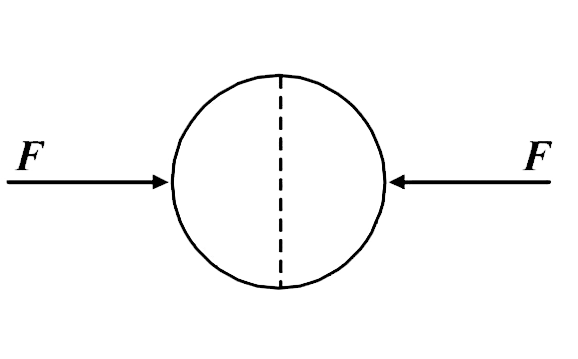
View SolutionA uniformly charged thin spherical shell of radius R carries uniform surface charge denisty of isgma per unit area. It is made of two hemispherical shells, held together by presisng them with force F(see figure). F is proportional to
View Solution

