In Rutherford formula, maximum number of a-particles deflecting is for which among the following angles θ:
30∘
45∘
60∘
90∘
To solve the problem regarding the maximum number of alpha particles deflecting in Rutherford's formula, we can follow these steps:
1. Understand the Relationship: According to Rutherford's formula, the number of alpha particles deflected is inversely proportional to sin4(θ/2). This means that as sin4(θ/2) increases, the number of deflected particles decreases.
2. Identify the Range of θ: The problem states that θ lies between 30 degrees and 90 degrees. Therefore, we can express this as:
30∘≤θ≤90∘
3. Transform the Angle: Since we are interested in sin4(θ/2), we need to calculate θ/2:
15∘≤θ2≤45∘
4. Analyze the Sine Function: The sine function increases from 0 to 1 as the angle increases from 0 to 90 degrees. Therefore, within the range of 15∘ to 45∘, sin(θ/2) will also increase.
5. Determine the Behavior of sin4(θ/2): Since sin(θ/2) is increasing, sin4(θ/2) will also be increasing. To minimize sin4(θ/2) (and thus maximize the number of deflected particles), we should choose the smallest angle in the range.
6. Find the Minimum Value: The minimum value of sin4(θ/2) occurs at the smallest angle, which is θ=30∘.
7. Conclusion: Therefore, the maximum number of alpha particles deflected occurs at θ=30∘.
Final Answer:
The maximum number of alpha particles deflecting is for θ=30∘.
---
30∘≤θ≤90∘
15∘≤θ2≤45∘
The maximum number of alpha particles deflecting is for θ=30∘.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
For which reaction among the following, is ΔS maximum ?
ACa(s)+12O2(g)→CaO(s)BCaCO3(s)→CaO(s)+CO2(g)CC(s)+O29g)→CO2(g)DN2(g)+O2(g)→2NO(g) - Question 2 - Select One
In the figure, which one of the following is the measure of angle θ?
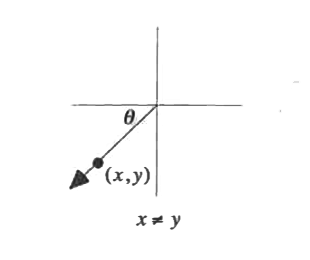 Aθ<45∘Bθ>45∘Cθ=45∘DIt cannot be determined from the information given
Aθ<45∘Bθ>45∘Cθ=45∘DIt cannot be determined from the information given
In which of the following bond angle is maximum
View SolutionIn which of the following bond angle is maximum ?
View SolutionRutherford's α scattering led to which of the following conclusions
View SolutionMaximum bond angle at nitrogen is present in which of the following ?
View SolutionWhich of the following has maximum angle strain ?
View SolutionWhich among the following has highest number of atoms?
View Solution

