The number of Br− Ions in 2.1 g CaBr2 is [NA=6×1023]
1.26×1022
6.3×1021
1.05×1021
1.2×1020
To find the number of Br− ions in 2.1 g of CaBr2, we can follow these steps:
Step 1: Calculate the molar mass of CaBr2
The molar mass of CaBr2 can be calculated as follows:
- Calcium (Ca) has a molar mass of approximately 40 g/mol.
- Bromine (Br) has a molar mass of approximately 80 g/mol. Since there are two bromine atoms in CaBr2, we multiply by 2.
So, the molar mass of CaBr2 is:
\(
\text{Molar mass of } CaBr2 = 40 \, \text{g/mol} + 2 \times 80 \, \text{g/mol} = 40 + 160 = 200 \, \text{g/mol}
\)
Step 2: Calculate the number of moles of CaBr2 in 2.1 g
Using the formula for moles:
\(
\text{Number of moles} = \frac{\text{mass (g)}}{\text{molar mass (g/mol)}}
\)
Substituting the values:
\(
\text{Number of moles of } CaBr2 = \frac{2.1 \, \text{g}}{200 \, \text{g/mol}} = 0.0105 \, \text{mol}
\)
Step 3: Calculate the number of moles of Br− ions
Since each molecule of CaBr2 contains 2 Br− ions, the number of moles of Br− ions will be:
\(
\text{Number of moles of } Br^- = 2 \times \text{Number of moles of } CaBr2 = 2 \times 0.0105 \, \text{mol} = 0.021 \, \text{mol}
\)
Step 4: Calculate the total number of Br− ions
To find the total number of Br− ions, we use Avogadro's number (NA=6×1023,ions/mol):
\(
\text{Total number of } Br^- \text{ ions} = \text{Number of moles of } Br^- \times N_A
\)
Substituting the values:
\(
\text{Total number of } Br^- \text{ ions} = 0.021 \, \text{mol} \times 6 \times 10^{23} \, \text{ions/mol} = 1.26 \times 10^{22} \, \text{ions}
\)
Final Answer
The total number of Br− ions in 2.1 g of CaBr2 is \( 1.26 \times 10^{22} \) ions.
---
The molar mass of CaBr2 can be calculated as follows:
- Calcium (Ca) has a molar mass of approximately 40 g/mol.
- Bromine (Br) has a molar mass of approximately 80 g/mol. Since there are two bromine atoms in CaBr2, we multiply by 2.
\(
\text{Molar mass of } CaBr2 = 40 \, \text{g/mol} + 2 \times 80 \, \text{g/mol} = 40 + 160 = 200 \, \text{g/mol}
\)
Using the formula for moles:
\(
\text{Number of moles} = \frac{\text{mass (g)}}{\text{molar mass (g/mol)}}
\)
Substituting the values:
\(
\text{Number of moles of } CaBr2 = \frac{2.1 \, \text{g}}{200 \, \text{g/mol}} = 0.0105 \, \text{mol}
\)
Since each molecule of CaBr2 contains 2 Br− ions, the number of moles of Br− ions will be:
\(
\text{Number of moles of } Br^- = 2 \times \text{Number of moles of } CaBr2 = 2 \times 0.0105 \, \text{mol} = 0.021 \, \text{mol}
\)
To find the total number of Br− ions, we use Avogadro's number (NA=6×1023,ions/mol):
\(
\text{Total number of } Br^- \text{ ions} = \text{Number of moles of } Br^- \times N_A
\)
Substituting the values:
\(
\text{Total number of } Br^- \text{ ions} = 0.021 \, \text{mol} \times 6 \times 10^{23} \, \text{ions/mol} = 1.26 \times 10^{22} \, \text{ions}
\)
The total number of Br− ions in 2.1 g of CaBr2 is \( 1.26 \times 10^{22} \) ions.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The number of atoms in 0.1 mole of a triatomic gas is (NA=6.02×1023mol−1)
A6.026×1022B1.806×1023C3.600×1023D1.800×1022
The number of electron in 3.1mgNO−3 is (NA = 6×1023 )
View SolutionThe total number of protons in 10 g of calcium carbonate is (N0=6.023×1023) :-
View Solution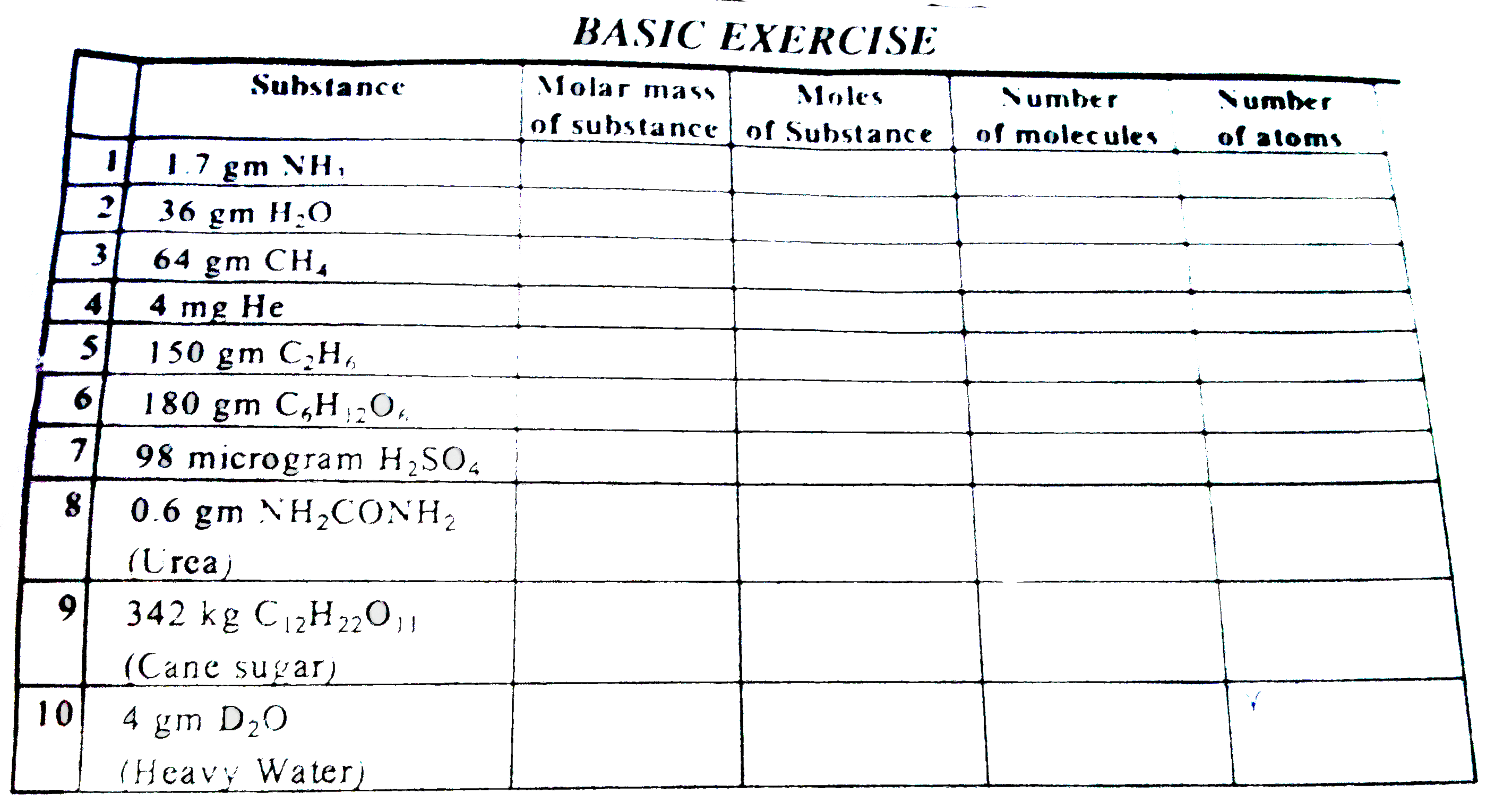
If a closed container contain 5 moles of CO2 gas, then find total number of CO2 molecules in the container (NA=6×1023)
View Solution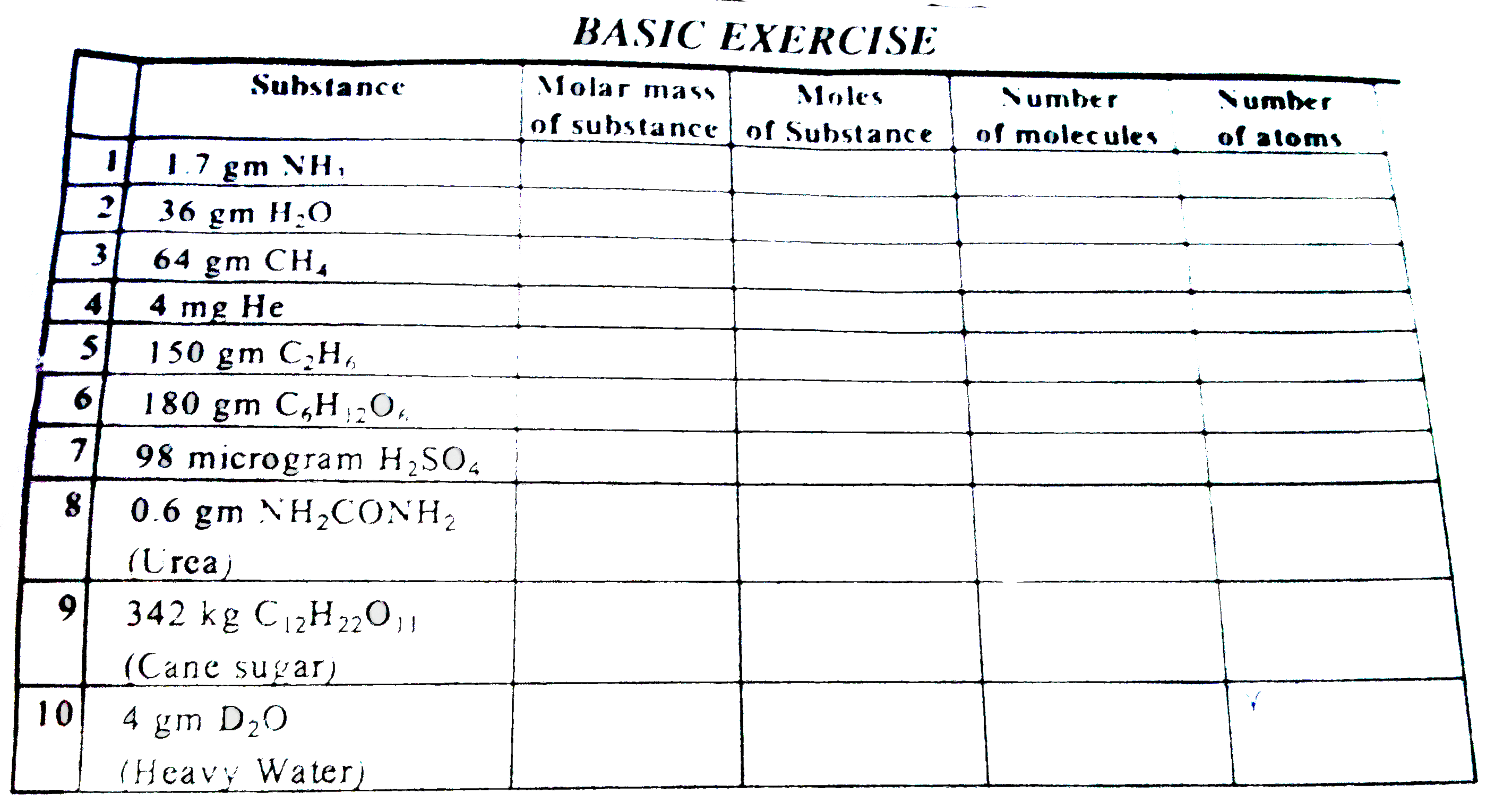
A box contains 12×1022 number of oxygen atoms. Find moles of O-atoms? (NA=6×1023)
View SolutionThe number of atoms in 0.1 mol of a tetraatomic gas is (NA=6.02×1023mol−1)
View SolutionIf NA is Avogadro's number then number of valence electrons in 4.2 g of nitride ions (N3−)
View SolutionThe total number of electrons in 4.2 g of N3− ion is (NA is the Avogadro's number)
View Solution
