Suppose a, b are integers and a + b is a root of x2+ax+b=0. What is the maximum possible values of b2 ?
The correct Answer is:81
To solve the problem, we need to find the maximum possible value of b2 given that a+b is a root of the equation x2+ax+b=0.
1. Identify the given quadratic equation:
The equation is x2+ax+b=0.
2. Substitute a+b as a root:
Since a+b is a root, we substitute x=a+b into the equation:
(a+b)2+a(a+b)+b=0
3. Expand the equation:
Expanding (a+b)2:
a2+2ab+b2+a2+ab+b=0
Combine like terms:
2a2+3ab+b2+b=0
4. Rearrange the equation:
Rearranging gives:
b2+(3a+1)b+2a2=0
This is a quadratic equation in b.
5. Use the quadratic formula:
The solutions for b can be found using the quadratic formula:
b=−(3a+1)±√(3a+1)2−4⋅1⋅2a22⋅1
6. Simplify the discriminant:
The discriminant D is:
D=(3a+1)2−8a2
Simplifying this:
D=9a2+6a+1−8a2=a2+6a+1
7. Condition for b to be an integer:
For b to be an integer, D must be a perfect square:
a2+6a+1=k2for some integer k
8. Rearranging gives a quadratic in a:
Rearranging gives:
a2+6a+(1−k2)=0
The discriminant of this quadratic must also be a perfect square:
36−4(1−k2)=4k2+32
9. Setting 4k2+32 to be a perfect square:
Let m2=4k2+32 for some integer m:
m2−4k2=32⇒(m−2k)(m+2k)=32
10. Finding pairs of factors of 32:
The pairs of factors of 32 are (1,32),(2,16),(4,8) and their negatives. Solving for m and k gives possible values.
11. Calculating values of b:
Substitute back to find b for each valid a derived from the factor pairs.
12. Finding maximum b2:
After calculating possible values of b for valid integers a, we find:
- For a=0, b=1 or −1 (values 0,1).
- For a=−6, b=9 or 8 (values 8,9).
The maximum value of b is 9.
13. Calculate b2:
The maximum value of b2:
b2=92=81
Conclusion:
The maximum possible value of b2 is 81.
The equation is x2+ax+b=0.
Since a+b is a root, we substitute x=a+b into the equation:
(a+b)2+a(a+b)+b=0
Expanding (a+b)2:
a2+2ab+b2+a2+ab+b=0
Combine like terms:
2a2+3ab+b2+b=0
Rearranging gives:
b2+(3a+1)b+2a2=0
This is a quadratic equation in b.
The solutions for b can be found using the quadratic formula:
b=−(3a+1)±√(3a+1)2−4⋅1⋅2a22⋅1
The discriminant D is:
D=(3a+1)2−8a2
Simplifying this:
D=9a2+6a+1−8a2=a2+6a+1
For b to be an integer, D must be a perfect square:
a2+6a+1=k2for some integer k
Rearranging gives:
a2+6a+(1−k2)=0
The discriminant of this quadratic must also be a perfect square:
36−4(1−k2)=4k2+32
Let m2=4k2+32 for some integer m:
m2−4k2=32⇒(m−2k)(m+2k)=32
The pairs of factors of 32 are (1,32),(2,16),(4,8) and their negatives. Solving for m and k gives possible values.
Substitute back to find b for each valid a derived from the factor pairs.
After calculating possible values of b for valid integers a, we find:
- For a=0, b=1 or −1 (values 0,1).
- For a=−6, b=9 or 8 (values 8,9).
The maximum value of b is 9.
The maximum value of b2:
b2=92=81
The maximum possible value of b2 is 81.
Topper's Solved these Questions
- EQUATIONS Book:RESONANCE ENGLISHChapter:EQUATIONS Exercise:EXERCISE-2 (PART-II: PREVIOUSLY ASKED QUESTION OF RMO)
Explore 5 Videos
- EQUATIONS Book:RESONANCE ENGLISHChapter:EQUATIONS Exercise:EXERCISE-1 (PART -II: RMO)
Explore 9 Videos
- DPPBook:RESONANCE ENGLISHChapter:DPPExercise:QUESTION
Explore 656 Videos
- FUNDAMENTAL OF MATHEMATICSBook:RESONANCE ENGLISHChapter:FUNDAMENTAL OF MATHEMATICSExercise:Exercise
Explore 135 Videos
Similar Questions
Knowledge Check
If 1−I is a root of the equation x2+ax+b=0 whereab∈R then value of a is
A−2B2C0Dnone of theseIf ab4=16 for positive integers a and b, what is one possible value of b ?
A3B1/2C4D20
IF 3+i is a root of the equation x2+ax+b=0 then a=
View Solution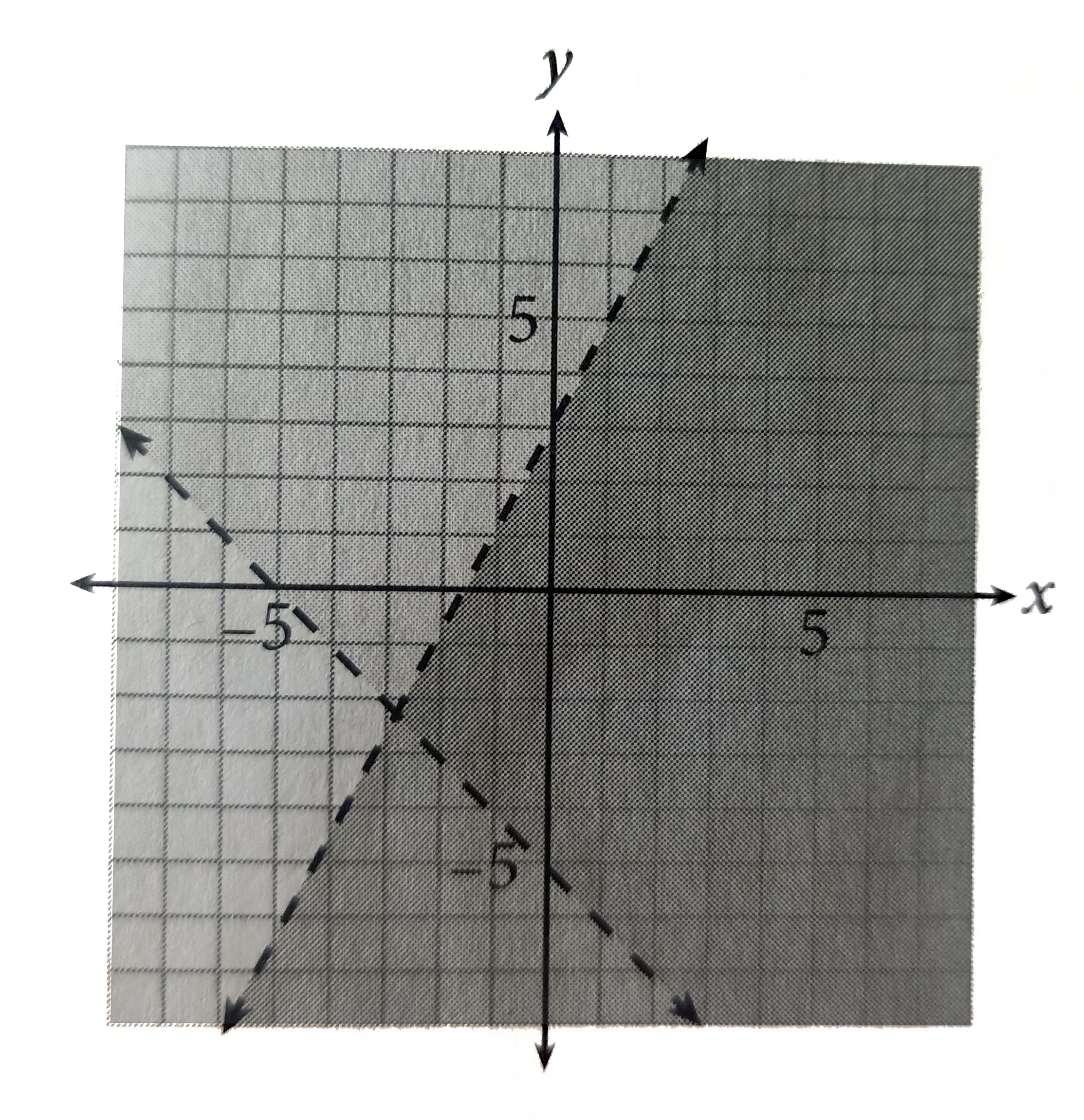
{y>−x−5y<2x+3
The figure above shows the solution for the system of inequalities shown. Suppose (a,b) is a solution to the system. If a=0, what is the greatest possible interger value of b ?
View SolutionStatement (1) : If a and b are integers and roots of x2+ax+b=0 are rational then they must be integers. Statement (2): If the coefficient of x2 in a quadratic equation is unity then its roots must be integers
View SolutionStatement (1) : If a and b are integers and roots of x2+ax+b=0 are rational then they must be integers. Statement (2): If the coefficient of x2 in a quadratic equation is unity then its roots must be integers
View SolutionIt is given that the equation x2+ax+20=0 has integer roots. What is the sum of all possible values of a ?
View SolutionIf a and b are positive integers such that the remainder is 4 when a is divided by b, what is the smallest possible value of a+b?
View Solution