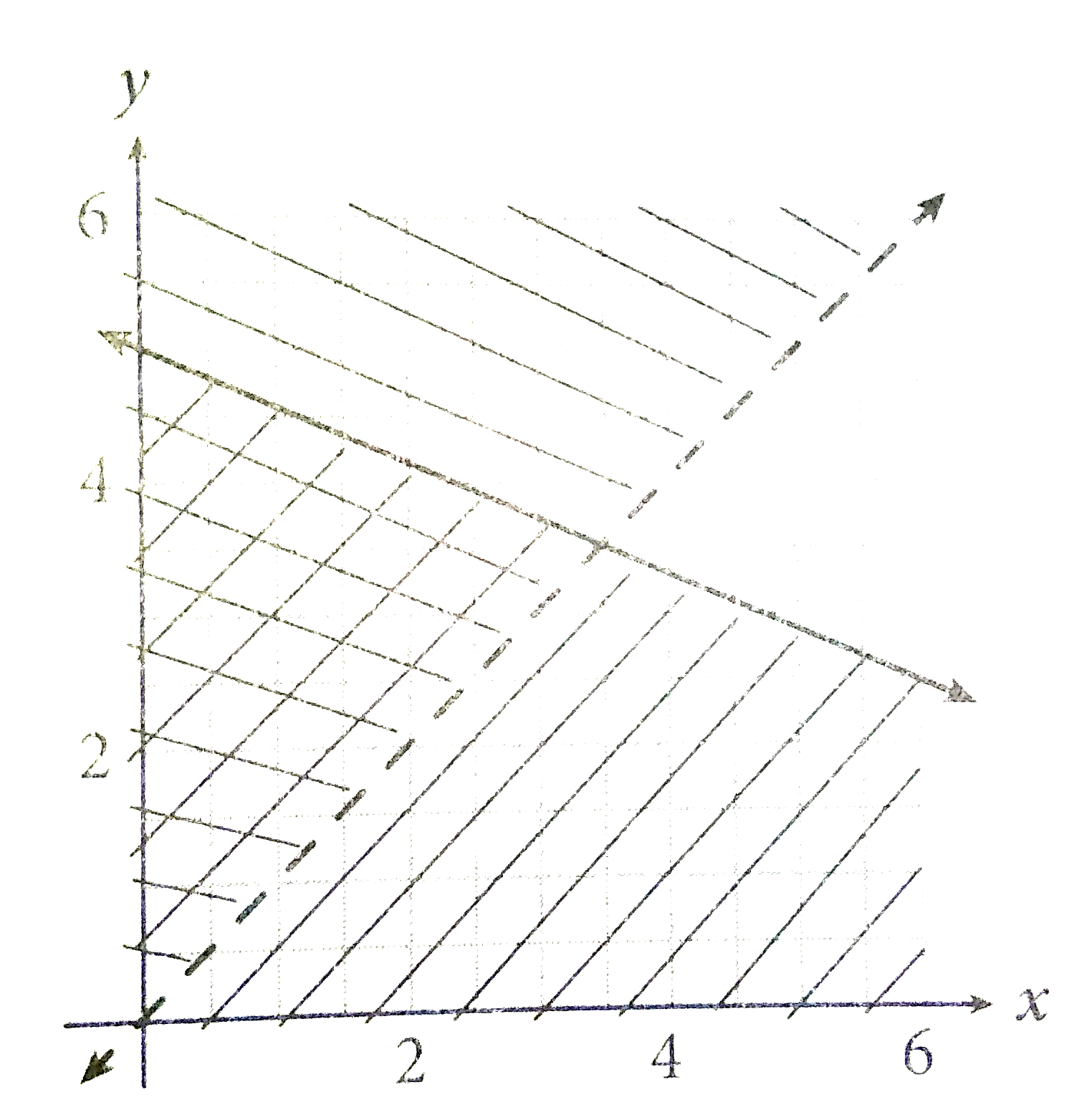
{y>−x−5y<2x+3
The figure above shows the solution for the system of inequalities shown. Suppose (a,b) is a solution to the system. If a=0, what is the greatest possible interger value of b ?
Video Solution
Text Solution
The correct Answer is:2
If (a,b) is a solution to the system, then a is the x-coordinate of any p[oint in the region where the shading overlaps, and b is the corrspojnding y-coordinate. When a=0(orx=o), then maximum possible value for b lies on the upper boundary line, y<2x+3. It looks like the y-coordinate is 3, but to be sure, substitute x=0 into the equation and simplify. You can replace the inequality symbol with an equal sign to make it an equatin because you are finding a point on the boundary line, not a range:
y=2(0)+3
y=3
The point on the boundary line is (0,3). The boundary line is dashed (because the inequality is strictly less than), so (0,3) is not a solution to the system. This means 2 is the greaterst possible integer value for b when a=0. Grid in 2.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - LINEAR INEQUALITIES1videos
INEQUALITIES
- BOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - SYSTEM OF INEQUALITIES1videos
INEQUALITIES
- BOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - MODELING REAL-LIFE SITUATIONS WITH INEQUALITIES1videos
INEQUALITIES
- BOOK - KAPLANCHAPTER - IMAGINARY NUMBERS EXERCISE - ARITHMETIC OPERATIONS WITH COMPLEX NUMBERS1videos
IMAGINARY NUMBERS
- BOOK - KAPLANCHAPTER - LINEAR EQUATIONS AND GRAPHSEXERCISE - LINEAR GRAPH1videos
LINEAR EQUATIONS AND GRAPHS
Similar Questions
Knowledge Check
- Question 1 - Select One
x+7y=−10
3x−4y=k
In the system of equation above,k is a constant. IF (a,b) is the solution to the system, what is the value of a, in terms of k?A−k−3025B3k+1025C6k−825D7k−4025 - Question 2 - Select One
y=3x+1
12y+x=1
In the system of equations above , if (x,y) is the solution to the system, what is the value of yx?A38B25C8D43 - Question 3 - Select One
xy=6
If (x,y) is the solution to the system of equations above, what is the value of y ?A2B4C12D24
x+2y=3
2x−y=8.5
If (x,y) is a solution to the above system of equtions, what is the value of x−y?View SolutionSolve the system of inequation: x+3>0,2x<14
View Solution−y≤6x−2200
3y≥9x−1500
Given the system of inequalities above, if point (a,b) lies within the solution set, what is the minimum possible value of b ?View Solution−2y≤8
y−3≤x
−13y+1≥x
Which of the following graphs shows the solutions to the system of inequalities above?View Solution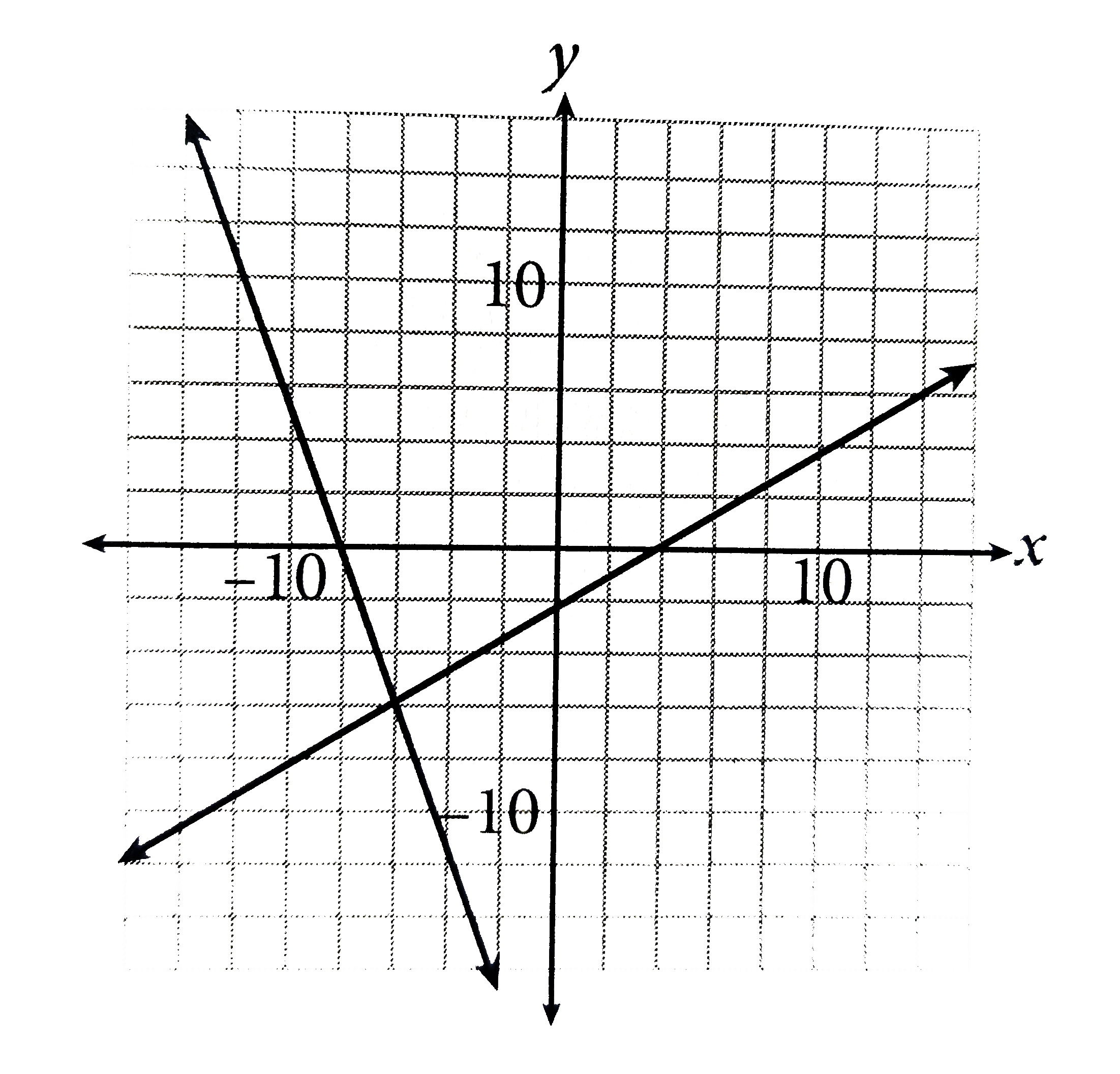
If (A,B) is the solution to the system of equations shown above, and A and B are intergers, then what is the value of A+B ?View Solution