Find the number of all integer-sided isosceles obtuse-angled triangles with perimeter 2008
The correct Answer is:86
To find the number of all integer-sided isosceles obtuse-angled triangles with a perimeter of 2008, we can follow these steps:
Step 1: Define the sides of the triangle
Let the sides of the isosceles triangle be x,x,y, where x is the length of the two equal sides and y is the length of the base.
Step 2: Set up the perimeter equation
The perimeter of the triangle is given by:
x+x+y=2008
This simplifies to:
2x+y=2008
From this, we can express y in terms of x:
y=2008−2x
Step 3: Apply the triangle inequality
For the triangle to be valid, it must satisfy the triangle inequality conditions. For our isosceles triangle, we have:
1. x+x>y or 2x>y
2. x+y>x (which is always true since y>0)
3. x+y>x (which is also always true)
From the first inequality 2x>y, substituting for y gives:
2x>2008−2x
Adding 2x to both sides:
4x>2008
Dividing by 4:
x>502
Step 4: Determine the range for y
Since y must also be positive, we have:
2008−2x>0⟹2008>2x⟹x<1004
Step 5: Combine inequalities
From the above steps, we have:
502<x<1004
Since x must be an integer, the possible integer values for x are:
x=503,504,…,1003
The total number of integer values for x can be calculated as:
1003−503+1=501
Step 6: Check for obtuse angle condition
For the triangle to be obtuse, we need to ensure that:
y2<2x2
Substituting y=2008−2x:
(2008−2x)2<2x2
Expanding and simplifying:
20082−2⋅2008⋅2x+4x2<2x2
20082−4016x+2x2<0
This is a quadratic inequality in x.
Step 7: Solve the quadratic inequality
To find the roots of the quadratic equation:
2x2−4016x+20082=0
Using the quadratic formula:
x=−b±√b2−4ac2a
where a=2,b=−4016,c=20082.
Calculating the discriminant:
D=(−4016)2−4⋅2⋅(20082)
We can find the roots and determine the valid integer values of x that satisfy the obtuse condition.
Conclusion
After calculating the valid integer values of x that satisfy both the triangle inequality and the obtuse angle condition, we find the total number of integer-sided isosceles obtuse-angled triangles with a perimeter of 2008.
Let the sides of the isosceles triangle be x,x,y, where x is the length of the two equal sides and y is the length of the base.
The perimeter of the triangle is given by:
x+x+y=2008
This simplifies to:
2x+y=2008
From this, we can express y in terms of x:
y=2008−2x
For the triangle to be valid, it must satisfy the triangle inequality conditions. For our isosceles triangle, we have:
1. x+x>y or 2x>y
2. x+y>x (which is always true since y>0)
3. x+y>x (which is also always true)
2x>2008−2x
Adding 2x to both sides:
4x>2008
Dividing by 4:
x>502
Since y must also be positive, we have:
2008−2x>0⟹2008>2x⟹x<1004
From the above steps, we have:
502<x<1004
Since x must be an integer, the possible integer values for x are:
x=503,504,…,1003
The total number of integer values for x can be calculated as:
1003−503+1=501
For the triangle to be obtuse, we need to ensure that:
y2<2x2
Substituting y=2008−2x:
(2008−2x)2<2x2
Expanding and simplifying:
20082−2⋅2008⋅2x+4x2<2x2
20082−4016x+2x2<0
This is a quadratic inequality in x.
To find the roots of the quadratic equation:
2x2−4016x+20082=0
Using the quadratic formula:
x=−b±√b2−4ac2a
where a=2,b=−4016,c=20082.
D=(−4016)2−4⋅2⋅(20082)
We can find the roots and determine the valid integer values of x that satisfy the obtuse condition.
After calculating the valid integer values of x that satisfy both the triangle inequality and the obtuse angle condition, we find the total number of integer-sided isosceles obtuse-angled triangles with a perimeter of 2008.
Topper's Solved these Questions
- COMBINATORICSBOOK - RESONANCE ENGLISHCHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-I: Previous Asked Question for Pre RMO)
17 Videos
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - High Level Problems (HLP)
35 Videos
- DEFINITE INTEGRATION & ITS APPLICATION BOOK - RESONANCE ENGLISHCHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - High Level Problem
26 Videos
Similar Questions
Find the ara of the given obtuse-angled triangle ABC.
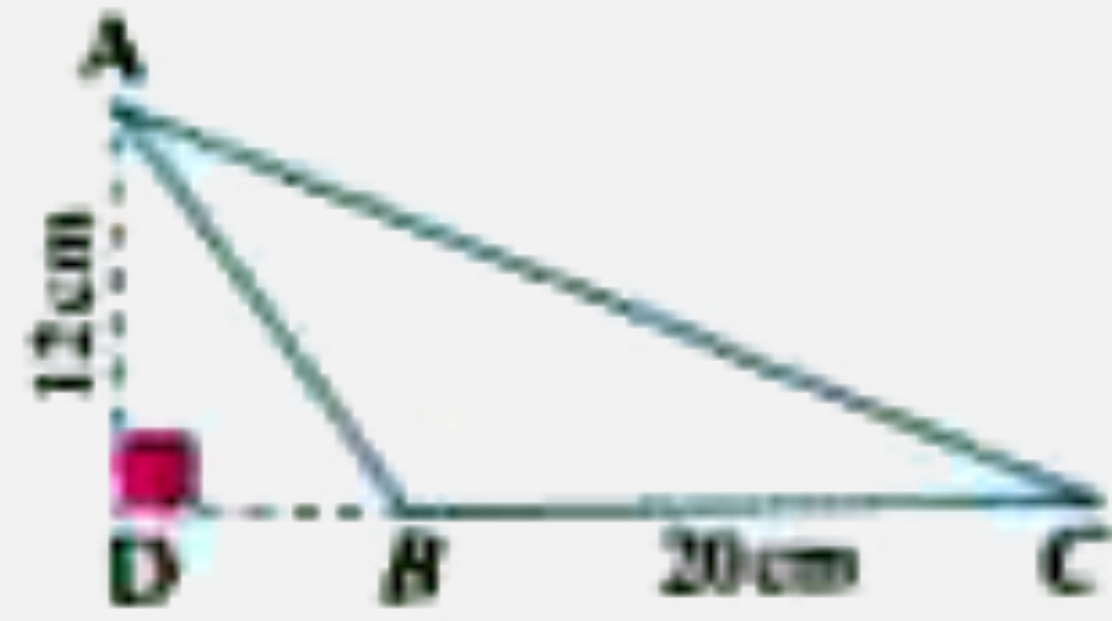
View SolutionIf the length of two sides of an isosceles triangle are 3 and 7, what is the perimeter of the triangle?
View SolutionFind the measures of each of the two equal angles of an isosceles right-angled triangle.
View SolutionFind the perimeter of the following shape.
An isosceles triangle with a base of 7cm and the two equal sides of 5 cm each
View SolutionFind the area of an isosceles triangle with perimeter 36 cm and base 16 cm.
View SolutionFind the perimeter of the following:
An equilateral triangle with each side 13 cm
View SolutionFind the altitude and area of an isosceles triangle whose perimeter is 64 cm and where base is 2 cm.
View SolutionFind the altitude and area of an isosceles triangle whose perimeter is 64 cm and whose base is 24 cm.
View SolutionFind the perimeter of an isosceles triangle with each equal side 13 cm and base 11 cm.
View SolutionFind the area of a figure formed by a square of side 8 cm and an isosceles triangle with base as one side of the square and perimeter as 18 cm.
View Solution
