For Bernoulli Distribution , state formula for E(X) and V(X)
The correct Answer is:E(X) = P, V(x) = pq
To find the expected value E(X) and variance V(X) for a Bernoulli distribution, we follow these steps:
Step 1: Define the Bernoulli Distribution
A Bernoulli distribution is a discrete probability distribution for a random variable which takes the value 1 with probability p (success) and the value 0 with probability 1−p (failure).
Step 2: Calculate the Expected Value E(X)
The expected value E(X) of a Bernoulli random variable can be calculated using the formula:
E(X)=∑xx⋅P(X=x)
For a Bernoulli distribution, this becomes:
E(X)=1⋅P(X=1)+0⋅P(X=0)=1⋅p+0⋅(1−p)=p
Step 3: Calculate the Variance V(X)
The variance V(X) of a Bernoulli random variable can be calculated using the formula:
V(X)=E(X2)−(E(X))2
For a Bernoulli distribution, since X can only take values 0 and 1:
E(X2)=12⋅P(X=1)+02⋅P(X=0)=1⋅p+0⋅(1−p)=p
Thus, we can substitute this into the variance formula:
V(X)=E(X2)−(E(X))2=p−p2
This can be simplified to:
V(X)=p(1−p)
Final Formulas
So, the formulas for the expected value and variance of a Bernoulli distribution are:
E(X)=p
V(X)=p(1−p)
A Bernoulli distribution is a discrete probability distribution for a random variable which takes the value 1 with probability p (success) and the value 0 with probability 1−p (failure).
The expected value E(X) of a Bernoulli random variable can be calculated using the formula:
E(X)=∑xx⋅P(X=x)
For a Bernoulli distribution, this becomes:
E(X)=1⋅P(X=1)+0⋅P(X=0)=1⋅p+0⋅(1−p)=p
The variance V(X) of a Bernoulli random variable can be calculated using the formula:
V(X)=E(X2)−(E(X))2
For a Bernoulli distribution, since X can only take values 0 and 1:
E(X2)=12⋅P(X=1)+02⋅P(X=0)=1⋅p+0⋅(1−p)=p
Thus, we can substitute this into the variance formula:
V(X)=E(X2)−(E(X))2=p−p2
This can be simplified to:
V(X)=p(1−p)
So, the formulas for the expected value and variance of a Bernoulli distribution are:
E(X)=p
V(X)=p(1−p)
Topper's Solved these Questions
- QUESTION BANK 2021Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:QUESTION BANK 2021Exercise:PROBABILITY DISTIBUTIONS
Explore 39 Videos
- PROBABILITY DISTRIBUTIONBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:PROBABILITY DISTRIBUTIONExercise:MULTIPLE CHOICE QUESTIONS
Explore 9 Videos
- THREE DIMENSIONAL GEOMETRY Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:THREE DIMENSIONAL GEOMETRY Exercise:MULTIPLE CHOICE QUESTIONS
Explore 8 Videos
Similar Questions
Knowledge Check
Bernoulli distribution is a particular case of binomial distribution if n = ...........
A4B10C2D1If x1andx2 are the means of two distributions such that x1<x2and¯x is the mean of the combined distribution, then
A¯x<¯x1B¯x>¯x2C¯x=¯x1+¯x22D¯x1<¯x<¯x2
Bernoulli Trial And Binomial Distribution
View SolutionFollowing is the distribution function F(x) of a discrete r.v. (1) Find the probability distribution of X.(ii) Find P(X≤3),P(2<x<5).(iii) Find P(X≤5∣X>3).
View SolutionA r.v X has the following probability distribution:
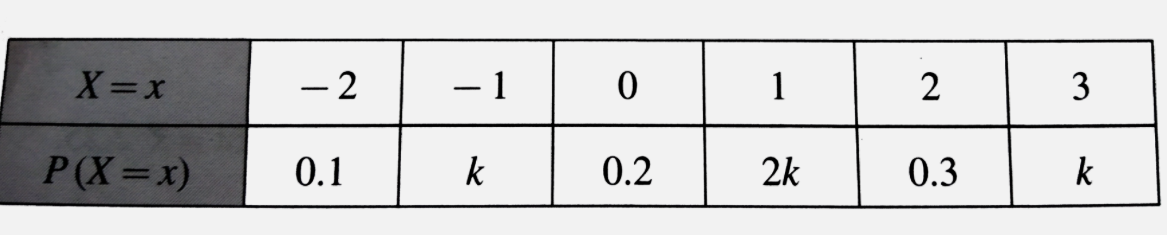
Find the value of k and calculate mean and varieance of X.
View SolutionBernoulli Trials and Binomial Distribution
View SolutionBernoulli trials and binomial distribution
View SolutionThe probability distribution of the random variable X is given by
Then, the value of V(X) is equal to
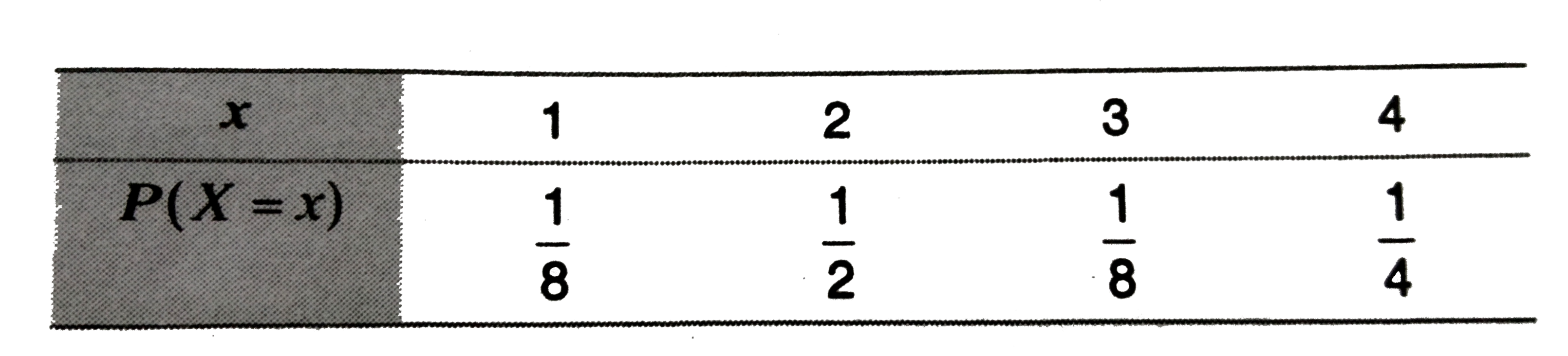
View Solution