An e.m. wave travels in vacuum along z direction:
→E=(E1ˆi+E2ˆj)cos(kz−ω). Choose the correct option from the following :
The associated magnetic field is given as ¯¯¯B=1C(E1ˆi+E2ˆj)cos(kz−ωt)
The associated magnetic field is given as ¯¯¯B=1C(E1ˆi−E2ˆj)cos(kz−ωt)
The given electromagnetic field is circularly polarised
The given electromagnetic field is not plane polarised
The correct Answer is:A
To solve the problem, we need to analyze the given electric field vector of the electromagnetic wave and determine the correct options based on its characteristics.
1. Identify the Electric Field Vector:
The electric field vector is given by:
→E=(E1^i+E2^j)cos(kz−ωt)
This indicates that the electric field has components in both the x-direction (i) and the y-direction (j).
2. Direction of Propagation:
The wave is traveling in the z-direction, as indicated by the argument of the cosine function, which contains kz−ωt. This means the wave propagates along the z-axis.
3. Determine the Magnetic Field Vector:
According to electromagnetic wave theory, the relationship between the electric field →E and the magnetic field →B in a vacuum is given by:
→B=1c→E
where c is the speed of light. Thus, we can express the magnetic field vector as:
→B=1c(E1^i+E2^j)cos(kz−ωt)
4. Check for Polarization:
The electric field vector has components in the x and y directions, which means it is oscillating in a plane. This indicates that the wave is plane-polarized.
5. Evaluate the Options:
- Option A: Since →B=1c→E, this option is correct.
- Option B: This option has a negative sign, which is incorrect.
- Option C: It states that the wave is circularly polarized, which is incorrect because the electric field oscillates in a plane.
- Option D: This option states that the electromagnetic field is not plane polarized, which is also incorrect.
Conclusion:
The only correct option is Option A. The wave is indeed plane polarized as it has electric field components in the x and y directions while propagating in the z direction.
The electric field vector is given by:
→E=(E1^i+E2^j)cos(kz−ωt)
This indicates that the electric field has components in both the x-direction (i) and the y-direction (j).
The wave is traveling in the z-direction, as indicated by the argument of the cosine function, which contains kz−ωt. This means the wave propagates along the z-axis.
According to electromagnetic wave theory, the relationship between the electric field →E and the magnetic field →B in a vacuum is given by:
→B=1c→E
where c is the speed of light. Thus, we can express the magnetic field vector as:
→B=1c(E1^i+E2^j)cos(kz−ωt)
The electric field vector has components in the x and y directions, which means it is oscillating in a plane. This indicates that the wave is plane-polarized.
- Option A: Since →B=1c→E, this option is correct.
- Option B: This option has a negative sign, which is incorrect.
- Option C: It states that the wave is circularly polarized, which is incorrect because the electric field oscillates in a plane.
- Option D: This option states that the electromagnetic field is not plane polarized, which is also incorrect.
The only correct option is Option A. The wave is indeed plane polarized as it has electric field components in the x and y directions while propagating in the z direction.
Topper's Solved these Questions
- JEE MAIN REVISION TEST- 24 BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST- 24 EXERCISE - PHYSICS ( SECTION - 2)
5 Videos
- JEE MAIN REVISION TEST- 16BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST- 16EXERCISE - PHYSICS (SECTION 2)
5 Videos
- JEE Main Revision Test-20 | JEE-2020 BOOK - VMC MODULES ENGLISHCHAPTER - JEE Main Revision Test-20 | JEE-2020 EXERCISE - PHYSICS
25 Videos
Similar Questions
Knowledge Check
An electromagnetic wave travelling along z-axis is given as E=E0 cos (kz−ωt). Choose the correct options from the following
AThe associated magnetic field is given as →B=1cˆk×→E=1ω(→k×→E)BThe electromagnetic field can be written in terms of the associated magnetic field as →E=c(→B׈j)Cˆk.→E=0,ˆk.→B≠0Dˆk.→E=0,ˆk×→B=0A plane EM wave travelling along z direction is described by
E=E0sin(kz−ωt)ˆiandB=B0sin(kz−ωt)ˆj.
show that
(i) The average energy density of the wave is given by uav=14ε0E20+14B20μ0.
(ii) The time averaged intensity of the wave is given by Iav=12cε0E20.AThe average energy density of the wave is given by uav=14ε0E20+14B20μ0BThe time averaged intensity of the wave is given by Iav=12cε0E20CBoth (a) and (b)DNone of these
As the e.m. waves travel in free space
View SolutionIdentify A to E choose the correct option accordingly .
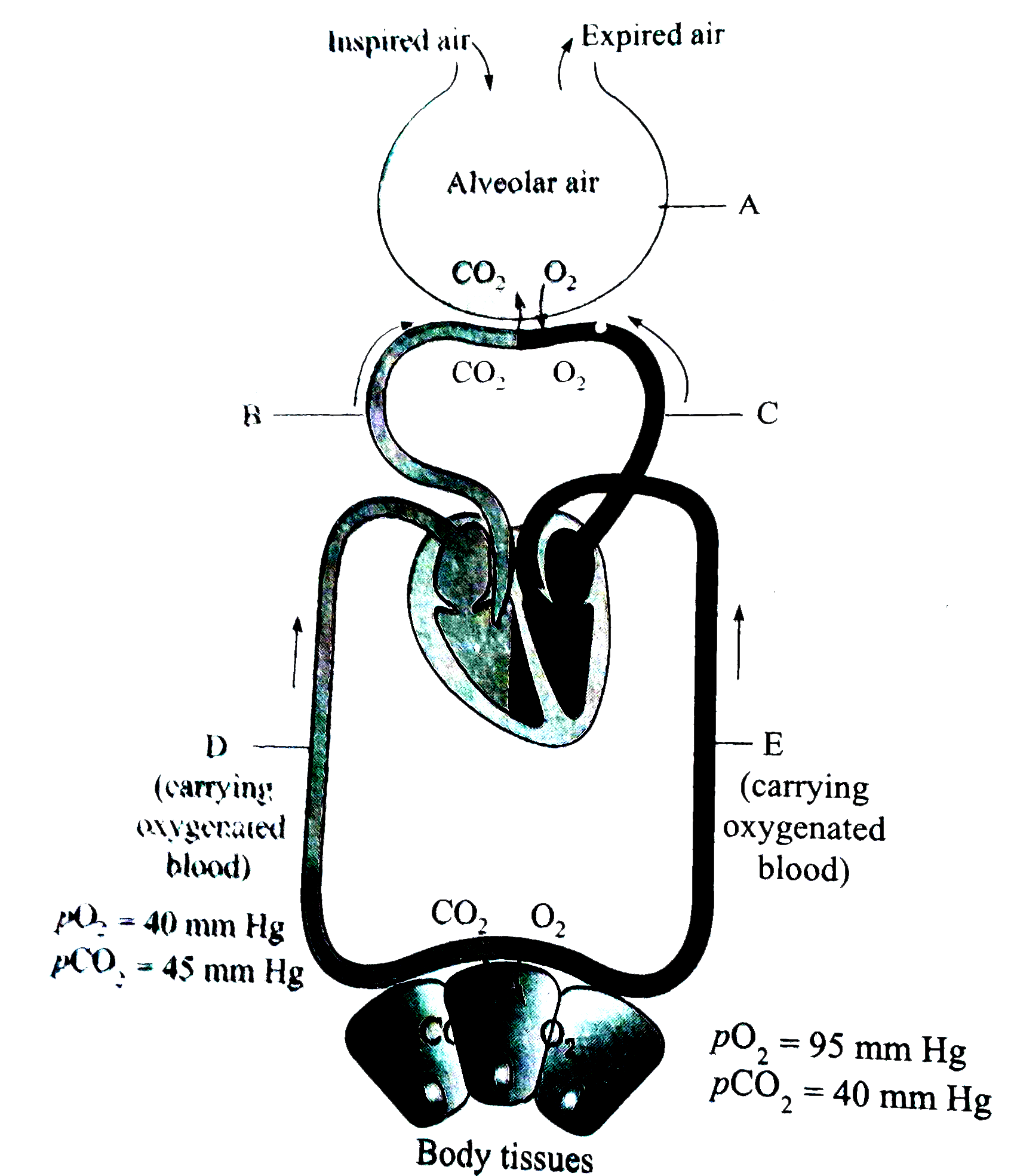
View SolutionA plane electromagnetic wave travels in vacuum along z- direction. What can you say about the directions of ots electric and magnetic field vectors. If the frequency of the wave is 30MHz, what is its wavelength?
View SolutionA plane electromagnetic wave travels in vacuum along z- direction. What can you say about the directions of ots electric and magnetic field vectors. If the frequency of the wave is 30MHz, what is its wavelength?
View SolutionA plane electromagnetic wave travels in vacuum along z-direction. What can you say about the direction of electric and magnetic field vectors?
View SolutionA plane EM wave travelling along z direction is described by
E=E0sin(kz−ωt)ˆiandB=B0sin(kz−ωt)ˆj.
show that
(i) The average energy density of the wave is given by uav=14ε0E20+14B20μ0.
(ii) The time averaged intensity of the wave is given by Iav=12cε0E20.
View Solution
