For emission line of atomic hydrogen from to the plot of wave number against will be (The Rydberg constant, is in wave number unit)
Linear with slope
Non linear
Linear with slope
Linear with intercept
The correct Answer is:A
To solve the problem, we need to analyze the emission lines of atomic hydrogen as it transitions from an initial energy level ni=8 to a final energy level nf=n. We will derive the relationship between the wave number ¯v and 1n2.
Step 1: Understand the formula for wave number
The wave number ¯v is related to the Rydberg formula for hydrogen:
¯v=RH⎛⎝1n2i−1n2f⎞⎠
where RH is the Rydberg constant.
Step 2: Substitute the initial and final states
Given that ni=8 and nf=n, we can substitute these values into the formula:
¯v=RH(182−1n2)
Calculating 82:
82=64
Thus, we have:
¯v=RH(164−1n2)
Step 3: Rearranging the equation
We can express this equation in terms of 1n2:
¯v=RH(164)−RH(1n2)
This can be rewritten as:
¯v=−RH(1n2)+RH(164)
Step 4: Identify the linear relationship
This equation is in the form of y=mx+c, where:
- y is ¯v
- m (the slope) is −RH
- x is 1n2
- c (the y-intercept) is RH(164)
Step 5: Conclusion about the plot
Since the relationship between ¯v and 1n2 is linear, we conclude that the plot of wave number ¯v against 1n2 will be a straight line with a negative slope.
Final Answer
The plot of wave number ¯v against 1n2 will be linear with a negative slope.
---
The wave number ¯v is related to the Rydberg formula for hydrogen:
¯v=RH⎛⎝1n2i−1n2f⎞⎠
where RH is the Rydberg constant.
Given that ni=8 and nf=n, we can substitute these values into the formula:
¯v=RH(182−1n2)
Calculating 82:
82=64
Thus, we have:
¯v=RH(164−1n2)
We can express this equation in terms of 1n2:
¯v=RH(164)−RH(1n2)
This can be rewritten as:
¯v=−RH(1n2)+RH(164)
This equation is in the form of y=mx+c, where:
- y is ¯v
- m (the slope) is −RH
- x is 1n2
- c (the y-intercept) is RH(164)
Since the relationship between ¯v and 1n2 is linear, we conclude that the plot of wave number ¯v against 1n2 will be a straight line with a negative slope.
The plot of wave number ¯v against 1n2 will be linear with a negative slope.
Topper's Solved these Questions
- ATOMIC STRUCTUREBook:VMC MODULES ENGLISHChapter:ATOMIC STRUCTUREExercise:JEE Advanced (Archive )
Explore 67 Videos
- ATOMIC STRUCTUREBook:VMC MODULES ENGLISHChapter:ATOMIC STRUCTUREExercise:LEVEL - 2 ( Numerical value type for JEE Main )
Explore 16 Videos
- AMINESBook:VMC MODULES ENGLISHChapter:AMINESExercise:IN CHAPTER EXERCISE H
Explore 10 Videos
- CHEMICAL BONDING & CHEMICAL STRUCTURE Book:VMC MODULES ENGLISHChapter:CHEMICAL BONDING & CHEMICAL STRUCTURE Exercise:IMPECCABLE
Explore 50 Videos
Similar Questions
For balmer series in the spectrum of atomic hydrogen the wave number of each line is given by ¯¯¯V=R[1n21−1n22] where RH is a constant and n1 and n2 are integers. Which of the following statements (s), is (are correct)
1. As wave length decreases the lines in the series converge
2. The integer n1 is equal to 2.
3. The ionisation energy of hydrogen can be calculated from the wave numbers of three lines.
4. The line of shortest wavelength corresponds to = 3.
View SolutionWhen a transition of electron in He+ takes place from n2 to n1 then wave number in terms of Rydberg constant R will be
(Given n1+n2=4,n2−n1=2)
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
The energy in Joule of an electron in the second orbit of H- atom is:
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
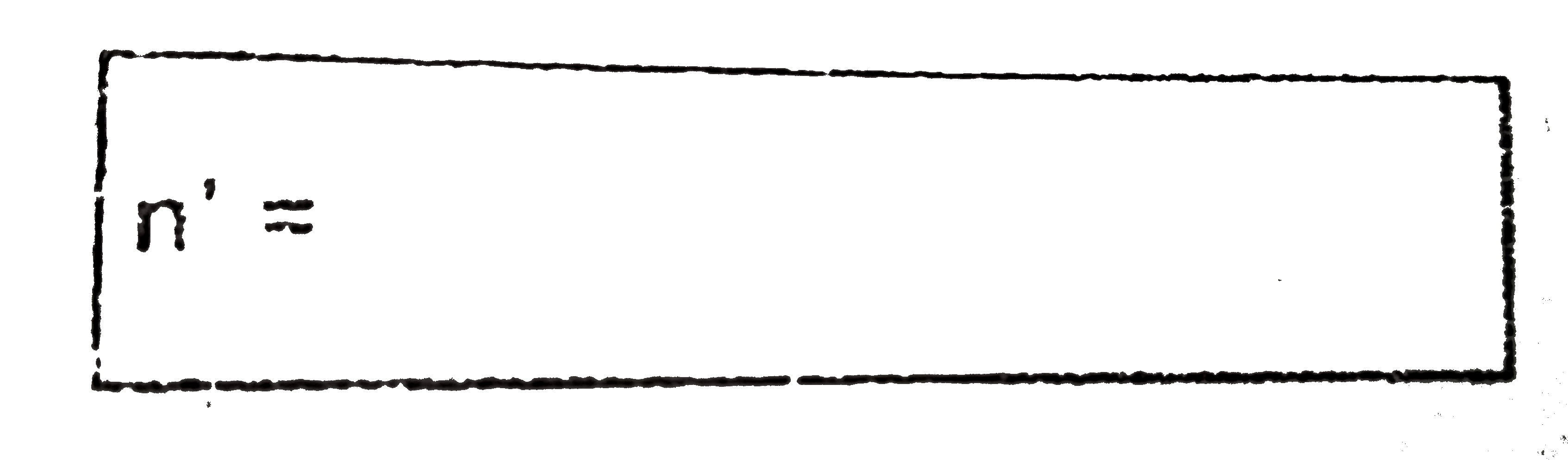
What is the principal quantum number, n' of the orbit of Be3 that has the same Bohr radius as that of ground state hydrogen atom ?
View SolutionThe shortest and longest wave number respectively in H spectrum of Lyman series is : (R = Rydberg constant)
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
Calculate the wavelength of light (nm) for the electronic transition of H-atom from the first excited state to ground state.
In the model of hydrogen like atom put forward by Niels Bohr (1913) the electron orbits around the central nucleus. the bohr radius of nth orbit of a hydrogen-like species is given by
r=kn2Z " " where, k is constant
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
Calculate the following :
(a) the kinetic energy (in eV) of an electron in the ground state of hydrogen atom.
(b) the potential energy (in eV) of an electron in the ground state of hydrogen atom.
View SolutionAn electron makes a transition from orbit n=4 to the orbit n=2 of a hydrogen atom. The wave number of the emitted radiations (R= Rydberg's constant) will be
View SolutionIf in Hydrogen atom, an electron jumps from n2=2 to n1=1 in Bohr's orbit, then the value of wave number of the emitted photon will be (R=109700cm−1)
View SolutionIn excited H atom when electron drop from n=4,5,6 to n=1, there is emission of
View Solution