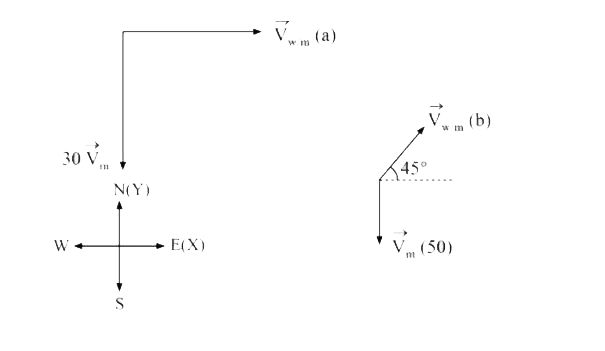
If particles A and B are moving with velocities →VA and →VB , respectively. The relative velocity of A with respect to B is defined as →VAB=→VAB=→VA−→VB . The driver of a car travelling Southward at 30kmh−1 observes that wind appears to be coming from the West. The driver of another car travelling Southward at 50kmh−1 observes that wind appears to be coming from the South-West.
Wind is blowing in a direction tan−1(32) South to East
Wind is blowing in a direction tan−1(32) North to West
The speed of wind is 5√3kmh−1
The speed of wind is 10√13kmh−1
The correct Answer is:ad
→vw=→vvm+→vm
→vmx=20
vwy=−30
tanα=3020=32
vw=√202+302=10√13
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Main ( ARCHIVE ) ( LEVEL-1)12videos
INTRODUCTION TO VECTORS & FORCES
- BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)12videos
INTRODUCTION TO VECTORS & FORCES
- BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 175videos
INTRODUCTION TO VECTORS & FORCES
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE1videos
GRAVITATION
- BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART I : PHYSICS (SECTION - 2)5videos
JEE MAIN - 5
Similar Questions
what is the angle between (→P+→Q) and (→P+→Q)?
View SolutionProjection of →P on →Q is :
View SolutionAre the magnitude and direction of →A−→B same as that →B−→A?
View SolutionLet →C=→A+→B then :
View SolutionIf →B=n→A and →A is antiparallel with →B, then n is :
View SolutionThe angle between vector (→A×→B) and (→B×→A) is :
View SolutionThe angle between vector (→A×→B) and (→B×→A) is :
View SolutionIf ∣∣→A−→B∣∣=∣∣→A∣∣−∣∣→B∣∣ the angle between →A and →B is
View SolutionThe end product of the reaction,
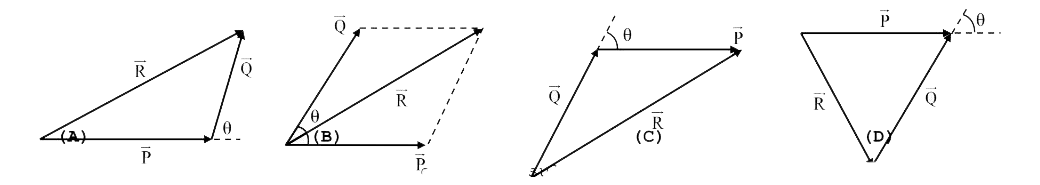
Ethyl amine HNO2−−−→(A)PCl5−−→(B)KCN−−→(C) (C) is,View SolutionWhich of the following representation is incorrect for vector equation →R=→P+→Q
 View Solution
View Solution
