Let →C=→A+→B then :
∣∣→C∣∣ is always greater then ∣∣→A∣∣
it is possible to have and ∣∣→C∣∣<∣∣→B∣∣
∣∣→C∣∣ is always equal to ∣∣→A+→B∣∣
∣∣→C∣∣ is never equal to ∣∣→A+→B∣∣
The correct Answer is:bc
To solve the problem, we need to analyze the vector equation given:
\overset{\rarr}{C} = \overset{\rarr}{A} + \overset{\rarr}{B}
We will evaluate the options based on vector properties.
Step 1: Understanding the vector addition
The equation states that vector \overset{\rarr}{C} is the resultant of vectors \overset{\rarr}{A} and \overset{\rarr}{B} . According to the triangle law of vector addition, the magnitude of the resultant vector C can be expressed in terms of the magnitudes of A and B.
Step 2: Applying the triangle inequality
From the triangle inequality, we know that:
|\overset{\rarr}{A}| + |\overset{\rarr}{B}| > |\overset{\rarr}{C}|
This implies that the sum of the magnitudes of vectors A and B is always greater than the magnitude of vector C.
Step 3: Analyzing the options
1. Option A: |\overset{\rarr}{C}| is always greater than |\overset{\rarr}{A}| .
- This is not necessarily true, as |\overset{\rarr}{C}| can be less than |\overset{\rarr}{A}| if \overset{\rarr}{B} is in the opposite direction of \overset{\rarr}{A} .
2. Option B: |\overset{\rarr}{C}| can be less than |\overset{\rarr}{B}| .
- This is possible if \overset{\rarr}{A} is in the opposite direction to \overset{\rarr}{B} and has a greater magnitude. Thus, this option can be correct.
3. Option C: |\overset{\rarr}{C}| is always equal to |\overset{\rarr}{A}| + |\overset{\rarr}{B}| .
- This is incorrect because equality holds only when \overset{\rarr}{A} and \overset{\rarr}{B} are in the same direction.
4. Option D: |\overset{\rarr}{C}| is never equal to |\overset{\rarr}{A}| + |\overset{\rarr}{B}| .
- This is also incorrect because |\overset{\rarr}{C}| can equal |\overset{\rarr}{A}| + |\overset{\rarr}{B}| when both vectors are in the same direction.
Conclusion
The correct option is Option B: |\overset{\rarr}{C}| can be less than |\overset{\rarr}{B}| .
\overset{\rarr}{C} = \overset{\rarr}{A} + \overset{\rarr}{B}
The equation states that vector \overset{\rarr}{C} is the resultant of vectors \overset{\rarr}{A} and \overset{\rarr}{B} . According to the triangle law of vector addition, the magnitude of the resultant vector C can be expressed in terms of the magnitudes of A and B.
From the triangle inequality, we know that:
|\overset{\rarr}{A}| + |\overset{\rarr}{B}| > |\overset{\rarr}{C}|
This implies that the sum of the magnitudes of vectors A and B is always greater than the magnitude of vector C.
1. Option A: |\overset{\rarr}{C}| is always greater than |\overset{\rarr}{A}| .
- This is not necessarily true, as |\overset{\rarr}{C}| can be less than |\overset{\rarr}{A}| if \overset{\rarr}{B} is in the opposite direction of \overset{\rarr}{A} .
- This is possible if \overset{\rarr}{A} is in the opposite direction to \overset{\rarr}{B} and has a greater magnitude. Thus, this option can be correct.
- This is incorrect because equality holds only when \overset{\rarr}{A} and \overset{\rarr}{B} are in the same direction.
- This is also incorrect because |\overset{\rarr}{C}| can equal |\overset{\rarr}{A}| + |\overset{\rarr}{B}| when both vectors are in the same direction.
The correct option is Option B: |\overset{\rarr}{C}| can be less than |\overset{\rarr}{B}| .
Topper's Solved these Questions
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Main ( ARCHIVE ) ( LEVEL-1)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 1
75 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Video
- JEE MAIN - 5BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART I : PHYSICS (SECTION - 2)
5 Videos
Similar Questions
Dot product of two vectors →A and →B is defined as →A.→B=aBcosϕ , where ϕ is angle between them when they are drawn with tails coinciding. For any two vectors . This means →A.→B=→B.→A that . The scalar product obeys the commutative law of multiplication, the order of the two vectors does not matter. The vector product of two vectors →A and →B also called the cross product, is denoted by →A×→B . As the name suggests, the vector product is itself a vector. →C=→A×→B then C=ABsinθ ,
→A=ˆi+ˆj−ˆk and →B=2ˆi+3ˆj+5ˆk angle between →A and →B is
View SolutionIf ∣∣→A−→B∣∣=∣∣→A∣∣−∣∣→B∣∣ the angle between →A and →B is
View SolutionThe maqunitudes of vecotr →A,→B and →C are respectively 12,5 and 13 units and →A+→B=→C then the angle between →A and →B is :
View SolutionAt what angle the two force →A+→B and →A−→B act so that their resultant is √3A2+B2 ?
View SolutionDot product of two vectors →A and →B is defined as →A.→B=ABcosϕ , where ϕ is angle between them when they are drawn with tails coinciding. For any two vectors . This means ovsert(→)A.→B=→B.→A that . The scalar product obeys the commutative law of multiplication, the order of the two vectors does not matter. The vector product of two vectors →A and →B also called the cross product, is denoted by →A×→B . As the name suggests, the vector product is itself a vector. →C=→A×→B then C=ABsinθ ,
For non zero vectors →A,→B,→C,∣∣(→A×→B).→C∣∣=∣∣→A∣∣∣∣→B∣∣∣∣→C∣∣ holds if and only if
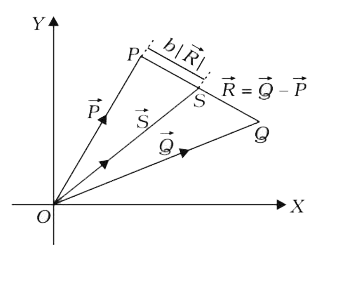
View SolutionThree vectors →P,→Q and →R are shown in the figure. Let S be any point on the vector →R The distance between the point P and S is ∣∣→R∣∣ . The general relation among vectors →P,→Q and →S is :
View SolutionDot product of two vectors →A and →B is defined as →A.→B=aBcosϕ , where ϕ is angle between them when they are drawn with tails coinciding. For any two vectors . This means →A.→B=→B.→A that . The scalar product obeys the commutative law of multiplication, the order of the two vectors does not matter. The vector product of two vectors →A and →B also called the cross product, is denoted by →A×→B . As the name suggests, the vector product is itself a vector. →C=→A×→B then C=ABsinθ ,
A force →F=3ˆi+cˆj+2ˆk acting on a particle causes a displacement d=4ˆi−2ˆj+3ˆk . If the work done (dot product of force and displacement) is 6J then the value of c is :
View SolutionIf ∣∣→A+→B∣∣=∣∣→A−→B∣∣ what is the angle between →A and →B ?
View SolutionThe angle between vector (→A×→B) and (→B×→A) is :
View SolutionThe angle between vector (→A×→B) and (→B×→A) is :
View Solution
