A block rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.8. If the frictional force on the block is 10N, the mass of the block (in kg) is
1.6
4.0
2.0
2.5
The correct Answer is:c
To solve the problem step by step, we will analyze the forces acting on the block resting on the inclined plane.
Step 1: Identify the Forces Acting on the Block
The block experiences two main forces:
1. The gravitational force acting downwards, which can be expressed as mg (where m is the mass of the block and g is the acceleration due to gravity, approximately 10m/s2).
2. The frictional force acting parallel to the incline, which is given as 10N.
Step 2: Resolve the Gravitational Force into Components
The gravitational force can be resolved into two components:
- Perpendicular to the incline: mgcosθ
- Parallel to the incline: mgsinθ
Given that the angle θ=30∘:
- The component of gravitational force parallel to the incline is mgsin(30∘).
- The component of gravitational force perpendicular to the incline is mgcos(30∘).
Step 3: Use the Given Frictional Force
According to the problem, the frictional force f is equal to 10N. The frictional force can be expressed in terms of the normal force N and the coefficient of static friction μ:
f=μN
Where μ=0.8.
Step 4: Calculate the Normal Force
The normal force N is equal to the component of the gravitational force perpendicular to the incline:
N=mgcos(30∘)
Substituting this into the frictional force equation:
10=0.8(mgcos(30∘))
Step 5: Calculate mgsin(30∘)
From the equilibrium condition, the frictional force must balance the component of the gravitational force acting down the incline:
mgsin(30∘)=10
Since sin(30∘)=12:
mg⋅12=10
Step 6: Solve for Mass m
Rearranging the equation gives:
mg=10⋅2=20
Now substituting g=10m/s2:
m⋅10=20
m=2010=2kg
Final Answer
The mass of the block is 2kg.
---
The block experiences two main forces:
1. The gravitational force acting downwards, which can be expressed as mg (where m is the mass of the block and g is the acceleration due to gravity, approximately 10m/s2).
2. The frictional force acting parallel to the incline, which is given as 10N.
The gravitational force can be resolved into two components:
- Perpendicular to the incline: mgcosθ
- Parallel to the incline: mgsinθ
- The component of gravitational force parallel to the incline is mgsin(30∘).
- The component of gravitational force perpendicular to the incline is mgcos(30∘).
According to the problem, the frictional force f is equal to 10N. The frictional force can be expressed in terms of the normal force N and the coefficient of static friction μ:
f=μN
Where μ=0.8.
The normal force N is equal to the component of the gravitational force perpendicular to the incline:
N=mgcos(30∘)
Substituting this into the frictional force equation:
10=0.8(mgcos(30∘))
From the equilibrium condition, the frictional force must balance the component of the gravitational force acting down the incline:
mgsin(30∘)=10
Since sin(30∘)=12:
mg⋅12=10
Rearranging the equation gives:
mg=10⋅2=20
Now substituting g=10m/s2:
m⋅10=20
m=2010=2kg
The mass of the block is 2kg.
Topper's Solved these Questions
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 2
65 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Videos
- JEE MAIN - 5BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART I : PHYSICS (SECTION - 2)
5 Videos
Similar Questions
A block rests on a rough inclined plane making an angle of 30∘ with horizontal. The coefficient of static friction between the block and inclined plane is 0.8 . If the frictional force on the block is 10N, the mass of the block in kg is (g=10m/s2)
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View Solution
Knowledge Check
A block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A10.3NB23.8NC11.9ND6.3NA block of mass 3 kg rests on a rough inclined plane making an angle of 30∘. With the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A9.8 NB0.7×9.8×√3 NC9.8×√3 ND0.7×9.8 N
A block of mass m slides down an inclined plane which makes an angle θ with the horizontal. The coefficient of friction between the block and the plane is μ. The force exerted by the block on the plane is
View SolutionA block of mass 2 kg rests on a rough inclined plane making an angle of 30° with the horizontal. If μS=0.6 , what is the frictional force on the block ?
View SolutionA block is lying on an inclined plane which makes an angle of 60∘ with the horizontal. If coefficient of friction between the block and the plane is 0.25 and g = 10 ms−2, the acceleration of block when it is moves along the plane will be
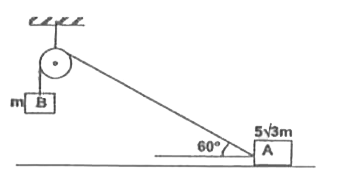
View SolutionA block A of mass 5√3m rests on a rough horizontal plane relative to which its coefficient of friction is μA light string attached to this block passes over a light frictionless pulley and another block B of mass m hangs from it. It is observed that the block A just slips when the string makes an angle of 60∘ with the horizontal .
Find the coefficient of static friction between the block A and the plane. If the same force acting through the sting is applied to the block A in the horizontal direction, find the acceleration of the block A.
View SolutionA block of mass m is placed on a rough plane inclined at an angle θ with the horizontal. The coefficient of friction between the block and inclined plane is μ . Ifθ<tan−1(μ) , then net contact force exerted by the plane on the block is :
View SolutionA block slides down an inclined plane that makes an angle θ with the horizontal. If the coefficient of kinetic friction is μ, find the acceleration of the block.
View Solution
