A piece of wire of length 4L is bent at random, to form a rectangle. The probability that its area is at most L24 is :
1/4
√32
2−√32
4−√3
The correct Answer is:C
To solve the problem, we need to determine the probability that the area of a rectangle formed by bending a wire of length 4L is at most L24.
1. Understanding the Problem:
- The wire is bent to form a rectangle with length x and breadth y.
- The perimeter of the rectangle is given by 2(x+y)=4L, which simplifies to x+y=2L.
2. Expressing Breadth in Terms of Length:
- From x+y=2L, we can express y as:
y=2L−x
3. Calculating the Area of the Rectangle:
- The area A of the rectangle is given by:
A=x⋅y=x(2L−x)=2Lx−x2
4. Setting Up the Inequality:
- We want to find the values of x for which the area is at most L24:
2Lx−x2≤L24
5. Rearranging the Inequality:
- Rearranging gives:
x2−2Lx+L24≥0
6. Factoring the Quadratic:
- This can be rewritten as:
(x−L)2−3L24≥0
- This is a difference of squares:
(x−L−√32L)(x−L+√32L)≥0
7. Finding the Critical Points:
- The critical points are:
x=L−√32Landx=L+√32L
- Simplifying gives:
x=L(1−√32)andx=L(1+√32)
8. Identifying the Valid Range for x:
- The valid range for x is:
0≤x≤2L
- The area condition gives two intervals:
x∈(0,L(1−√32))∪(L(1+√32),2L)
9. Calculating the Length of the Intervals:
- The length of the first interval:
L(1−√32)−0=L(1−√32)
- The length of the second interval:
2L−L(1+√32)=L(2−1−√32)=L(1−√32)
- Total length of valid intervals:
2L(1−√32)
10. Finding the Total Length of Possible Values for x:
- The total length for x is:
2L
11. Calculating the Probability:
- The probability P that the area is at most L24 is given by:
P=Length of valid intervalsTotal length=2L(1−√32)2L=1−√32
Final Answer:
Thus, the probability that the area of the rectangle is at most L24 is:
1−√32
- The wire is bent to form a rectangle with length x and breadth y.
- The perimeter of the rectangle is given by 2(x+y)=4L, which simplifies to x+y=2L.
- From x+y=2L, we can express y as:
y=2L−x
- The area A of the rectangle is given by:
A=x⋅y=x(2L−x)=2Lx−x2
- We want to find the values of x for which the area is at most L24:
2Lx−x2≤L24
- Rearranging gives:
x2−2Lx+L24≥0
- This can be rewritten as:
(x−L)2−3L24≥0
- This is a difference of squares:
(x−L−√32L)(x−L+√32L)≥0
- The critical points are:
x=L−√32Landx=L+√32L
- Simplifying gives:
x=L(1−√32)andx=L(1+√32)
- The valid range for x is:
0≤x≤2L
- The area condition gives two intervals:
x∈(0,L(1−√32))∪(L(1+√32),2L)
- The length of the first interval:
L(1−√32)−0=L(1−√32)
- The length of the second interval:
2L−L(1+√32)=L(2−1−√32)=L(1−√32)
- Total length of valid intervals:
2L(1−√32)
- The total length for x is:
2L
- The probability P that the area is at most L24 is given by:
P=Length of valid intervalsTotal length=2L(1−√32)2L=1−√32
Thus, the probability that the area of the rectangle is at most L24 is:
1−√32
Topper's Solved these Questions
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - NUMERICAL VALUE TYPE FOR JEE MAIN
15 Videos
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - JEE MAIN (ARCHIVE)
37 Videos
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - LEVEL - 1
95 Videos
- PERMUTATION & COMBINATIONBOOK - VMC MODULES ENGLISHCHAPTER - PERMUTATION & COMBINATIONEXERCISE - JEE ARCHIVE
50 Videos
- PROPERTIES OF TRIANGLEBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF TRIANGLEEXERCISE - JEE Advanced (Archive)
50 Videos
Similar Questions
A wire of length I is cut into three pleces. What Is the probability that the three pieces form a triangle ?
View SolutionA piece of wire of length 108 cm is bent to form a semicircular arc bounded by its diameter. Find its radius and enclosed area.
View SolutionA wire of resistance 4Ω is bent to form a circle. The resistance point A and B will be
_PHY_E05_303_Q01.png)
View SolutionChoose the correct option:
A wire of mass m and length l is bent in the form of a quarter circle. The moment of the inertia of the wire about an axis is passing through the centre of the quarter circle is approximately
View SolutionA thin uniform wire of mass m and length l is bent into to shape of a semicircle. The moment of inertia of that wire about an axis passing through its free ends is
View SolutionA wire of length l and mass m is bent in the form of a semicircle. The gravitational field intensity at the centre of semicircle is

View SolutionA uniform wire of length l is bent into the shape of 'V' as shown. The distance of its centre of mass from the vertex A is :-
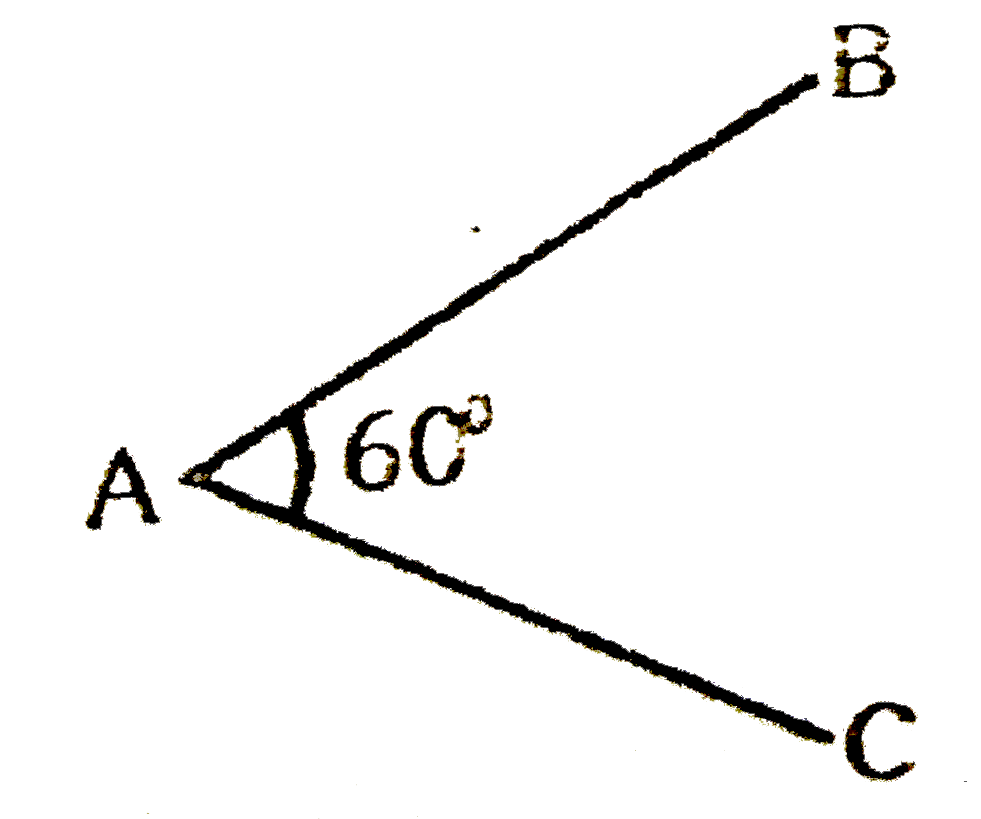
View SolutionA wire is in the form of a square of side 18 m. It is bent in the form of a rectangle, whose length and breadth are in the ratio 3:1. The area of the rectangle is
(a) 81 m2
(b) 243 m2
(c) 144 m2
(d) 324 m2
View SolutionDeepak took a wire of length 176 cm and bent it into the shape of a circle. Find the radius of that circle. Also, tind its area. IF the sam wire is bent into the shape of a rectangle of length 7 cm, what will be the breadth of the rectangle ? Which encloses more area, the circle or the rectangle ?
View SolutionA wire of length l is used to form a coil. The magnetic field at its centre for a given current in it is minimum if the coil has
View Solution
