A certain mass of an ideal gas is at pressure and volume . If is compressed isothermally and then allowed to expand adiabatically untill its pressure returns to . The gas is then allowed to expand its original volume. Which of the following graphs are these process correctly shown?
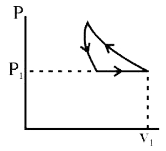

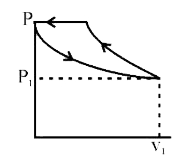
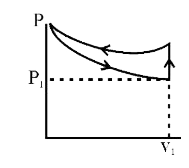
The correct Answer is:A
To solve the problem, we need to analyze the processes involved in the transformation of the ideal gas and how they are represented in a P-V graph.
1. Identify Initial Conditions:
- The gas starts at pressure P1 and volume V1.
2. Isothermal Compression:
- The gas is compressed isothermally. In an isothermal process, the temperature remains constant, and the relationship between pressure and volume is given by Boyle's Law: P1V1=P2V2.
- On a P-V graph, this process will be represented by a hyperbolic curve that slopes downwards to the right, indicating that as volume decreases, pressure increases.
3. Adiabatic Expansion:
- After isothermal compression, the gas undergoes adiabatic expansion until it returns to pressure P1. During an adiabatic process, no heat is exchanged with the surroundings, and the relationship between pressure and volume is given by PVγ=constant, where γ is the heat capacity ratio.
- The P-V graph for this process will also slope downwards but steeper than the isothermal curve, indicating that pressure decreases more rapidly with volume increase.
4. Return to Original Volume:
- Finally, the gas is allowed to expand to its original volume V1 at constant pressure P1. This is an isobaric process, represented by a horizontal line on the P-V graph, as the pressure remains constant while the volume increases.
5. Combining the Processes:
- The complete cycle on the P-V graph will start from point (P1,V1), move downwards along the isothermal curve to a new point (P2,V2), then steeply downwards along the adiabatic curve until it reaches back to pressure P1 at a new volume V3, and finally move horizontally to the right back to the original volume V1 at pressure P1.
6. Identifying the Correct P-V Graph:
- The correct P-V graph will show:
- A downward curve for the isothermal compression.
- A steeper downward curve for the adiabatic expansion.
- A horizontal line returning to the original volume at constant pressure.
Conclusion:
The correct P-V graph will show these three distinct processes clearly, with the isothermal curve having a gentler slope than the adiabatic curve, followed by a horizontal line indicating isobaric expansion.
- The gas starts at pressure P1 and volume V1.
- The gas is compressed isothermally. In an isothermal process, the temperature remains constant, and the relationship between pressure and volume is given by Boyle's Law: P1V1=P2V2.
- On a P-V graph, this process will be represented by a hyperbolic curve that slopes downwards to the right, indicating that as volume decreases, pressure increases.
- After isothermal compression, the gas undergoes adiabatic expansion until it returns to pressure P1. During an adiabatic process, no heat is exchanged with the surroundings, and the relationship between pressure and volume is given by PVγ=constant, where γ is the heat capacity ratio.
- The P-V graph for this process will also slope downwards but steeper than the isothermal curve, indicating that pressure decreases more rapidly with volume increase.
- Finally, the gas is allowed to expand to its original volume V1 at constant pressure P1. This is an isobaric process, represented by a horizontal line on the P-V graph, as the pressure remains constant while the volume increases.
- The complete cycle on the P-V graph will start from point (P1,V1), move downwards along the isothermal curve to a new point (P2,V2), then steeply downwards along the adiabatic curve until it reaches back to pressure P1 at a new volume V3, and finally move horizontally to the right back to the original volume V1 at pressure P1.
- The correct P-V graph will show:
- A downward curve for the isothermal compression.
- A steeper downward curve for the adiabatic expansion.
- A horizontal line returning to the original volume at constant pressure.
The correct P-V graph will show these three distinct processes clearly, with the isothermal curve having a gentler slope than the adiabatic curve, followed by a horizontal line indicating isobaric expansion.
Topper's Solved these Questions
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE MAIN (ARCHIVE )
81 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )
111 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - Level - 1
75 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
1 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Videos
Similar Questions
A monoatomic gas at pressure P1 and volume V1 is compressed adiabatically to 18th of its original volume. What is the final pressure of gas.
View SolutionA monatomic gas at a pressure P, having a volume V expands isothermally to a volume 2 V and then adiabatically to a volume 16 V. The final pressure of the gas is ( take γ=53)
View Solution
Knowledge Check
A mass of ideal gas at pressure P is expanded isothermally to four times the originl volume and then slowly compressed adiabatically to its original volume. Assuming γ to be 1.5 , the new pressure of the gas is
A0.58333333333333BPC0.66666666666667DP/2
An ideal gas having initial pressure P, volume Vand temperature T is allowed to expand adiabatically until its volume becomes 5.66 V while the temperature falls to T/2. How many degrees of freedom do the gas molecule have ?
View SolutionAn ideal gas is initially at P1,V1 is expands to P2,V2 isothermally and then compressed adiabatically to the same volume V1 and pressure P3. If W is the net work done by the gas in complete process which of the following is true.
View SolutionAn ideal gas is initially at P1,V1 is expands isothermally to P2,V2 and then compressed adiabatically to the same volume V1 and pressure P3. If W is the net work done by the gas in complete process which of the following is true.
View Solutionwhen an ideal gas with pressure p and volume V is compressed Isothermally to one - fourth of its volume, is pressure is P1 when the same gas is compressed polytropically according to the equation PV1.5 contents to one - fourth of its initial volume, the pressure is P2 the ratio P1P2 is
View SolutionWhen an ideal gas at pressure P, temperature T and volume V is isothermally compressed to V/n. its pressure becomes Pi. If the gas is compressed adiabatically to V/n, its pressure becomes Pa. The ratio Pi/Pa is: ( l=CP/CV)
View SolutionA sample of an ideal gas has pressure p0, volume V0 and tempreture T0. It is isothermally expanded to twice its oringinal volume.it is then compressed at constant pressure to have the original volume V0. Finally, the gas is heated at constant volume to get the original tempreture.(a) show the process in a V-T diagram (b) calculate the heat absorbed in the process.
View SolutionThe pressure p and volume V of an ideal gas both increase in a process.
View Solution
