Two parallel wires each of length 10 cm are 0.5 cm apart. A film of water is formed between them. If surface tension of water is 0.072 N/m, then the work done in increasing the distance between the wires by 1 mm is:
1.44×10−5J
1.72×10−5J
1.44×10−4J
1.72×10−4J
The correct Answer is:A
To solve the problem step by step, we will calculate the work done in increasing the distance between the two parallel wires by 1 mm, given the surface tension of water.
Step 1: Understand the given data
- Length of each wire (L) = 10 cm = 0.1 m
- Initial distance between the wires (d) = 0.5 cm = 0.005 m
- Increase in distance (Δd) = 1 mm = 0.001 m
- Surface tension of water (T) = 0.072 N/m
Step 2: Calculate the change in area (ΔA)
When the distance between the wires is increased, the area of the water film between them changes. The change in area can be calculated using the formula:
ΔA=L×Δd
Since there are two surfaces (one on each wire), the total change in area will be:
ΔA=2×(L×Δd)
Substituting the values:
ΔA=2×(0.1m×0.001m)=2×0.0001m2=0.0002m2
Step 3: Calculate the work done (W)
The work done in increasing the distance between the wires is given by the formula:
W=T×ΔA
Substituting the values:
W=0.072N/m×0.0002m2
Calculating this gives:
W=0.072×0.0002=0.0000144J
Step 4: Convert the work done to standard form
To express the work done in standard form:
W=1.44×10−5J
Final Answer
The work done in increasing the distance between the wires by 1 mm is:
1.44×10−5J
- Length of each wire (L) = 10 cm = 0.1 m
- Initial distance between the wires (d) = 0.5 cm = 0.005 m
- Increase in distance (Δd) = 1 mm = 0.001 m
- Surface tension of water (T) = 0.072 N/m
When the distance between the wires is increased, the area of the water film between them changes. The change in area can be calculated using the formula:
ΔA=L×Δd
Since there are two surfaces (one on each wire), the total change in area will be:
ΔA=2×(L×Δd)
ΔA=2×(0.1m×0.001m)=2×0.0001m2=0.0002m2
The work done in increasing the distance between the wires is given by the formula:
W=T×ΔA
Substituting the values:
W=0.072N/m×0.0002m2
Calculating this gives:
W=0.072×0.0002=0.0000144J
To express the work done in standard form:
W=1.44×10−5J
The work done in increasing the distance between the wires by 1 mm is:
1.44×10−5J
Topper's Solved these Questions
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL -2
50 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE MAIN (LEVEL - 1 )
31 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL-0 ( Short Answer Type - II )
15 Videos
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE
53 Videos
- MAGNETIC EFFECTS OF CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Advanced (Archive)
78 Videos
Similar Questions
Knowledge Check
If the surface tension of water is 0.06 N/m, then the capillary rise in a tube of diameter 1mm is (θ=0∘)
A1.22 cmB2.44 cmC3.12 cmD3.86 cmThe surface tension of soap solution is 0.03N/m . The work done in blowing to from a soap bublle of surface area 40cm2, (in J) , is
A2.4×10−4 JB1.2×10−2 JC3.6×10−4 JD4.2×10−2 J
A small drop of water kept pressed between two glass plates spreads as a circle of diameter 10cm. If the distance between the plates is 0.005 mm, and surface tension of water is 0.072Nm−1 , what force will be required to separate the plates ?
View SolutionFind the area of a trapezium whose parallel sides are of lengths 10cm and 12cm and the distance between them is 4cm.
View SolutionTwo glass plates are separated by water. If surface tension of water is 75dyn/cm and the area of each plate wetted by water is 8cm2 and the distance between the plates is 0.12mm, then the force applied to separate the two plates is
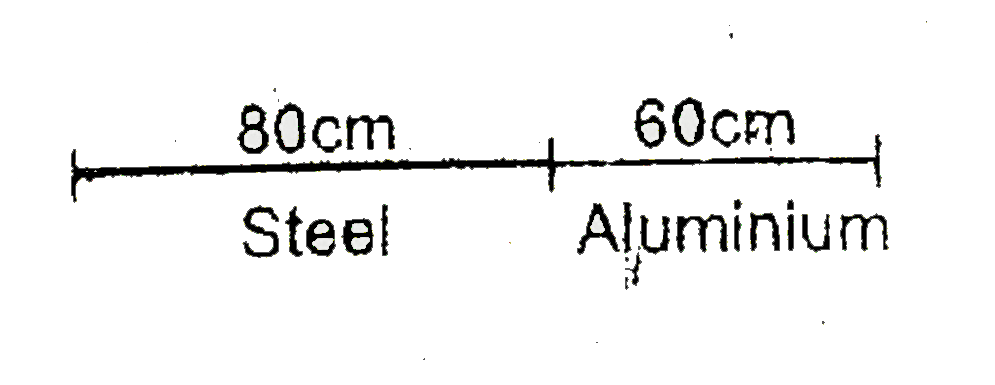
View SolutionFigure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
View SolutionA film of water is formed between two straight parallel wires each 10cm long and at a seperation of 0.5cm. Calculate the work required to increase 1mm distance between the wires. Surface tension of water =72×10−3N/m.
View SolutionA film of water is formed between two straight parallel wires each 10cm long and at a seperation of 0.5cm. Calculate the work required to increase 1mm distance between the wires. Surface tension of water =72×10−3N/m.
View Solution