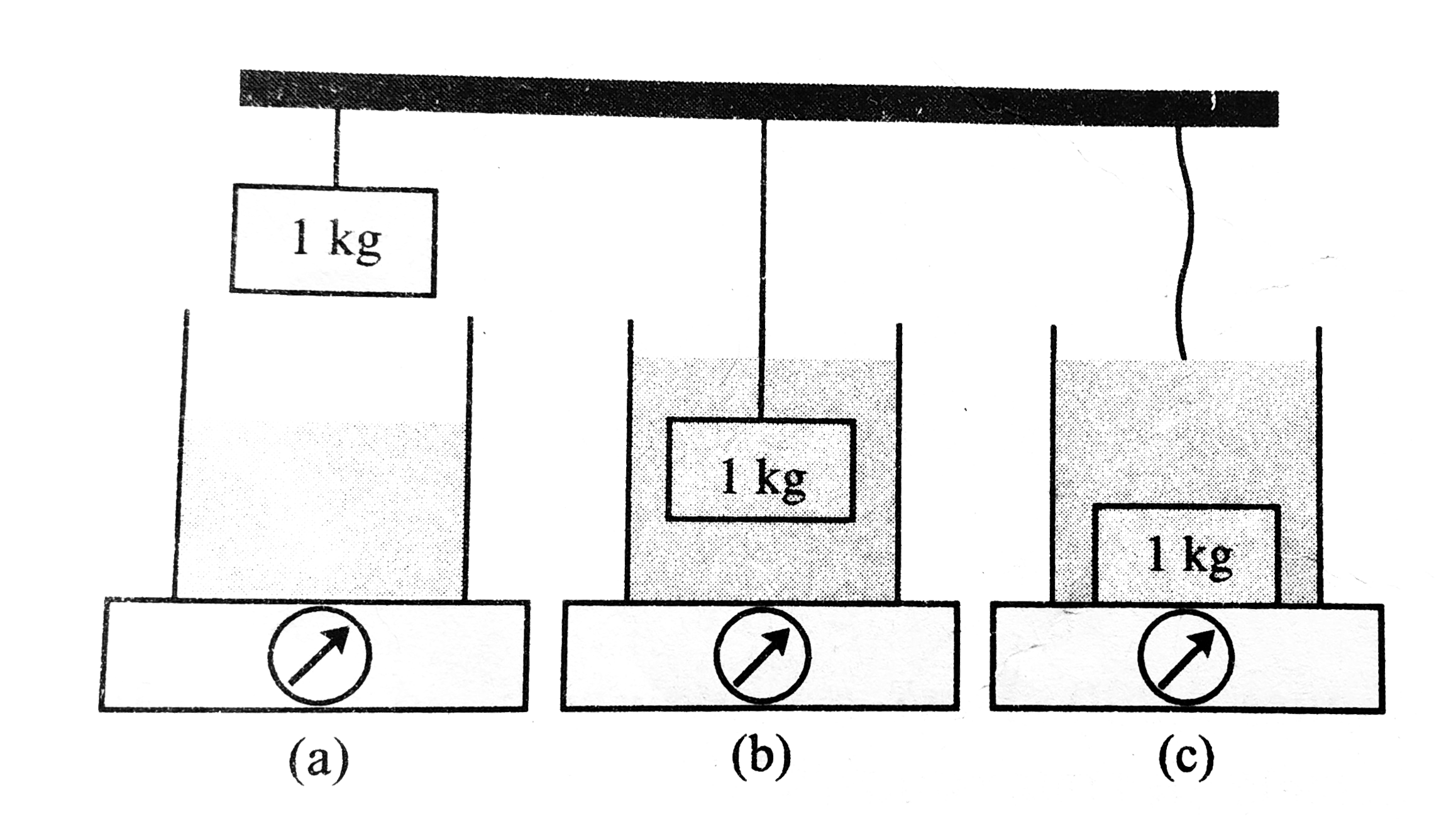
Three identical blocks each of mass m=1kg and volume 3×10−4m3 are suspended by massless strings from a support as shown. Underneath are three identical containers containing the same amount of water that are placed over the scales. In Fig. (a), the block is completely out of the water, in Fig. (b), the block is completely submerged but not touching the beaker and in Fig. (c), the block rests on the bottom of the beaker. The scale in Fig (a) reads 14N.
The tension in the string in (b) is 10 N
The tension in the string in (b) is 7 N
The reading of the scale in (b) is 17 N
The reading of the scale in (c) is 24 N
The correct Answer is:B, C, D
Density of block =13×10−4kg/m3=103×103kg/m3
Buoyant force, B=3×10−4×103×g=3N⇒TB=10−3=7N
Reading of B=14+3=17N. Reading of C=[14+(10−3)+3]N=24N
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE MAIN (LEVEL - 1 )31videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)55videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL -190videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE53videos
LAWS OF MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Advanced (Archive)78videos
MAGNETIC EFFECTS OF CURRENT
Similar Questions
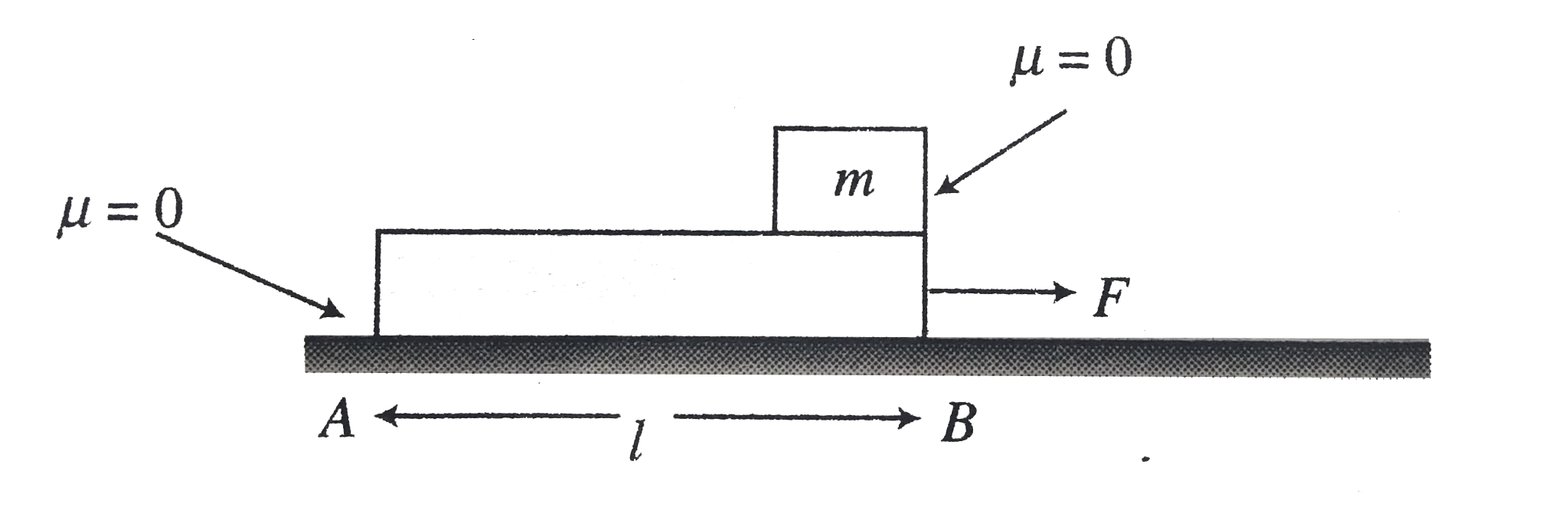
In fig. a small block is kept on m. then
 View Solution
View SolutionA block of mass 4 kg and volume 5×10−4m3 is suspended by a spring balance in a lift which is accelerating. The apparent weight shown by the spring balance is 3 kg. Now the block is immersed in water in kg shown by the spring balance is
View Solution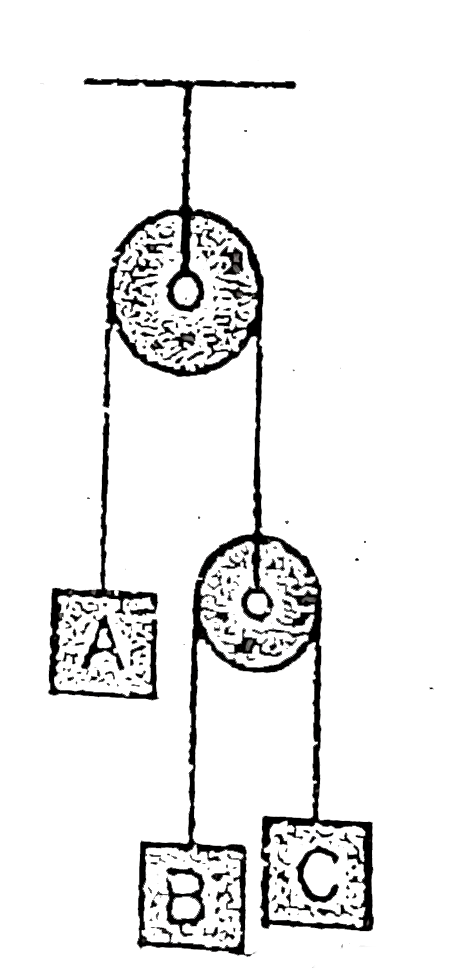
Three blocks A, B and C havig masses m kg, 2kg and 3 kg are attached by massless strings and ideal pulleys as shown in the figure. When the system is realeased from rest if the block A remains stationary. the mass of block A isView SolutionA block of mass 30 kg is suspended by three string as shown in fig, Find the tension in each string.
 View Solution
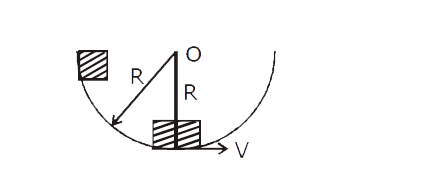
View SolutionA block of mass m slides down along the surface of the bowl from the rim to the bottom as shown in fig. The velocity of the block at the bottom will be-
 View Solution
View SolutionFigure shows a string stretched by a block going over a pulley. The string vibrates in its tenth harmonic in unison with a particular tuning fork. When a beaker containing water is brought under the block so that the block is completely dipped into the beaker, the string vibrates in its eleventh harmonic. Find the density of the material of the block.
 .View Solution
.View SolutionTwo identical blocks A and B of mass m joined together with a massless spring as shown in figure are placed on a smooth surface. If the block A moves with an acceleration a0, then the acceleration of the block B is
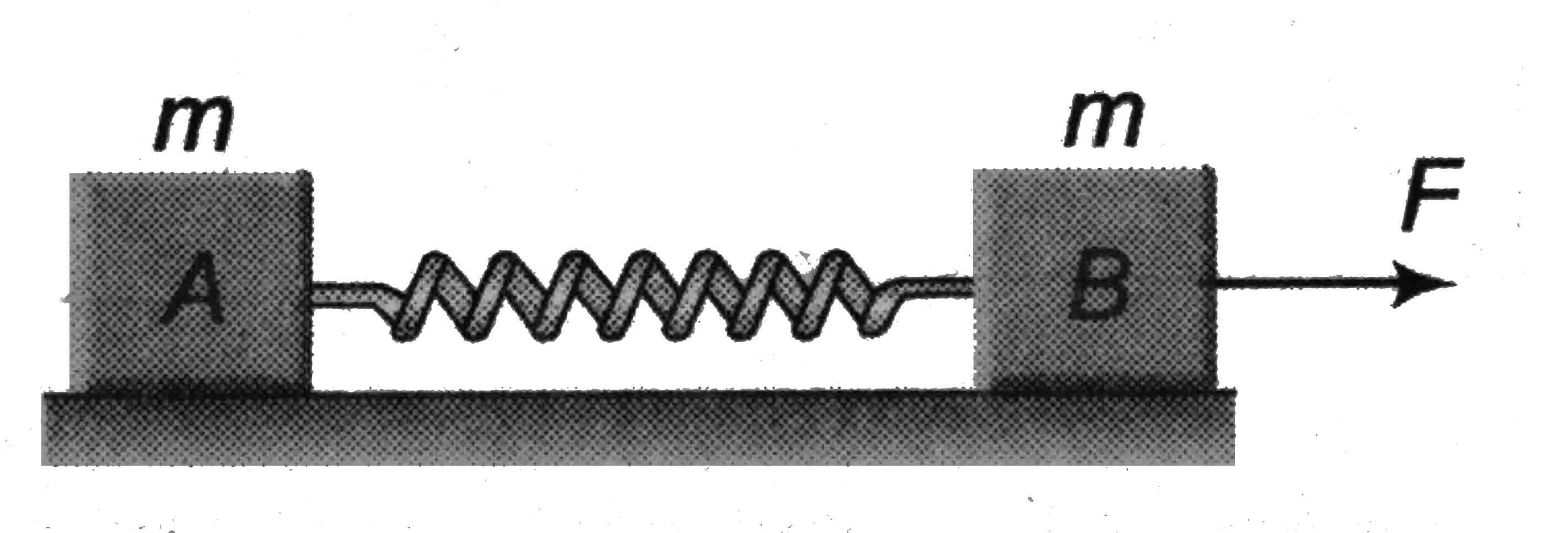 View Solution
View SolutionTwo blocks A and B of same mass m attached with a light spring are suspended by a string as shown in fig. Find the acceleration of block A and B just after the string is cut.
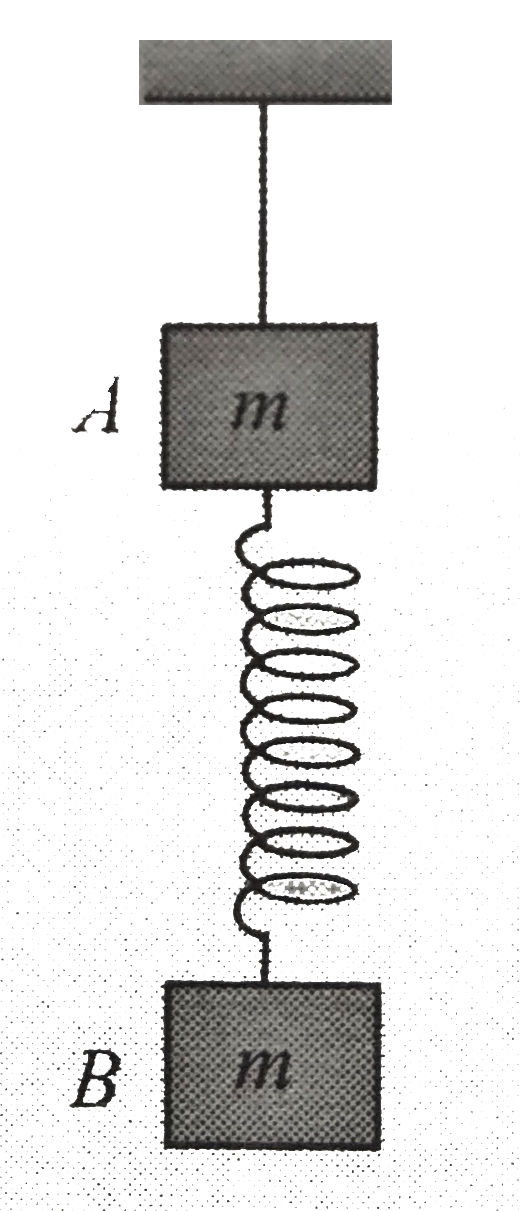 View Solution
View SolutionFind the acceleration of blocks in fig. The pulley and the string are massless.
 View Solution
View SolutionA block of mass m is placed on the inclined sufrace of a wedge as shown in fig. Calculate the acceleration of the wedge and the block when the block is released. Assume all surfaces are frictionless.
 View Solution
View Solution

