There is a small hole in the bottom of a fixed container containing a liquid upto height 'h'. The top of the liquid as well as the hole at the bottom are exposed to atmosphere. As the liquid come out of the hole. (Area of the hole is 'a' and that of the top surface is 'A') :
upwards at g
upwards at g(a2)A2
downwards at g(a)A
downwards at g(a2)A2
The correct Answer is:D
To solve the problem step by step, we will analyze the situation using principles from fluid dynamics, specifically Torricelli's law and the continuity equation.
Step 1: Understand the Setup
We have a container filled with liquid up to a height h. There is a small hole at the bottom of the container, and both the top surface of the liquid and the hole are exposed to atmospheric pressure.
Step 2: Determine the Velocity of the Liquid Exiting the Hole
According to Torricelli's law, the velocity v2 of the liquid flowing out of the hole can be determined using the formula:
v2=√2gh
where g is the acceleration due to gravity and h is the height of the liquid column above the hole.
Step 3: Apply the Continuity Equation
The continuity equation states that the product of the cross-sectional area and the velocity of the fluid must remain constant along a streamline. If A is the area of the top surface of the liquid and a is the area of the hole, we can write:
Av1=av2
where v1 is the velocity of the liquid surface.
Step 4: Express v1 in Terms of v2
From the continuity equation, we can express v1 as:
v1=aAv2
Substituting v2 from Step 2:
v1=aA√2gh
Step 5: Analyze the Change in Height of the Liquid
As the liquid flows out, the height h of the liquid in the container decreases. We need to find the acceleration of the liquid surface, which can be expressed as the change in velocity with respect to the change in height:
a1=dv1dt=dv1dh⋅dhdt
Step 6: Differentiate v1 with Respect to h
To find dv1dh, we differentiate v1:
v1=aA√2gh
Differentiating with respect to h:
dv1dh=aA⋅12√2gh⋅2g=agA√2gh
Step 7: Substitute Back into the Acceleration Equation
Now substituting dv1dh back into the acceleration equation:
a1=agA√2gh⋅dhdt
Since dhdt is negative (as height decreases), we can denote it as:
a1=−agA√2gh⋅dhdt
Step 8: Conclusion
The negative sign indicates that the acceleration of the liquid surface is directed downward, which is consistent with the fact that the height of the liquid is decreasing as it flows out.
Final Result
The acceleration of the liquid surface is given by:
a1=−a2gA2
We have a container filled with liquid up to a height h. There is a small hole at the bottom of the container, and both the top surface of the liquid and the hole are exposed to atmospheric pressure.
According to Torricelli's law, the velocity v2 of the liquid flowing out of the hole can be determined using the formula:
v2=√2gh
where g is the acceleration due to gravity and h is the height of the liquid column above the hole.
The continuity equation states that the product of the cross-sectional area and the velocity of the fluid must remain constant along a streamline. If A is the area of the top surface of the liquid and a is the area of the hole, we can write:
Av1=av2
where v1 is the velocity of the liquid surface.
From the continuity equation, we can express v1 as:
v1=aAv2
Substituting v2 from Step 2:
v1=aA√2gh
As the liquid flows out, the height h of the liquid in the container decreases. We need to find the acceleration of the liquid surface, which can be expressed as the change in velocity with respect to the change in height:
a1=dv1dt=dv1dh⋅dhdt
To find dv1dh, we differentiate v1:
v1=aA√2gh
Differentiating with respect to h:
dv1dh=aA⋅12√2gh⋅2g=agA√2gh
Now substituting dv1dh back into the acceleration equation:
a1=agA√2gh⋅dhdt
Since dhdt is negative (as height decreases), we can denote it as:
a1=−agA√2gh⋅dhdt
The negative sign indicates that the acceleration of the liquid surface is directed downward, which is consistent with the fact that the height of the liquid is decreasing as it flows out.
The acceleration of the liquid surface is given by:
a1=−a2gA2
Topper's Solved these Questions
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE MAIN (LEVEL - 1 )
31 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)
55 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL -1
90 Videos
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE
53 Videos
- MAGNETIC EFFECTS OF CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Advanced (Archive)
78 Videos
Similar Questions
Knowledge Check
A tank filled with fresh water has a hole in its bottom and water is flowing out of it. If the size of the hole is increased, then
Athe volume of water flowing out per second will decreaseBthe velocity of outflow of water remains unchangedCthe volume of water volume out per second remains zeroDBoth (b) and (c)
There are two identical small holes on the opposite sides of a tank containing a liquid. The tank is open at the top. The difference in height between the two holes is h. As the liquid comes out of the two holes. The tank will experience a net horizontal force proportional to.
 .
.
View SolutionA wide vessel with a small hole at the bottom is filled with two liquids. The density and height of one liquid are ρ1 and h1 and that of the other are ρ2 and h2(ρ1>ρ2). The velocity of liquid coming out of the hole is:
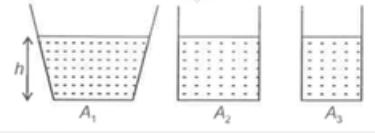
View SolutionThree vessels containing same liquid, upto the same height h, then pressure at the bottom is (A1>A2>A3)
View SolutionA small illuminated bulb is at the bottom of a tank, containing a liquid of refractive index μ upto a height H. Find the expression for the diameter of an opaque disc, floating symmetrically on the liquid surface in order to cut- off the light from the bulb.
View SolutionThere is a hole at the bottom of a large open vessel. If water is filled upto a height h, it flows out in time t. if water is filled to a height 4h, it will flow out in time
View SolutionA beaker containing a liquid is kept inside a big closed jar. If the air inside the jar is continuously pumped out, the pressure in the liquid near the bottom of the liquid will
View Solution
Artificial satellites go round the earth in their respective orbits. Astronauts are able to float in side the satellite. Inside space of the satellite could be treated as gravity free space. If a beaker filled with a liquid upto height h having a small hole in the bottom is placed in such a satellite, you will wonder te liquid does not come out of the hole. You have to squeeze or force the liquid to come out. suppose a constant pressure p is applied on the liquid through piston to force it out through the hole. Out side pressure could be neglected in comparison with p Cross-sectional area of beaker is too greater than that of hole (A>>a)
View Solution
