The points (−a,−b), (0,0). (a,b) and (a2,ab)) are
collinear
vertices of a rectangle
vertices of a parallelogram
None of these
The correct Answer is:A
To determine whether the points (−a,−b), (0,0), (a,b), and (a2,ab) are collinear, we can use the concept of slopes between the points. If the slopes between any two pairs of points are equal, then the points are collinear.
1. Identify the Points:
Let the points be:
- A(−a,−b)
- B(0,0)
- C(a,b)
- D(a2,ab)
2. Calculate the Slope of Line AB:
The slope mAB between points A and B is given by:
mAB=y2−y1x2−x1=0−(−b)0−(−a)=ba
3. Calculate the Slope of Line BC:
The slope mBC between points B and C is given by:
mBC=b−0a−0=ba
4. Calculate the Slope of Line CD:
The slope mCD between points C and D is given by:
m_{CD} = \frac{ab - b}{a^2 - a} = \frac{b(a - 1)}{a(a - 1)} = \frac{b}{a} \quad \text{(assuming a \neq 1 )}
5. Compare the Slopes:
We have:
mAB=ba,mBC=ba,mCD=ba
Since all slopes are equal, we conclude that the points A, B, C, and D are collinear.
Conclusion:
The points (−a,−b), (0,0), (a,b), and (a2,ab) are collinear.
Let the points be:
- A(−a,−b)
- B(0,0)
- C(a,b)
- D(a2,ab)
The slope mAB between points A and B is given by:
mAB=y2−y1x2−x1=0−(−b)0−(−a)=ba
The slope mBC between points B and C is given by:
mBC=b−0a−0=ba
The slope mCD between points C and D is given by:
m_{CD} = \frac{ab - b}{a^2 - a} = \frac{b(a - 1)}{a(a - 1)} = \frac{b}{a} \quad \text{(assuming a \neq 1 )}
We have:
mAB=ba,mBC=ba,mCD=ba
Since all slopes are equal, we conclude that the points A, B, C, and D are collinear.
The points (−a,−b), (0,0), (a,b), and (a2,ab) are collinear.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - VMC MODULES ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - JEE Main Archive (State true or false: Q. 16 to 18)
3 Videos
- STRAIGHT LINESBOOK - VMC MODULES ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - JEE Advanced Archive
42 Videos
- STRAIGHT LINESBOOK - VMC MODULES ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Numerical Value Type for JEE Main
15 Videos
- SEQUENCE AND SERIES BOOK - VMC MODULES ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - JEE MAIN & Advance ( ARCHIVE)
46 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - VMC MODULES ENGLISHCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - JEE ADVANCED (ARCHIVE)
34 Videos
Similar Questions
Knowledge Check
In the rectangular coordinate system shown, ABCD is a parallogram. If the coordinates of the points A,B,C and D are (0,2),(a,b),(a,2) and (0,0), respectively, then b =
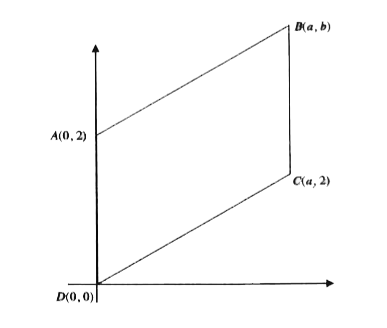 A0B2C3D4
A0B2C3D4The coordinates of the point which is equidistant from the points O(0,0,0) A (a,0,0), B(0,b,0) and C(0,0,c)
A(a,b,c)B(a2,b2,c2)C(a3,b3,c3)D(−a2,−b2,−c2)
If the points A(1,2) , B(0,0) and C (a,b) are collinear , then
View SolutionThe coordinates of the circumcentre of the triangle formed by the points O (0, 0) , A(a, 0) and B(0, b) are (a, b) (b) (a2,b2) (c) (b2,a2) (d) (b, a)
View SolutionIf points (a2,0),(0,b2)and(1,1) are collinear, then
View SolutionFind the product of the following two matrices ⎡⎢⎣0c−bc0ab−a0⎤⎥⎦and⎡⎢⎣a2abacabb2bcacbcc2⎤⎥⎦.
View SolutionThe four points A(α,0),B(β,0),C(γ,0) and D(δ,0) are such that α,β are the roots of equation ax2+2hx+b=0, and γ,δ are the roots of equation a'x2+2h'x+b'=0. Show that the sum of the ratios in which C and D divide AB is zero, if ab'+a'b=2hh'.
View SolutionIf points (a, 0), (0, b) and (1, 1) are collinear, then 1a+1b= (a) 1 (b) 2 (c) 0 (d) −1
View Solution
