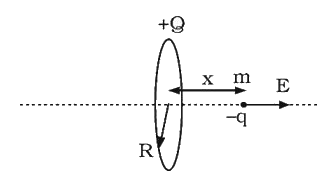
A thin fixed ring of radius a has a positive charge q uniformly distributed over it.A particle of mass m having a negative charge Q, is placed on the axis at a distance of x(x<<a) form the center of the ring. Show that the motion of the negatively charged particle is approximately simple harmonic. Calculate the time period of oscillation.

The correct Answer is:0.63
Eaxis=14πε0Qx(X2+R2)3/2
For x<<R,R2+x2≈R2
E≈Qx4πε0R3 , Fnet on negatively charged particle F=−Qqx(4πε0)R3
a=Fm=−Qqx(4πε0R3)m=−(Qq4πε0mR3)(x)
→a=−ω2x⇒SHMT=2πω=√4πε0mR3Qq⇒r=0.628sec≈063sec
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE MAIN65videos
ELECTROSTATICS
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - IMPECCABLE52videos
ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE1videos
ENERGY & MOMENTUM
Similar Questions
A thin fixed ring of radius a has a positive chage q unformly distributed over it.A particle of mass m having a megative charge Q, is placed on the axis at a distance of x(x<<a) form the center of the ring. Show that the motion of the negatively chaged particle is approximatly simple harmonic. Calculate the time period of oscillation.
 View Solution
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .
 View Solution
View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring.
 View Solution
View SolutionA thin semi-circular ring of radius r has a positive charge q distributed uniformly over it. The net field →E at the centre O is
 View Solution
View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring .The
 View Solution
View SolutionPositive charge Q is distributed uniformly over a circular ring of radius R. A particle having a mass m and a negative charge q, is placed on its axis at a distance x from the centre. Find the force on the particle. Assuming x is very less than R, find the time period of oscillation of the particle if it is released from there.
View SolutionA charge of 5 nC is uniformly distributed on a ring of radius 4 cm. Find the potential at the axis at a distance of 3 cm from the center.
View SolutionA ring of radius R carries a uniformly distributed charge +Q. A point charge −q is placed on the axis of the ring at a distance 2R from the centre of the ring and released from rest. The particle executes a simple harmonic motion along the axis of the ring. State True or False.
View SolutionFind the electric flux due to a point charge 'Q' through the circular region of radius R if the charge is placed on the axis of ring at a distance x.
 View Solution
View SolutionA charge +Q is uniformly distributed over a thin ring with radius R. A negative point charge −Q and mass m starts from rest at a point far away from the centre of the ring and moves towards the centre. Find the velocity of this particle at the moment it passes through the centre of the ring .
View Solution

