A ball thrown vertically upwards with an initial velocity of 1.4m/s. The total displcement of the ball is
22.4cm
zero
44.8m
33.6m
The correct Answer is:B
To solve the problem of finding the total displacement of a ball thrown vertically upwards with an initial velocity of 1.4 m/s, we can follow these steps:
1. Understanding Displacement: Displacement is defined as the change in position of an object. It is a vector quantity that has both magnitude and direction.
2. Initial Conditions: The ball is thrown vertically upwards with an initial velocity (u) of 1.4 m/s.
3. Motion of the Ball: As the ball moves upwards, it will eventually reach a maximum height where its velocity will become zero due to the acceleration of gravity acting downwards (g = 9.81 m/s²).
4. Maximum Height Calculation:
- To find the maximum height (h) reached by the ball, we can use the kinematic equation:
v2=u2+2as
where:
- v=0 m/s (final velocity at the maximum height),
- u=1.4 m/s (initial velocity),
- a=−g=−9.81 m/s² (acceleration due to gravity),
- s=h (displacement or height reached).
Rearranging the equation gives:
0=(1.4)2+2(−9.81)h
0=1.96−19.62h
19.62h=1.96
h=1.9619.62≈0.1 m
5. Total Displacement:
- After reaching the maximum height, the ball will fall back down to the original position (the point from where it was thrown).
- Since displacement is the difference between the final position and the initial position, and both positions are the same (the ball returns to the original point), the total displacement is:
Total Displacement=Final Position−Initial Position=0 m
Conclusion:
The total displacement of the ball is 0 meters.
- To find the maximum height (h) reached by the ball, we can use the kinematic equation:
v2=u2+2as
where:
- v=0 m/s (final velocity at the maximum height),
- u=1.4 m/s (initial velocity),
- a=−g=−9.81 m/s² (acceleration due to gravity),
- s=h (displacement or height reached).
Rearranging the equation gives:
0=(1.4)2+2(−9.81)h
0=1.96−19.62h
19.62h=1.96
h=1.9619.62≈0.1 m
- After reaching the maximum height, the ball will fall back down to the original position (the point from where it was thrown).
- Since displacement is the difference between the final position and the initial position, and both positions are the same (the ball returns to the original point), the total displacement is:
Total Displacement=Final Position−Initial Position=0 m
The total displacement of the ball is 0 meters.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - EFFICIENT
50 Videos
- MOCK TEST 9BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 9EXERCISE - PHYSICS (SECTION 2)
5 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
Similar Questions
A ball is thrown vertically upwards with an initial velocity of 49 m s−1. Calculate the time taken by it before it reaches the groung again (Take g= 9.8 m s−2)
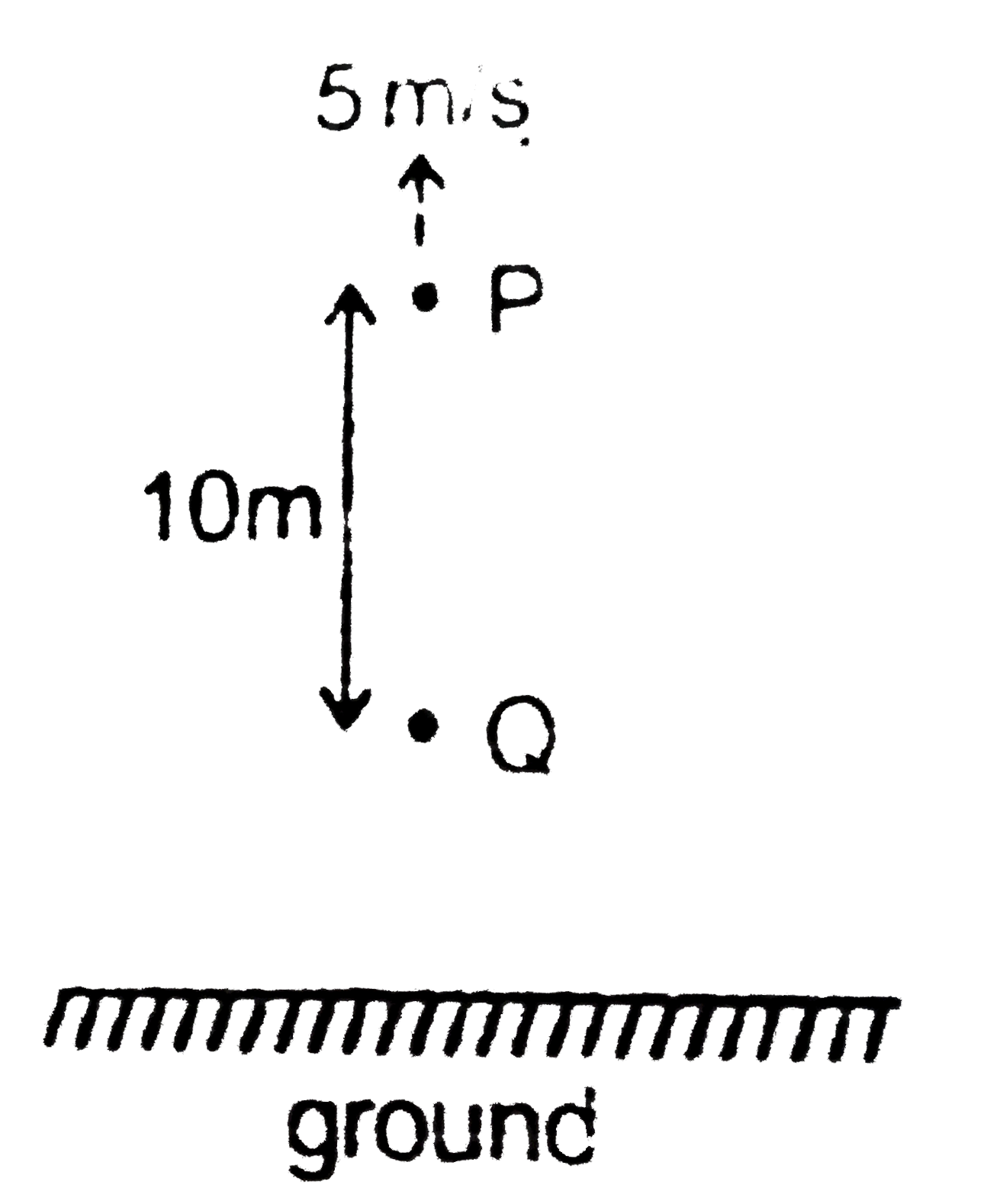
View SolutionA ball is thrown vertically upwards with an initial velocity of 5m/sec from point P as shown Q is a point 10m vertically below the point P. Then the speed of ball at point Q will be : (take g=10m/s2 and neglect air resistance)
View SolutionA ball is thrown vertically upward from the 12 m level with an initial velocity of 18m/s. At the same instant an open platform elevator passes the 5 m level, moving upward with a constant velocity of 2m/s. Determine (g=9.8m/s2 )
(a) when and where the ball will meet the elevator,
(b) the relative velocity of the ball with respect to the elevator when the ball hits the elevator.
View SolutionIf a ball is thrown vertically upwards with a velocity of 40m/s, then velocity of the ball after 2s will be (g=10m/s2)
(a) 15m/s (b) 20m/s (c) 25m/s (d) 28m/s
View SolutionA ball thrown vertically upward with initial velocity u. Which of the following graphs represent the velocity of ball correctly as function of time?
(Take upward direction as positive)
View SolutionA pebble thrown vertically upwards with an initial velocity 50 m s−1 comes to a stop in 5 s. Find the retardation.
View SolutionA ball is thrown vertically upwards with a velocity of 49m/s. Calulate
(i) The maximum height to which it rises,
(ii) the total time it takes to return to the surface of the earth.
View SolutionA ball is projected vertically up wards with a velocity of 100 m/s. Find the speed of the ball at half the maximum height. (g=10m/s2)
View SolutionA body is thrown vertically upwards with an initial velocity of 9.8ms−1. What is its speed and direction after 1 s (g=9.8 m/s2). Also, find the height to which it will rise.
View SolutionA ball is thrown vertically upward with a velocity of 20 m/s. Calculate the maximum height attain by the ball.
View Solution
