A cyclotron in which protons are accelerated has a flux density of 1.57t. The variation of frequency of electric field is ( in Hz)
4.8×108 cycles/sec
2.5×107 cycles/sec
4.8×106 cycles/sec
8.4×108 cycles/sec
The correct Answer is:B
To find the variation of frequency of the electric field in a cyclotron where protons are accelerated, we can use the formula for the frequency of the electric field in a cyclotron:
1. Identify the Given Values:
- Magnetic flux density, B=1.57T
- Charge of a proton, q=1.6×10−19C
- Mass of a proton, m=1.67×10−27kg
2. Use the Formula for Frequency:
The frequency f of the electric field in a cyclotron is given by the formula:
f=qB2πm
3. Substitute the Values into the Formula:
f=(1.6×10−19C)(1.57T)2π(1.67×10−27kg)
4. Calculate the Denominator:
First, calculate 2πm:
2π(1.67×10−27)≈3.14×1.67×10−27≈3.14×1.67×10−27≈5.24×10−27
5. Calculate the Numerator:
Now calculate the numerator qB:
(1.6×10−19)(1.57)≈2.512×10−19
6. Combine the Results:
Now, substitute the calculated values into the frequency formula:
f=2.512×10−195.24×10−27≈4.79×107Hz
7. Final Result:
The variation of frequency of the electric field is approximately:
f≈4.79×107Hz
- Magnetic flux density, B=1.57T
- Charge of a proton, q=1.6×10−19C
- Mass of a proton, m=1.67×10−27kg
The frequency f of the electric field in a cyclotron is given by the formula:
f=qB2πm
f=(1.6×10−19C)(1.57T)2π(1.67×10−27kg)
First, calculate 2πm:
2π(1.67×10−27)≈3.14×1.67×10−27≈3.14×1.67×10−27≈5.24×10−27
Now calculate the numerator qB:
(1.6×10−19)(1.57)≈2.512×10−19
Now, substitute the calculated values into the frequency formula:
f=2.512×10−195.24×10−27≈4.79×107Hz
The variation of frequency of the electric field is approximately:
f≈4.79×107Hz
Topper's Solved these Questions
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - EFFICIENT
50 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IMPECCABLE
53 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K
10 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
1 Videos
Similar Questions
In the Dubna heavy ion cyclotron neon ions are accelerated to an energy of 100 MeV. The diameter of the dees is 310 cm, the magnetic field induction in the gap is 1.1 T the accelerating potential is 300 kV. Find the degree of ionization of a neon atom, the total number of revolutions of an ion in the process of its acceleration and the frequency of the change in polarity of the accelerating field.
View SolutionThe law which states that the variations of electric field causes magnetic field is
View Solution
Knowledge Check
A cyclotron is operated at an oscillator frequency of 12 MHz and has a dee radius R 50 cm. What is the magnitude of the magnetic field needed for a proton to be accelerated in the cyclotron?
A0.78 TB0.65 TC0.39 TD0.12 T
A non-conducting solid sphere of radius R, has a uniform charge density. The graph representing variation of magnitude of electric field (E) as a function of distance (x) from the centre of the sphere is
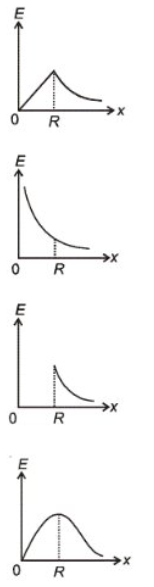
View SolutionThe pair of particles which have same acceleration in a uniform electric field is
View SolutionAnswer the following:
(i) Obtain the expression for the cyclotron frequency.
(ii) A deuteron and a proton are accelerated by the cyclotron. Can both be accelerated with the same oscillator frequency ? Give reason to justify your answer.
View SolutionA source of frequency 'f' is stationary and an obsercer starts moving towards it at t=0 with constant small acceleration. Then the variation of observed frequency 'f' registered by the observer with time is best represented as
View SolutionThe magnetic field In a travelling electromagnetic wave has a peak value of 20nT The peak value of electric field strength is :
View SolutionThe diameter of the magnet of the Serpukhov synchrotron is 472 m. The protons enter the accelerating chamber with an energy of 100 MeV and leave it with an energy of 76.5 GeV. Find the initial and the final magnetic induction in the gap and the accelerating field frequencies. We
View SolutionA non-conducting semi circular disc (as shown in figure) has a uniform surface charge density σ. The ratio of electric field to electric potential at the centre of the disc will be
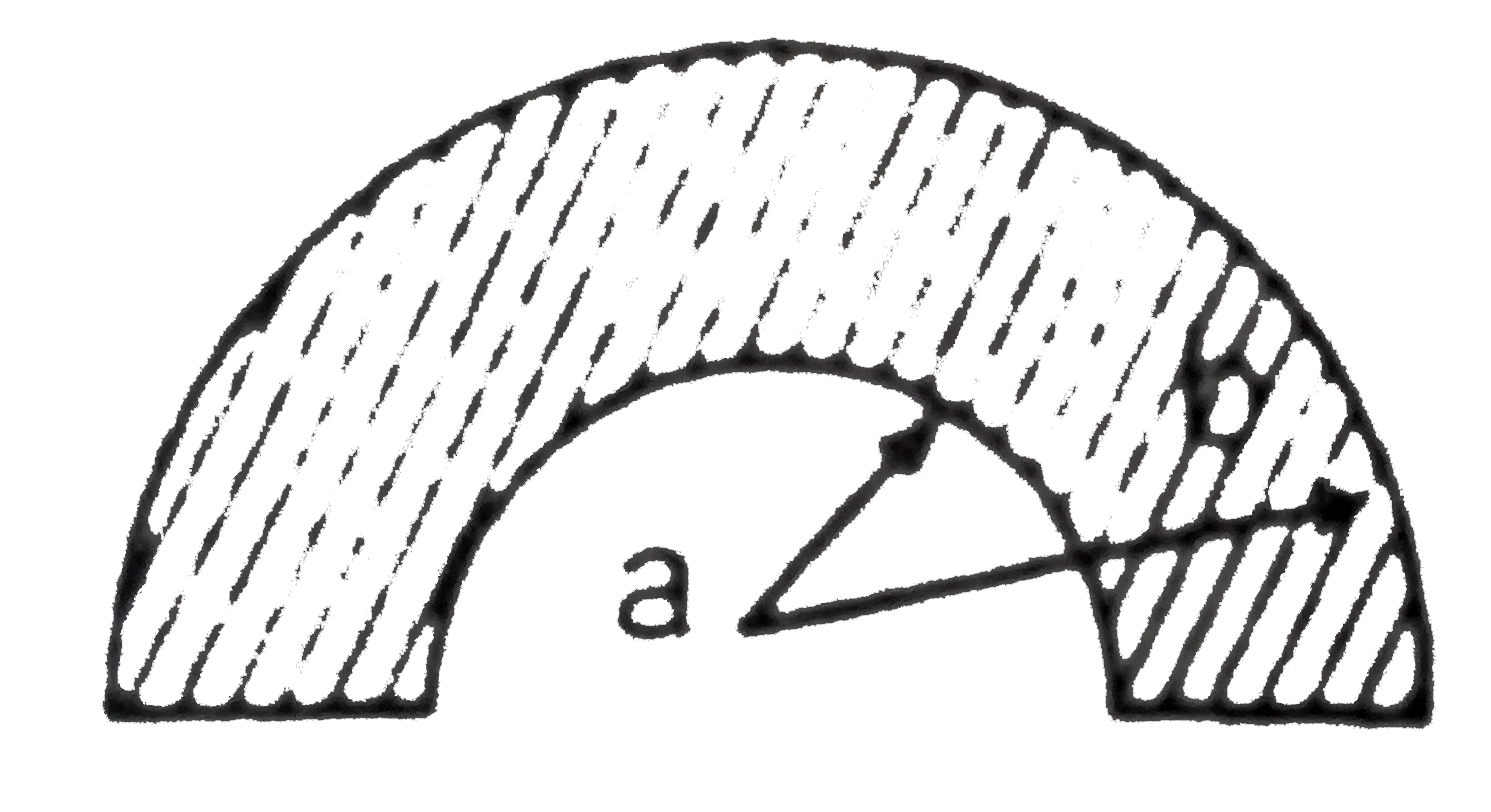
View Solution
