Two very long, straight, parallel wires carry steady currents I and −I respectively.The distance between the wires is d.At a certain instant of time, a point charge q is at a point equidistant from the two wires,in the plane of wires.Its instantaneous velocity →v is perpendicular to this plane.The magnitude of the force due to the magnetic field acting on the charge at this instant is:
μ0Iqv2πd
μ0Iqvπd
2μ0Iqvπd
zero
The correct Answer is:D
To solve the problem, we need to analyze the situation involving two long, straight, parallel wires carrying currents and the magnetic field they produce, as well as the force experienced by a point charge in that magnetic field.
1. Understand the Configuration:
- We have two long, straight, parallel wires. Wire 1 carries a current I upwards, and Wire 2 carries a current −I (downwards).
- The distance between the two wires is d.
- A point charge q is placed at a point equidistant from both wires, which we can denote as point A.
- The instantaneous velocity →v of the charge is perpendicular to the plane formed by the two wires.
2. Determine the Magnetic Fields:
- The magnetic field →B produced by a long straight wire at a distance r from it is given by the formula:
B=μ0I2πr
- At point A, which is equidistant from both wires, the distance from each wire to point A is d2.
3. Calculate the Magnetic Fields at Point A:
- For Wire 1 (current I):
B1=μ0I2πd2=μ0Iπd
- For Wire 2 (current −I):
B2=μ0(−I)2πd2=−μ0Iπd
- The direction of B1 (due to Wire 1) is out of the plane, and the direction of B2 (due to Wire 2) is into the plane.
4. Net Magnetic Field at Point A:
- Since B1 and B2 are equal in magnitude but opposite in direction, they will cancel each other out:
Bnet=B1+B2=μ0Iπd−μ0Iπd=0
5. Calculate the Magnetic Force on the Charge:
- The magnetic force →F on a charge moving in a magnetic field is given by:
→F=q→v×→B
- Since the net magnetic field Bnet=0, the force on the charge will also be zero:
F=qvBnet=qv⋅0=0
6. Conclusion:
- The magnitude of the force due to the magnetic field acting on the charge at this instant is:
F=0
- We have two long, straight, parallel wires. Wire 1 carries a current I upwards, and Wire 2 carries a current −I (downwards).
- The distance between the two wires is d.
- A point charge q is placed at a point equidistant from both wires, which we can denote as point A.
- The instantaneous velocity →v of the charge is perpendicular to the plane formed by the two wires.
- The magnetic field →B produced by a long straight wire at a distance r from it is given by the formula:
B=μ0I2πr
- At point A, which is equidistant from both wires, the distance from each wire to point A is d2.
- For Wire 1 (current I):
B1=μ0I2πd2=μ0Iπd
- For Wire 2 (current −I):
B2=μ0(−I)2πd2=−μ0Iπd
- The direction of B1 (due to Wire 1) is out of the plane, and the direction of B2 (due to Wire 2) is into the plane.
- Since B1 and B2 are equal in magnitude but opposite in direction, they will cancel each other out:
Bnet=B1+B2=μ0Iπd−μ0Iπd=0
- The magnetic force →F on a charge moving in a magnetic field is given by:
→F=q→v×→B
- Since the net magnetic field Bnet=0, the force on the charge will also be zero:
F=qvBnet=qv⋅0=0
- The magnitude of the force due to the magnetic field acting on the charge at this instant is:
F=0
Topper's Solved these Questions
- MOVING CHARGES & MAGNETISM Book:VMC MODULES ENGLISHChapter:MOVING CHARGES & MAGNETISM Exercise:IMPECCABLE
Explore 53 Videos
- MOVING CHARGES & MAGNETISM Book:VMC MODULES ENGLISHChapter:MOVING CHARGES & MAGNETISM Exercise:Illustration
Explore 55 Videos
- MOVING CHARGES & MAGNETISM Book:VMC MODULES ENGLISHChapter:MOVING CHARGES & MAGNETISM Exercise:ENABLE
Explore 50 Videos
- Motion in Two DimensionsBook:VMC MODULES ENGLISHChapter:Motion in Two DimensionsExercise:MCQ
Explore 2 Videos
- PROPERTIES OF MATTERBook:VMC MODULES ENGLISHChapter:PROPERTIES OF MATTERExercise:JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
Explore 1 Video
Similar Questions
Two very long, straight , parallel wires carry steady currents I&−I respectively . The distance between the wires is d. At a certain instant of time, a point charge q is at a point equidistant from the wires , in the plane of the wires. Its instantaneous vel,ocity v is perpendicular to this plane. The magnitude of the force due to the magnetic field acting on the charge at this instant is
View SolutionTwo very long straight parallel wires carry steady currents i and 2i in opposite directions. The distance between the wires is d. At a certain instant of time a point charge q is at a point equidistant from the two wires in the plane of the wires. Its instantaneous velocity →v is perpendicular to this plane. The magnitude of the force due to the magnetic field acting on t he charge at this instant is
View SolutionThe two long, straight wires carrying electric currents in opposite directions. The separation between the wires is 5.0 cm. Find the magnetic field at a point P midway between the wires.
View SolutionTwo perpendicular infinite wires are carrying current i as shown in the figure. The magnetic field at point P is :
_2019_CBT2_E01_008_Q01.png)
View SolutionTwo long parallel wires carry equal current I flowing in the same direction are at a distance 2d apart. The magnetic field B at a point lying on the perpendicular line joining the wires and at a distance x from the midpoint is
View SolutionIn Fig. two long parallel wires (seen end-on) that are a distance R apart carry equal currents i in the same sense. Find the magnitude of the magnetic field at point P , which lies quidistant from the two wires at an angle θ from the plane of the wires.
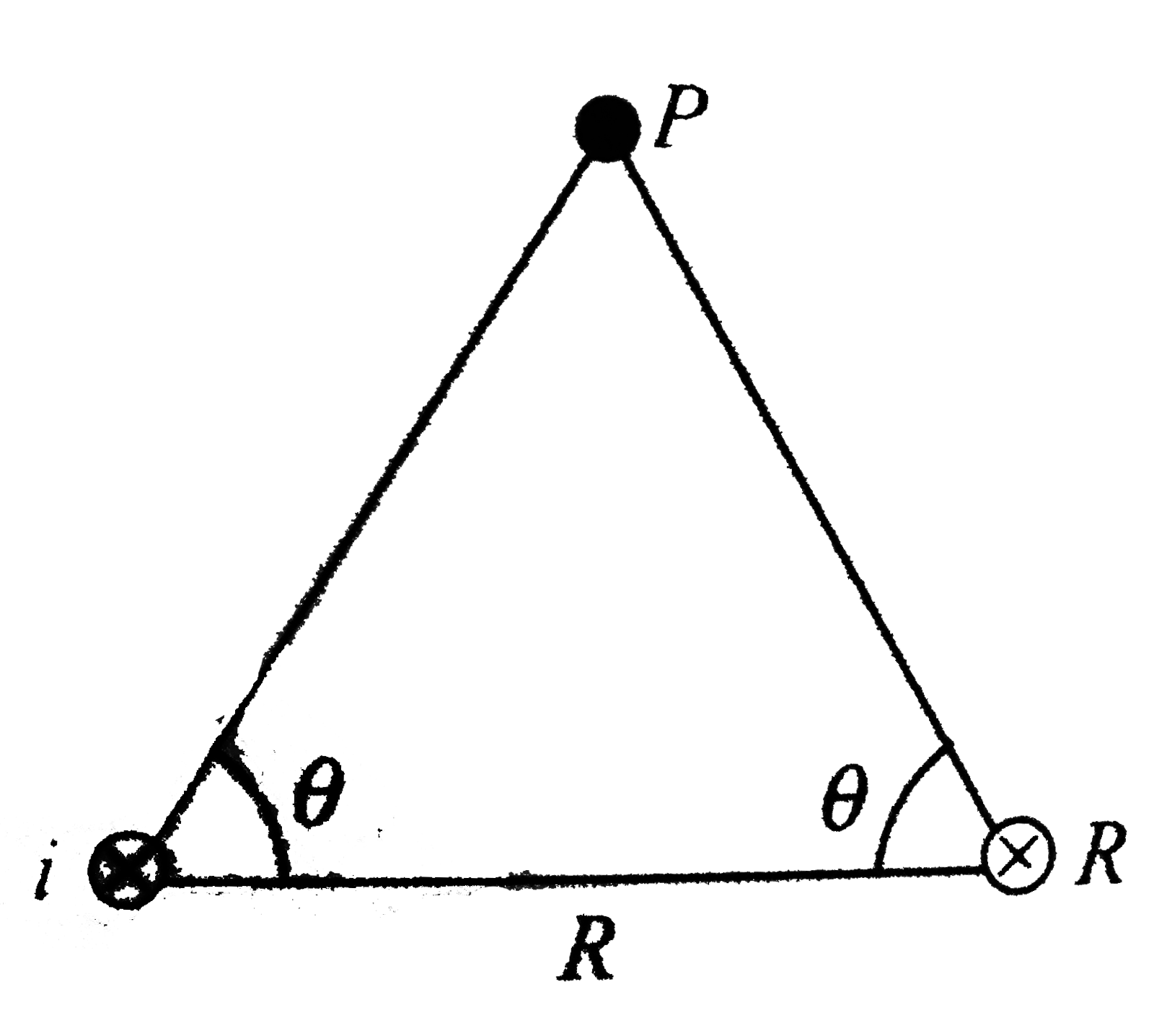
View SolutionTwo thin, long, parallel wires, separated by a distance 'd' carry a current of 'i' A in the same direction. They will
View SolutionA semicircular wire of radius 5.0 cm carries a current of 5.0 A. A magnetic field B of magnitude 0.50 T exists along the perpendicular to the plane of the wire. Find the magnitude of the magnetic force acting on the wire.
View SolutionTwo long parallel copper wires carry current of 5A each in opposite directions. If the wires are separated by a distance distance of 0.5m, then the force per unit length between the two wires is
View Solutiona very long straight wire carries a current I. at the instant when are charge -Q at point P has velocity v as shown in figure the force on the charge is

View Solution