The magnetic field due to short bar magnet of magnetic dipole moment M and length 21, on the axis at a distance z (where z>>I) from the centre of the magnet is given by formula
μ0M4πz3T
2μ0M4πz3T
4μ0Mπz3T
μ0M2πz3T
The correct Answer is:B
To find the magnetic field due to a short bar magnet of magnetic dipole moment M and length 2L at a distance z on the axis (where z≫L), we can follow these steps:
Step 1: Understand the Configuration
We have a short bar magnet with a magnetic dipole moment M and length 2L. The distance from the center of the magnet to the point where we want to calculate the magnetic field is z.
Step 2: Identify the Points of Interest
The magnetic field at point P on the axis of the magnet can be calculated by considering the contributions from both poles of the magnet:
- The distance from the negative pole (−M) to point P is z−L.
- The distance from the positive pole (+M) to point P is z+L.
Step 3: Write the Magnetic Field Expressions
The magnetic field B due to a magnetic dipole at a distance r along the axial line is given by:
B=μ04π2Mr3
Thus, the magnetic field contributions from both poles at point P are:
B−M=μ04π−M(z−L)2
B+M=μ04πM(z+L)2
Step 4: Combine the Magnetic Fields
The total magnetic field B at point P is the sum of the contributions from both poles:
B=B+M+B−M=μ04π(M(z+L)2−M(z−L)2)
Step 5: Simplify the Expression
To simplify the expression, we can find a common denominator:
B=μ0M4π((z−L)2−(z+L)2(z+L)2(z−L)2)
Expanding the numerator:
(z−L)2−(z+L)2=z2−2zL+L2−(z2+2zL+L2)=−4zL
Thus, we have:
B=μ0M4π(−4zL(z+L)2(z−L)2)
Step 6: Approximate for z≫L
Since z≫L, we can approximate:
(z+L)2≈z2and(z−L)2≈z2
Therefore:
B≈μ0M4π(−4zLz2⋅z2)=μ0M4π⋅−4Lz4
This simplifies to:
B≈2μ0M4πz3
Final Answer
Thus, the magnetic field at a distance z on the axis of a short bar magnet is given by:
B=2μ0M4πz3
We have a short bar magnet with a magnetic dipole moment M and length 2L. The distance from the center of the magnet to the point where we want to calculate the magnetic field is z.
The magnetic field at point P on the axis of the magnet can be calculated by considering the contributions from both poles of the magnet:
- The distance from the negative pole (−M) to point P is z−L.
- The distance from the positive pole (+M) to point P is z+L.
The magnetic field B due to a magnetic dipole at a distance r along the axial line is given by:
B=μ04π2Mr3
Thus, the magnetic field contributions from both poles at point P are:
B−M=μ04π−M(z−L)2
B+M=μ04πM(z+L)2
The total magnetic field B at point P is the sum of the contributions from both poles:
B=B+M+B−M=μ04π(M(z+L)2−M(z−L)2)
To simplify the expression, we can find a common denominator:
B=μ0M4π((z−L)2−(z+L)2(z+L)2(z−L)2)
Expanding the numerator:
(z−L)2−(z+L)2=z2−2zL+L2−(z2+2zL+L2)=−4zL
Thus, we have:
B=μ0M4π(−4zL(z+L)2(z−L)2)
Since z≫L, we can approximate:
(z+L)2≈z2and(z−L)2≈z2
Therefore:
B≈μ0M4π(−4zLz2⋅z2)=μ0M4π⋅−4Lz4
This simplifies to:
B≈2μ0M4πz3
Thus, the magnetic field at a distance z on the axis of a short bar magnet is given by:
B=2μ0M4πz3
Topper's Solved these Questions
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - Illustration
55 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - SOLVED EXAMPLES
20 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - EFFICIENT
50 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
1 Videos
Similar Questions
The magnetic field on the axis at a distance z from the centre of the bar magnet would be ?
View SolutionThe dipole moment of a short bar magnet is 1.25A−m2. The magnetic field on its axis at a distance of 0.5 metre from the centre of the magnet is
View SolutionThe magnet field lines due to a bar magnet are correctly shown in
View SolutionThe magnet field lines due to a bar magnet are correctly shown in
View SolutionMagnetic intensity for an axial point due to a short bar magnet of magnetic moment M is given by
View SolutionThe field due to a magnet at a distance R~ from the centre of the magnet is proportional
View SolutionThe magnetic field intensity due to a very small bar magnet having magnetic dipole moment as 2.5Am2 at end on position at a distance of 0.5m in μT is_______.
View SolutionThe ratio of the magnetic fields due to small bar magnet in end position an broad side on position is (at equal distance from the magnet)
View SolutionThe magnetic induction field strength due to a short bar magnet at a distance 0.20 m on the equatorial line is 20×10−6T. The magnetic moment of the bar magnet is
View SolutionA bar magnet of lenth l and magnetic dipole moment 'M' is bent in the form of an arc as shown in figure. The new magnetic dipole moment will be
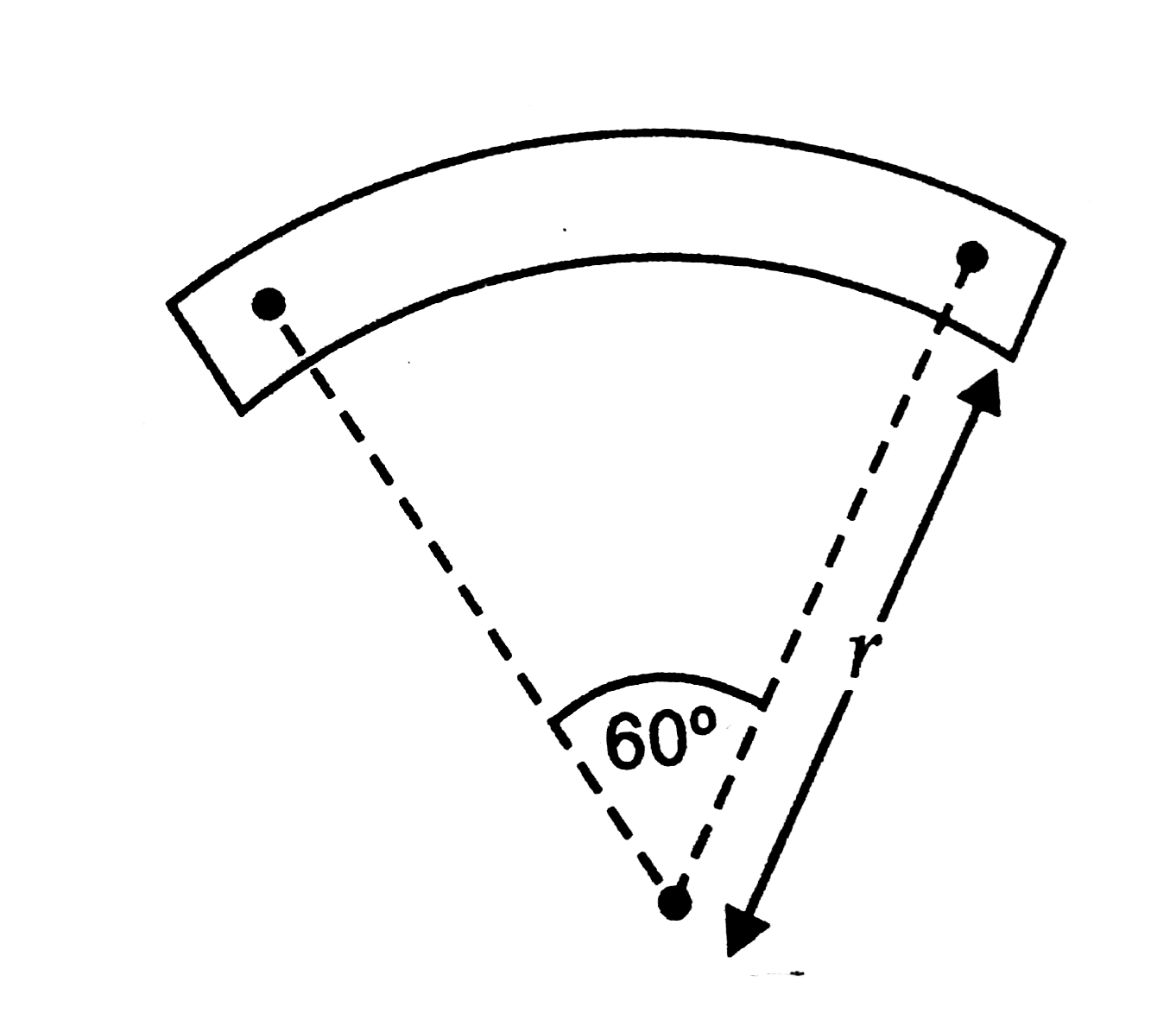
View Solution
