A magnetic wire of dipole moment 4πAm2 is bent in the form of semicircle. The new magnetic moment is
4πAm2
8πAm2
4Am2
none of thse
The correct Answer is:D
To solve the problem, we need to determine the new magnetic moment of a wire that has been bent into the shape of a semicircle. We start with the given dipole moment and follow through the steps logically.
1. Identify the Initial Magnetic Moment:
The initial magnetic moment M of the wire is given as:
M=4πA m2
2. Understand the Relationship of Magnetic Moment:
The magnetic moment M can be expressed as:
M=m⋅L
where m is the pole strength and L is the length of the wire.
3. Determine the Initial Length:
Let the initial length of the wire be L.
4. Bend the Wire into a Semicircle:
When the wire is bent into a semicircle, the length of the semicircle is equal to the initial length L:
Circumference of semicircle=πR=L
From this, we can find the radius R:
R=Lπ
5. Calculate the New Length:
The total length of the wire when bent into a semicircle is:
L′=2R=2(Lπ)=2Lπ
6. Determine the New Magnetic Moment:
The new magnetic moment M′ can be calculated using the pole strength m which remains constant:
M′=m⋅L′
Since m=ML=4πL, we substitute this into the equation:
M′=(4πL)⋅(2Lπ)
7. Simplify the Expression:
Simplifying the expression gives:
M′=4π⋅2LL⋅π=8ππ=8A m2
8. Final Answer:
Therefore, the new magnetic moment when the wire is bent into a semicircle is:
M′=8A m2
The initial magnetic moment M of the wire is given as:
M=4πA m2
The magnetic moment M can be expressed as:
M=m⋅L
where m is the pole strength and L is the length of the wire.
Let the initial length of the wire be L.
When the wire is bent into a semicircle, the length of the semicircle is equal to the initial length L:
Circumference of semicircle=πR=L
From this, we can find the radius R:
R=Lπ
The total length of the wire when bent into a semicircle is:
L′=2R=2(Lπ)=2Lπ
The new magnetic moment M′ can be calculated using the pole strength m which remains constant:
M′=m⋅L′
Since m=ML=4πL, we substitute this into the equation:
M′=(4πL)⋅(2Lπ)
Simplifying the expression gives:
M′=4π⋅2LL⋅π=8ππ=8A m2
Therefore, the new magnetic moment when the wire is bent into a semicircle is:
M′=8A m2
Topper's Solved these Questions
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - Illustration
55 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - SOLVED EXAMPLES
20 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - EFFICIENT
50 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
1 Videos
Similar Questions
Knowledge Check
A thin wire of length l and mass m is bent in the form of a semicircle as shown in the figure. Its moment of inertia about an axis joining its free ends will be
 Aml2BzeroCml2π2Dml22π2
Aml2BzeroCml2π2Dml22π2
A bar magnet of lenth l and magnetic dipole moment 'M' is bent in the form of an arc as shown in figure. The new magnetic dipole moment will be
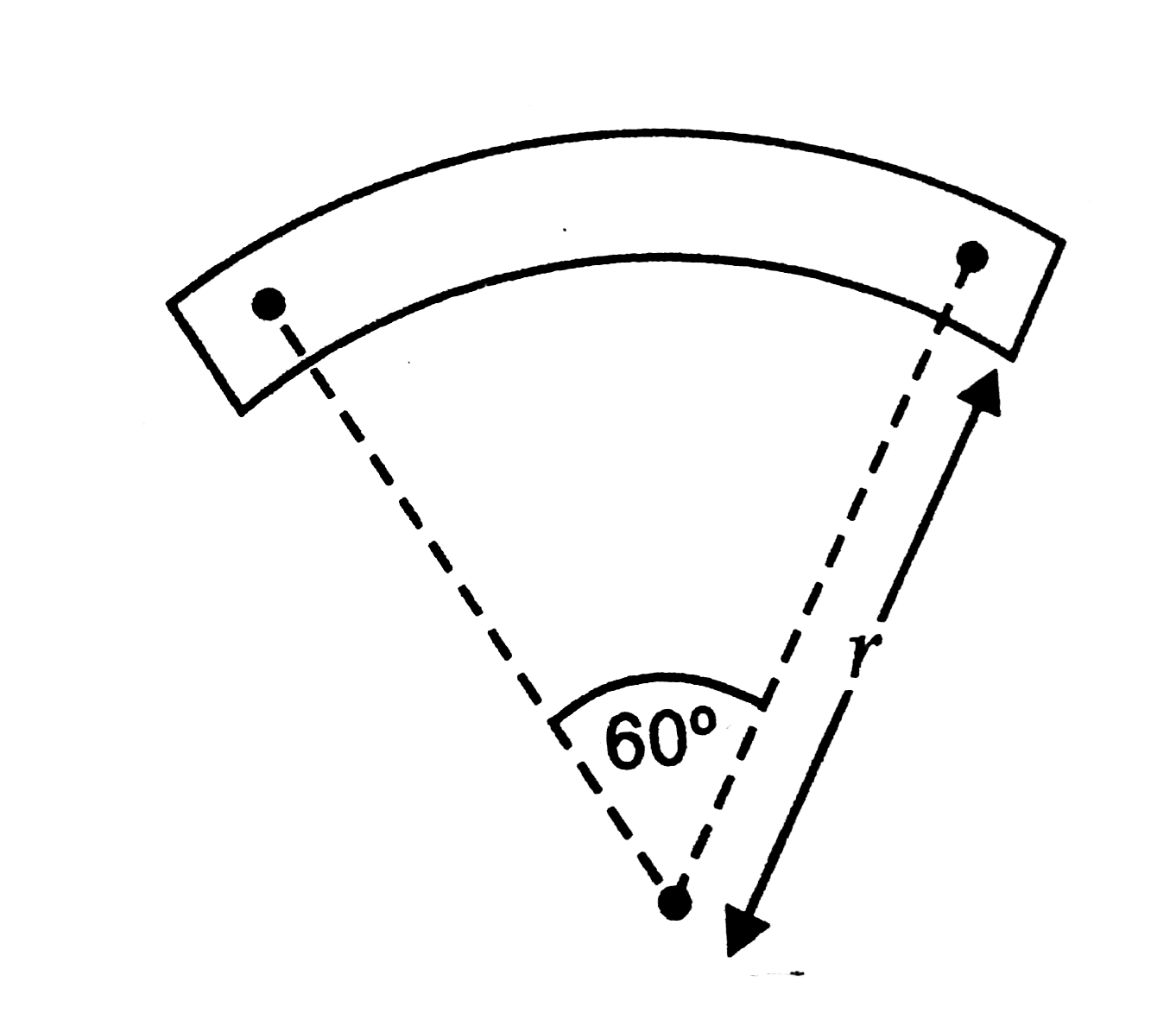
View SolutionAn iron rod of length L and magnetic moment M is bent in the form of a semicircle. Now its magnetic moment will be
View SolutionA long thin magnet of moment M is bent into a semi circle. The decrease in the magnetic moment is
View SolutionWith reference to magnetic dipole, match the tems of Column I with the tems of Column Ii and Choose the correct option from the codes given below.

View SolutionA wire of length l meter carrying current i ampere is bent in form of circle. Magnetic moment is
View SolutionA wire of length Lmetre , carrying a current Iampere is bent in the form of a circle . The magnitude of its magnetic moment is …………. MKSunits .
View SolutionA thin wire of length l and mass m is bent in the form of a semicircle as shown in the figure. Its moment of inertia about an axis joining its free ends will be

View Solution
