An alternating current source of frequency is joined to a combination of a resistance, a capacitance and a coil in series. The potential difference across the coil, the resistance and the capacitor is volt respectively. The electromotive force of alternating current source in volt is
94
14
10
76
The correct Answer is:C
To find the electromotive force (emf) of the alternating current source in the given circuit, we will follow these steps:
Step 1: Identify the given values
- Voltage across the coil (inductor), VL=46V
- Voltage across the resistor, VR=8V
- Voltage across the capacitor, VC=40V
Step 2: Use the formula for the net voltage in an RLC series circuit
In a series RLC circuit, the total voltage (emf) can be calculated using the following formula:
E=√(VL−VC)2+V2R
Step 3: Substitute the known values into the formula
Substituting the values we have:
E=√(46−40)2+82
Step 4: Simplify the expression
Calculate the difference:
E=√(6)2+(8)2
Step 5: Calculate the squares
Now, calculate the squares:
E=√36+64
Step 6: Add the results
Add the results:
E=√100
Step 7: Take the square root
Finally, take the square root:
E=10V
Conclusion
The electromotive force of the alternating current source is 10V.
---
- Voltage across the coil (inductor), VL=46V
- Voltage across the resistor, VR=8V
- Voltage across the capacitor, VC=40V
In a series RLC circuit, the total voltage (emf) can be calculated using the following formula:
E=√(VL−VC)2+V2R
Substituting the values we have:
E=√(46−40)2+82
Calculate the difference:
E=√(6)2+(8)2
Now, calculate the squares:
E=√36+64
Add the results:
E=√100
Finally, take the square root:
E=10V
The electromotive force of the alternating current source is 10V.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - ENABLE
50 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - EFFICIENT
49 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - IN-CHAPTER EXERCISE-G
10 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)
89 Videos
Similar Questions
An alternating current of 1.5 mA with angular frequency 100 rad/s flows through a 10kΩ resistor and a 0.50μF capacitor in series. The rms potential drop across the capacitor is
View SolutionA 100 volt AC source of angular frequency 500 rad/s is connected to a LCR circuit with L = 0.8 H, C=5muF and R = 10Omega, all connected in series. The potential difference across the resistance is
View SolutionThe potential difference across 8 ohm resistance is 48 volt as shownin the figure. The value of potential difference across X and Y point will be
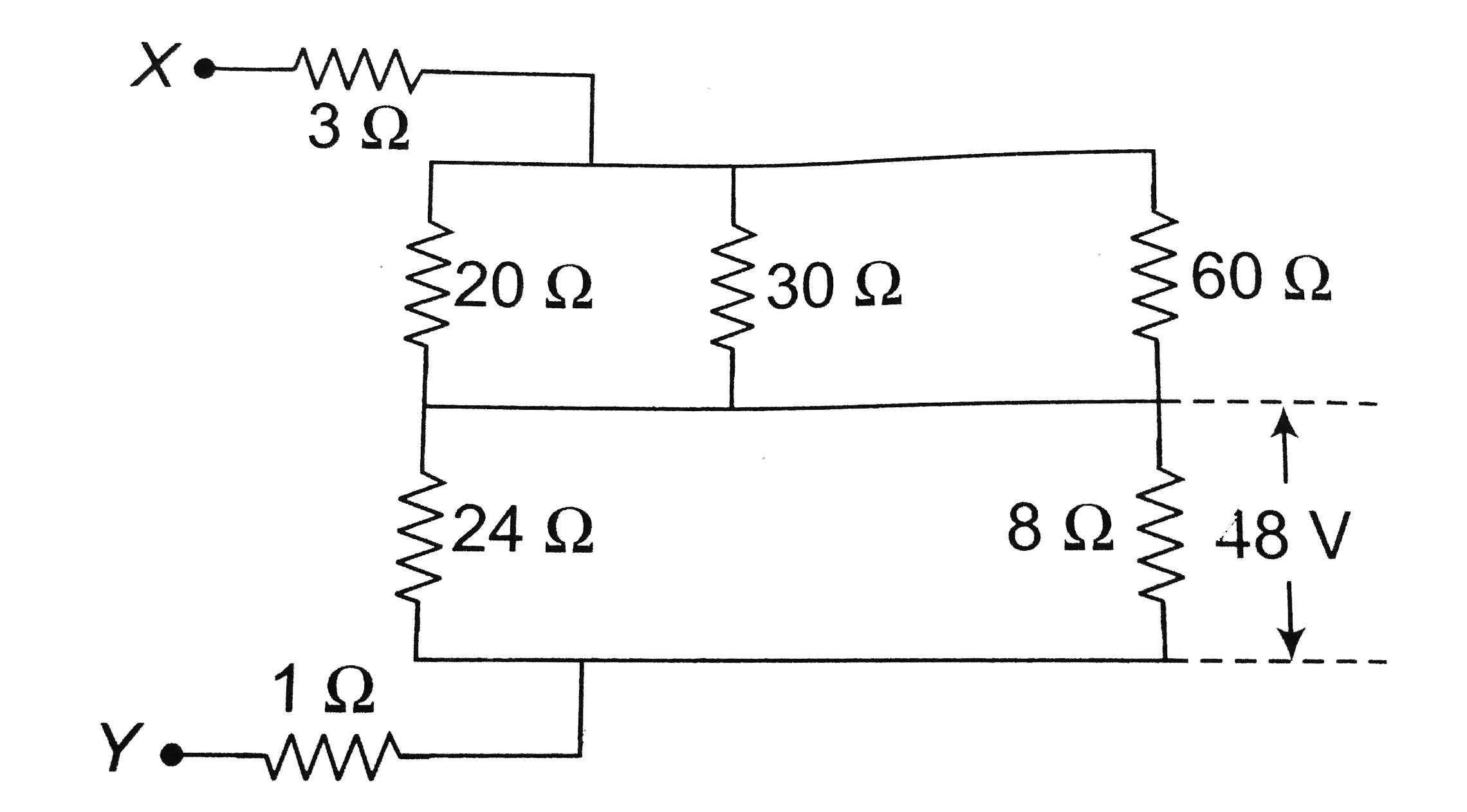
View SolutionThe potential difference across 8 ohm resistance is 48 volt as shownin the figure. The value of potential difference across X and Y point will be

View SolutionIn an ac circuit the potential differences across an inductance and resistance joined in series are, respectively, 16 V and 20 V. The total potential difference across the circuit is
View SolutionIn an ac circuit the potential differences across an inductance and resistance joined in series are, respectively, 16 V and 20 V. The total potential difference across the circuit is
View SolutionWhen an AC signal of frequency 1kHz is applied across a coil of resistance 100Ω, then the applied voltage leads the current by 45∘. The inductance of the coil is
View SolutionA cell of emf ε and internal resistance r is charged by a current I, then
View SolutionThree capacitors 3μF,6μF and 6μF are connected in series to source of 120 V. The potential difference in volt, across the 3μF capacitor will be
View SolutionAn AC source is connected with a resistance ( R) and an unchanged capacitance C, in series. The potential difference across the resistor is in phase with the initial potential difference across the capacitor for the first time at the instant (assume that at t =0 , emf is zero)
View Solution
