Three capacitors 3μF,6μF and 6μF are connected in series to source of 120 V. The potential difference in volt, across the 3μF capacitor will be
24 V
30 V
40 V
60 V
The correct Answer is:D
To solve the problem of finding the potential difference across the 3 µF capacitor in a series circuit with three capacitors (3µF, 6µF, and 6µF) connected to a 120V source, we can follow these steps:
Step 1: Calculate the Equivalent Capacitance
For capacitors in series, the formula for the equivalent capacitance \( C_{eq} \) is given by:
\(
\frac{1}{C_{eq}} = \frac{1}{C_1} + \frac{1}{C_2} + \frac{1}{C_3}
\)
Substituting the values:
\(
\frac{1}{C_{eq}} = \frac{1}{3 \mu F} + \frac{1}{6 \mu F} + \frac{1}{6 \mu F}
\)
Calculating each term:
\(
\frac{1}{C_{eq}} = \frac{1}{3} + \frac{1}{6} + \frac{1}{6}
\)
Finding a common denominator (which is 6):
\(
\frac{1}{C_{eq}} = \frac{2}{6} + \frac{1}{6} + \frac{1}{6} = \frac{4}{6} = \frac{2}{3}
\)
Now, taking the reciprocal to find \( C_{eq} \):
\(
C_{eq} = \frac{3}{2} \mu F = 1.5 \mu F
\)
Step 2: Calculate the Total Charge in the Circuit
The total charge \( Q \) stored in the circuit can be calculated using the formula:
\(
Q = C_{eq} \times V
\)
Substituting the values:
\(
Q = 1.5 \mu F \times 120 V = 1.5 \times 10^{-6} F \times 120 V = 180 \times 10^{-6} C
\)
Step 3: Calculate the Potential Difference Across the 3µF Capacitor
Using the formula for charge \( Q = C \times V \), we can find the potential difference \( V_1 \) across the 3µF capacitor:
\(
V_1 = \frac{Q}{C_1}
\)
Substituting the values:
\(
V_1 = \frac{180 \times 10^{-6} C}{3 \mu F} = \frac{180 \times 10^{-6} C}{3 \times 10^{-6} F} = 60 V
\)
Conclusion
The potential difference across the 3µF capacitor is \( 60 V \).
---
For capacitors in series, the formula for the equivalent capacitance \( C_{eq} \) is given by:
\frac{1}{C_{eq}} = \frac{1}{C_1} + \frac{1}{C_2} + \frac{1}{C_3}
\)
\frac{1}{C_{eq}} = \frac{1}{3 \mu F} + \frac{1}{6 \mu F} + \frac{1}{6 \mu F}
\)
\frac{1}{C_{eq}} = \frac{1}{3} + \frac{1}{6} + \frac{1}{6}
\)
\frac{1}{C_{eq}} = \frac{2}{6} + \frac{1}{6} + \frac{1}{6} = \frac{4}{6} = \frac{2}{3}
\)
C_{eq} = \frac{3}{2} \mu F = 1.5 \mu F
\)
The total charge \( Q \) stored in the circuit can be calculated using the formula:
Q = C_{eq} \times V
\)
Q = 1.5 \mu F \times 120 V = 1.5 \times 10^{-6} F \times 120 V = 180 \times 10^{-6} C
\)
Using the formula for charge \( Q = C \times V \), we can find the potential difference \( V_1 \) across the 3µF capacitor:
V_1 = \frac{Q}{C_1}
\)
V_1 = \frac{180 \times 10^{-6} C}{3 \mu F} = \frac{180 \times 10^{-6} C}{3 \times 10^{-6} F} = 60 V
\)
The potential difference across the 3µF capacitor is \( 60 V \).
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
A series combination of three capacitors of capacities 1μF,2μF and 8μF is connected to a battery of e.m.f. 13 volt .The potential difference across the plates of 2μF capacitor will be
View SolutionIn the adjoning figure, the potential difference across the 4.5μF capacitor is

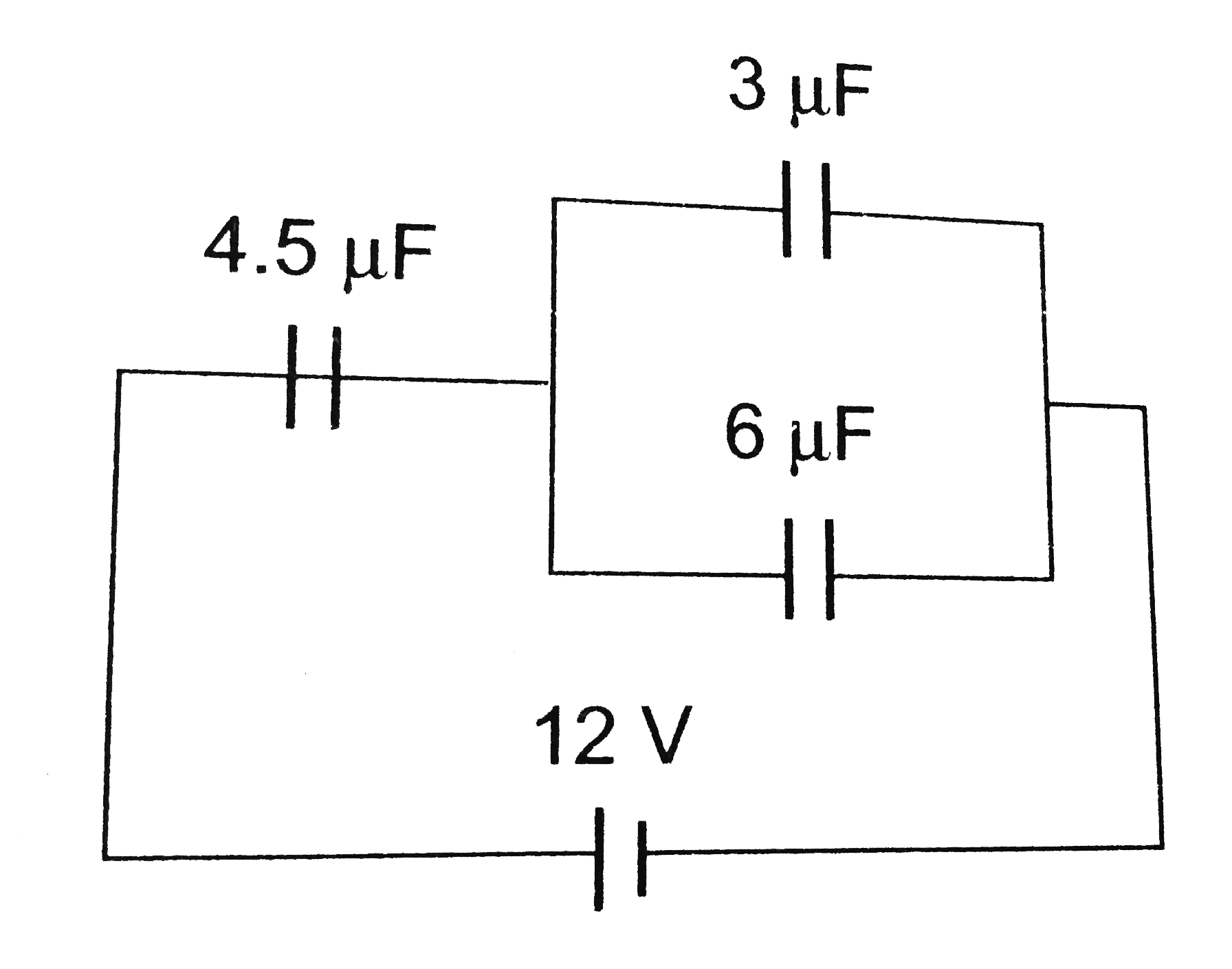
View SolutionIn the circuit shown in the figure, the potential difference across the 4.5μF capacitor is
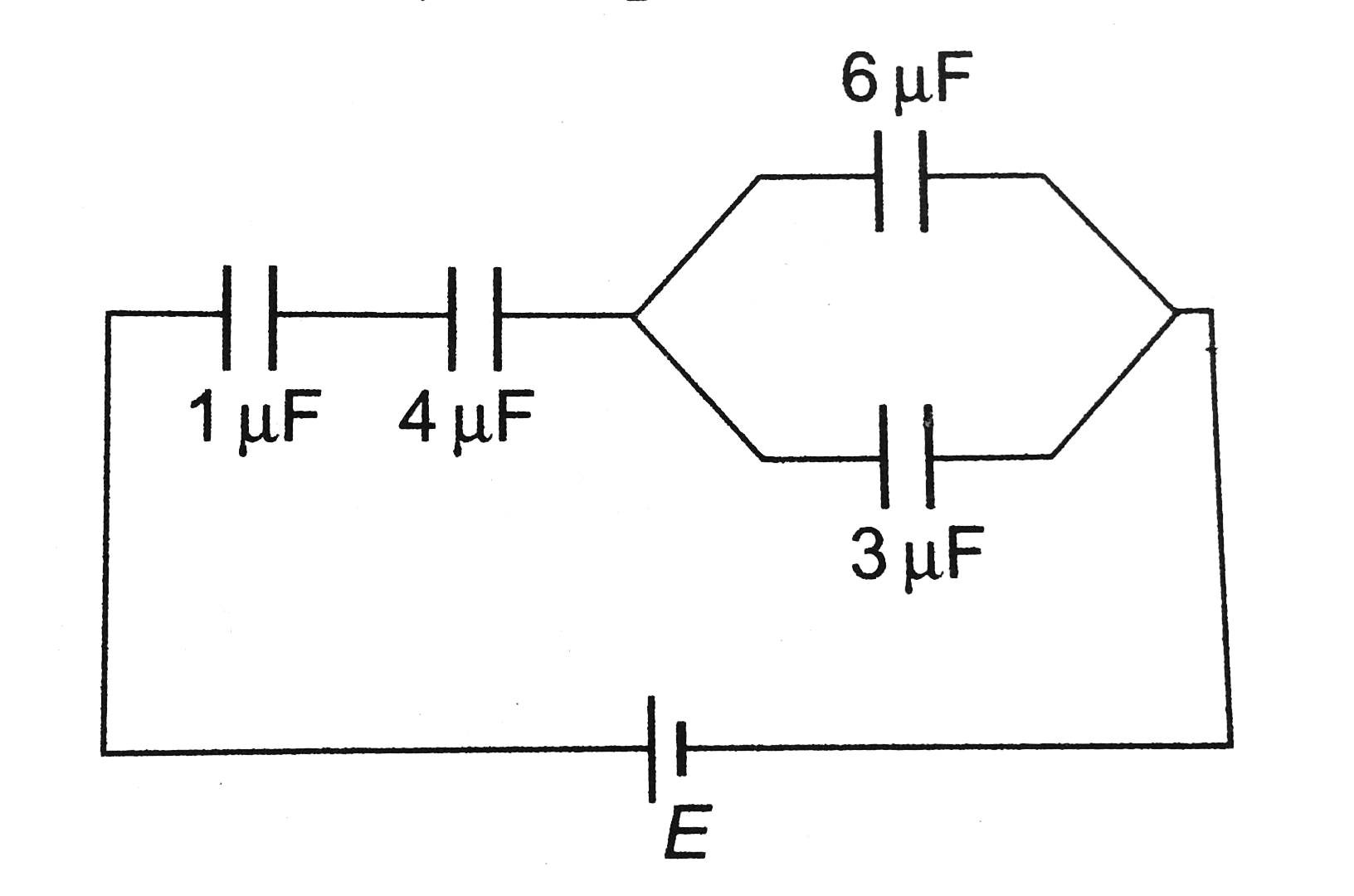
View Solutiongiven that potential differene across 1μF capacitor is 10V. Then,
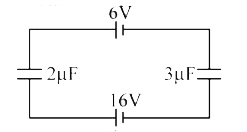
View SolutionWhat is the potential difference across 2μF capacitor in the circuit shown?
View SolutionThree capacitors having capacitances of 8.4μF,8.4μF and 4.2μF are connected in series across a 36 potential difference.
(a) What is the charge on 4.2μF capacitor?
(b) What is the total energy stored in all three capacitors?
(c) The capacitors are disconnected from the potential difference without allowing them to discharge. They are then reconnected in parallel with each other, with the positively charged plates connected together. What is the voltage across each capacitor in the parallel combination?
(d) What is the total energy now stored in the capacitors?
View SolutionThe capacitor of capacitance 4μF and 6μF are connected in series. A potential difference of 500 volts applied to the outer plates of the two capacitor system. Then the charge on each capacitor is numerically
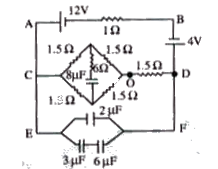
View SolutionIn the given circuit, the potential difference across the 6μF capacitor in steady state is
View SolutionThe ratio of potential differences between 1μFand5μF capacitors is
View SolutionThree capacitors of 2μF,3μF and 6μF are joined in series and the combination is charged by means of a 24 volt battery. The potential difference between the plates of the 6μF capacitor is
View Solution
