If number of turns of 70cm2 coil is 200 and it is placed in a magnetic field of 0.8Wb/m2 which is perpendicular to the plane of coil and it is rotated through an angle 180∘ in 0.1 sec , then induced emf in coil :
11.2V
1.12V
22.4V
2.24V
The correct Answer is:C
To solve the problem of induced EMF in a rotating coil placed in a magnetic field, we can follow these steps:
Step 1: Understand the Given Data
- Number of turns (N) = 200
- Area of the coil (A) = 70 cm² = 70 × 10⁻⁴ m² (conversion to m²)
- Magnetic field strength (B) = 0.8 Wb/m²
- Angle of rotation (θ) = 180°
- Time taken for rotation (t) = 0.1 s
Step 2: Calculate Initial and Final Magnetic Flux
The magnetic flux (Φ) through the coil is given by the formula:
Φ=N⋅B⋅A⋅cos(θ)
Initial Flux (Φ_initial):
- When the coil is perpendicular to the magnetic field, θ = 0°.
- Thus, cos(0°)=1.
- Therefore, the initial flux is:
Φinitial=N⋅B⋅A⋅cos(0°)=200⋅0.8⋅(70×10−4)
Φinitial=200⋅0.8⋅0.007=1.12 Wb
Final Flux (Φ_final):
- After rotating 180°, the angle becomes θ = 180°.
- Thus, cos(180°)=−1.
- Therefore, the final flux is:
Φfinal=N⋅B⋅A⋅cos(180°)=200⋅0.8⋅(70×10−4)⋅(−1)
Φfinal=−1.12 Wb
Step 3: Calculate Change in Magnetic Flux
The change in magnetic flux (ΔΦ) is given by:
ΔΦ=Φfinal−Φinitial
ΔΦ=−1.12−1.12=−2.24 Wb
Step 4: Calculate Induced EMF
The induced EMF (ε) can be calculated using Faraday's law of electromagnetic induction:
ϵ=−ΔΦΔt
Substituting the values:
ϵ=−−2.240.1=2.240.1=22.4 V
Final Answer
The induced EMF in the coil is 22.4 V.
---
- Number of turns (N) = 200
- Area of the coil (A) = 70 cm² = 70 × 10⁻⁴ m² (conversion to m²)
- Magnetic field strength (B) = 0.8 Wb/m²
- Angle of rotation (θ) = 180°
- Time taken for rotation (t) = 0.1 s
The magnetic flux (Φ) through the coil is given by the formula:
Φ=N⋅B⋅A⋅cos(θ)
- When the coil is perpendicular to the magnetic field, θ = 0°.
- Thus, cos(0°)=1.
- Therefore, the initial flux is:
Φinitial=N⋅B⋅A⋅cos(0°)=200⋅0.8⋅(70×10−4)
Φinitial=200⋅0.8⋅0.007=1.12 Wb
- After rotating 180°, the angle becomes θ = 180°.
- Thus, cos(180°)=−1.
- Therefore, the final flux is:
Φfinal=N⋅B⋅A⋅cos(180°)=200⋅0.8⋅(70×10−4)⋅(−1)
Φfinal=−1.12 Wb
The change in magnetic flux (ΔΦ) is given by:
ΔΦ=Φfinal−Φinitial
ΔΦ=−1.12−1.12=−2.24 Wb
The induced EMF (ε) can be calculated using Faraday's law of electromagnetic induction:
ϵ=−ΔΦΔt
Substituting the values:
ϵ=−−2.240.1=2.240.1=22.4 V
The induced EMF in the coil is 22.4 V.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - IMPECCABLE
52 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - ENABLE
50 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - IN-CHAPTER EXERCISE-G
10 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)
89 Videos
Similar Questions
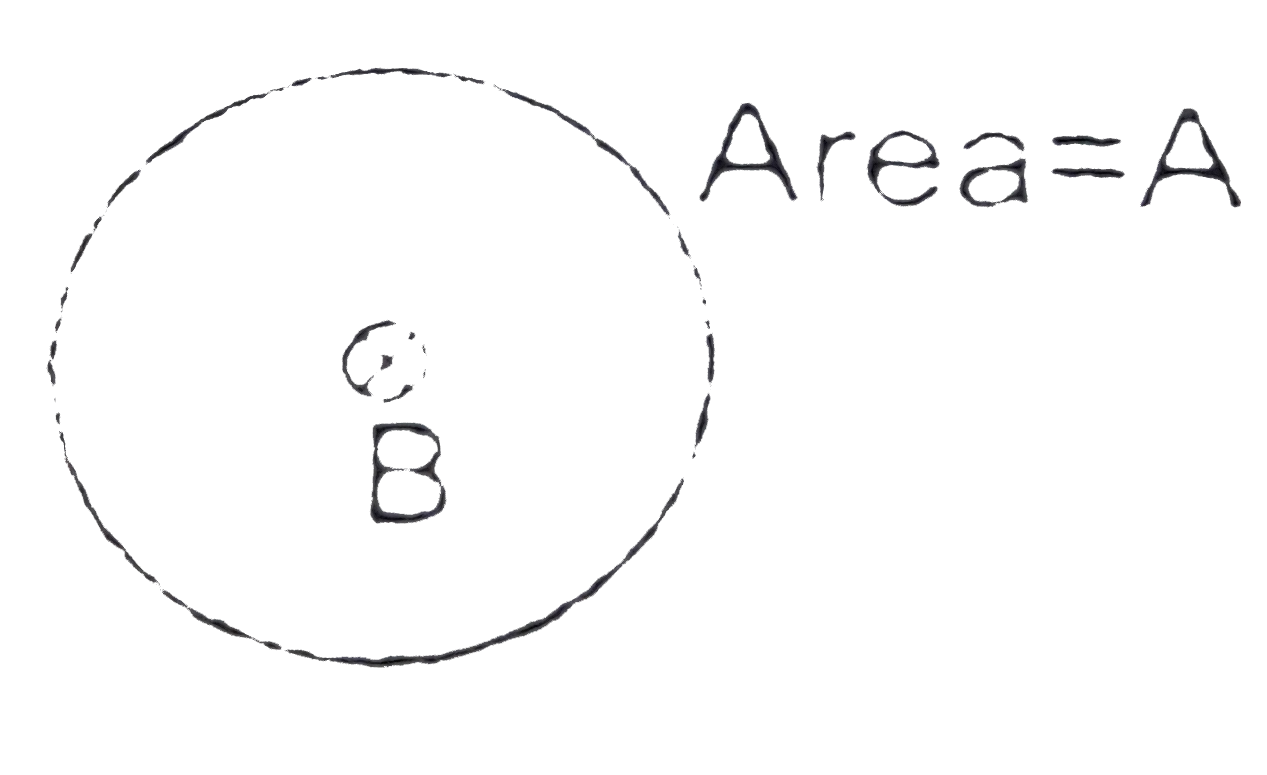
Find the emf induced in the coil shown in figure.The magnetic field is perpendicular to the plane of the coil and is constant.
View SolutionA coil has 2000 turns and area of 70cm2. The magnetic field perpendicular to the plane of the coil is 0.3Wb/m2 and takes 0.1 sec to rotate through 1800. The value of the induced e.m.f. will be
View Solution
Knowledge Check
A coil of area 0.4m2 has 100 turns. A magnetic field of 0.04 Wb m−2 is acting normal to the coil surface. If this magnetic field is reduced to zero in 0.01 s, then the induced emf in the coil is
A160VB250 VC270 VD320 V
A coil of area 500 cm2 having 1000 turns is put perpendicular to a magnetic field of intensity 4×10−5T. if it is rotated by 180∘ in 0.1 s, the induced emf produced is
View Solution1. A coil of area 2cm2 with turns 500 is placed in a magnetic field of 0.5 T with its plane parallel to field. The flux linked with the coil is
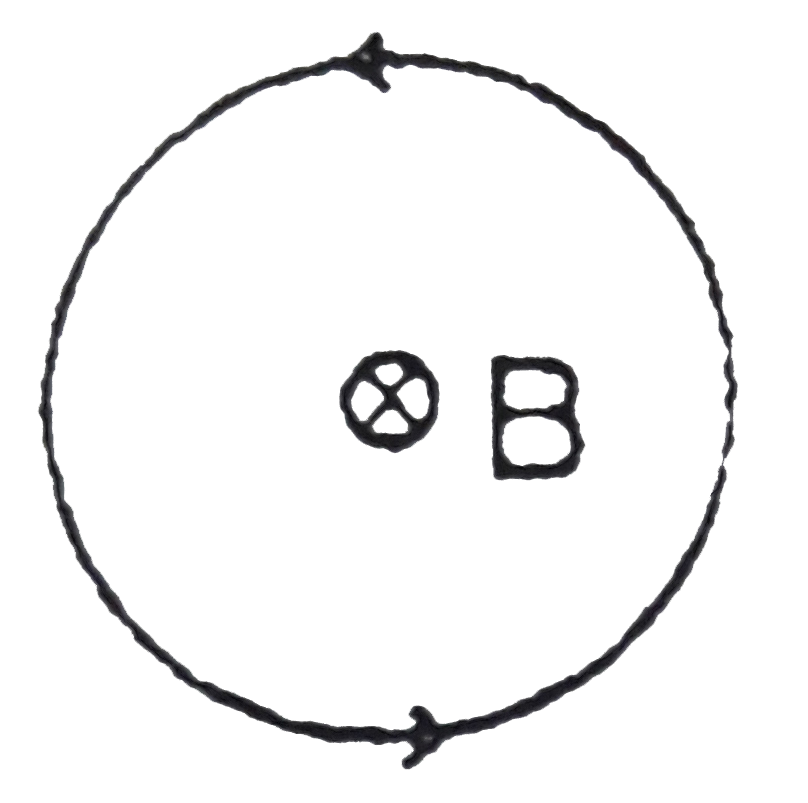
View SolutionFind the direction of induced current in the coil shown in figure.Magnetic field is perpendicular to the plane of coil and it is increasing with time.
View SolutionA circular coil of area 8 m2 and number of turns 20 is placed in a magnetic field of 2T with its plane perpendicular to it. It is rotated with an angular velocity of 20 rev/s about its natural axis. The emf induced is
View SolutionA circular coil of area 8m2 and number of turns 20 is placed in a magnetic field of 2T with its plane perpendicular to it. It is rotated with an angular velocity of 20rev/s about its natural axis. The emf induced is
View SolutionA rectangular copper coil is placed in a uniform magnetic field of induction 40 mT with its plane perpendicular to the field. The area of the coil is shrinking at a constant rate of 0.5m2s−1. The emf induced in the coil is
View SolutionA coil of area 5cm2 and having 20 turns is placed in a uniform magnetic field of 103 gauss. The normal to the plane of the coil makes an angle of 60∘ with the magnetic field. The flux in Maxwell through the coil is:
View Solution