A prism of a certain angle deviates the red and blue rays by 8∘ and 12∘ respectively. Another prism of the same angle deviates the red and blue rays by 10∘ and 14∘ respectively. The prisms are small angled and made of different materials. The dispersive powers of the materials of the prisms are in the ratio
5:6
9:11
6:5
11:9
The correct Answer is:C
To solve the problem, we need to find the dispersive powers of two prisms made of different materials based on the given deviations for red and blue rays.
1. Understand the Formula for Dispersive Power:
The dispersive power (ω) of a prism is given by the formula:
ω=ΔB−ΔRΔavg
where:
- ΔB = deviation for blue light
- ΔR = deviation for red light
- Δavg = average deviation
2. Calculate for the First Prism:
- Given deviations:
- ΔB=12∘ (for blue)
- ΔR=8∘ (for red)
- Calculate the average deviation:
Δavg=ΔB+ΔR2=12+82=10∘
- Now, substitute into the dispersive power formula:
ω1=12−810=410=25
3. Calculate for the Second Prism:
- Given deviations:
- ΔB=14∘ (for blue)
- ΔR=10∘ (for red)
- Calculate the average deviation:
Δavg=ΔB+ΔR2=14+102=12∘
- Now, substitute into the dispersive power formula:
ω2=14−1012=412=13
4. Find the Ratio of Dispersive Powers:
- Now we need to find the ratio of the dispersive powers of the two prisms:
ω1ω2=2513=25×31=65
- Therefore, the ratio of the dispersive powers is:
ω1:ω2=6:5
Final Answer:
The dispersive powers of the materials of the prisms are in the ratio 6:5.
---
The dispersive power (ω) of a prism is given by the formula:
ω=ΔB−ΔRΔavg
where:
- ΔB = deviation for blue light
- ΔR = deviation for red light
- Δavg = average deviation
- Given deviations:
- ΔB=12∘ (for blue)
- ΔR=8∘ (for red)
- Calculate the average deviation:
Δavg=ΔB+ΔR2=12+82=10∘
- Now, substitute into the dispersive power formula:
ω1=12−810=410=25
- Given deviations:
- ΔB=14∘ (for blue)
- ΔR=10∘ (for red)
- Calculate the average deviation:
Δavg=ΔB+ΔR2=14+102=12∘
- Now, substitute into the dispersive power formula:
ω2=14−1012=412=13
- Now we need to find the ratio of the dispersive powers of the two prisms:
ω1ω2=2513=25×31=65
- Therefore, the ratio of the dispersive powers is:
ω1:ω2=6:5
The dispersive powers of the materials of the prisms are in the ratio 6:5.
Topper's Solved these Questions
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - EFFICIENT
49 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
- RAY OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- REVISION TEST-15 JEE - 2020BOOK - VMC MODULES ENGLISHCHAPTER - REVISION TEST-15 JEE - 2020EXERCISE - PHYSICS
25 Videos
Similar Questions
Two small angled prisms A and B deviate the blue rays by 6∘ and 8∘ and the red rays by 4∘ and 6∘ respectively. Which prism has a greater dispersive power ?
View SolutionCompare the dispersive powers of two prisms if one of them deviates the blue and red rays through 10∘ and 6∘ respectively and the second prism through 8∘ and 4.5∘
View SolutionFor a small angled prism, angle of minimum deviation (δ) varies with the refractive index of the prism as shown in the graph
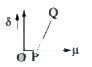
View SolutionThe difference between angle of minimum deviation of violet and red rays in the spectrum of white light from a prism is 2∘. If angle of minimum deviation of mean ray is 48∘, the dispersive power of material of the prism is
View SolutionIf the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
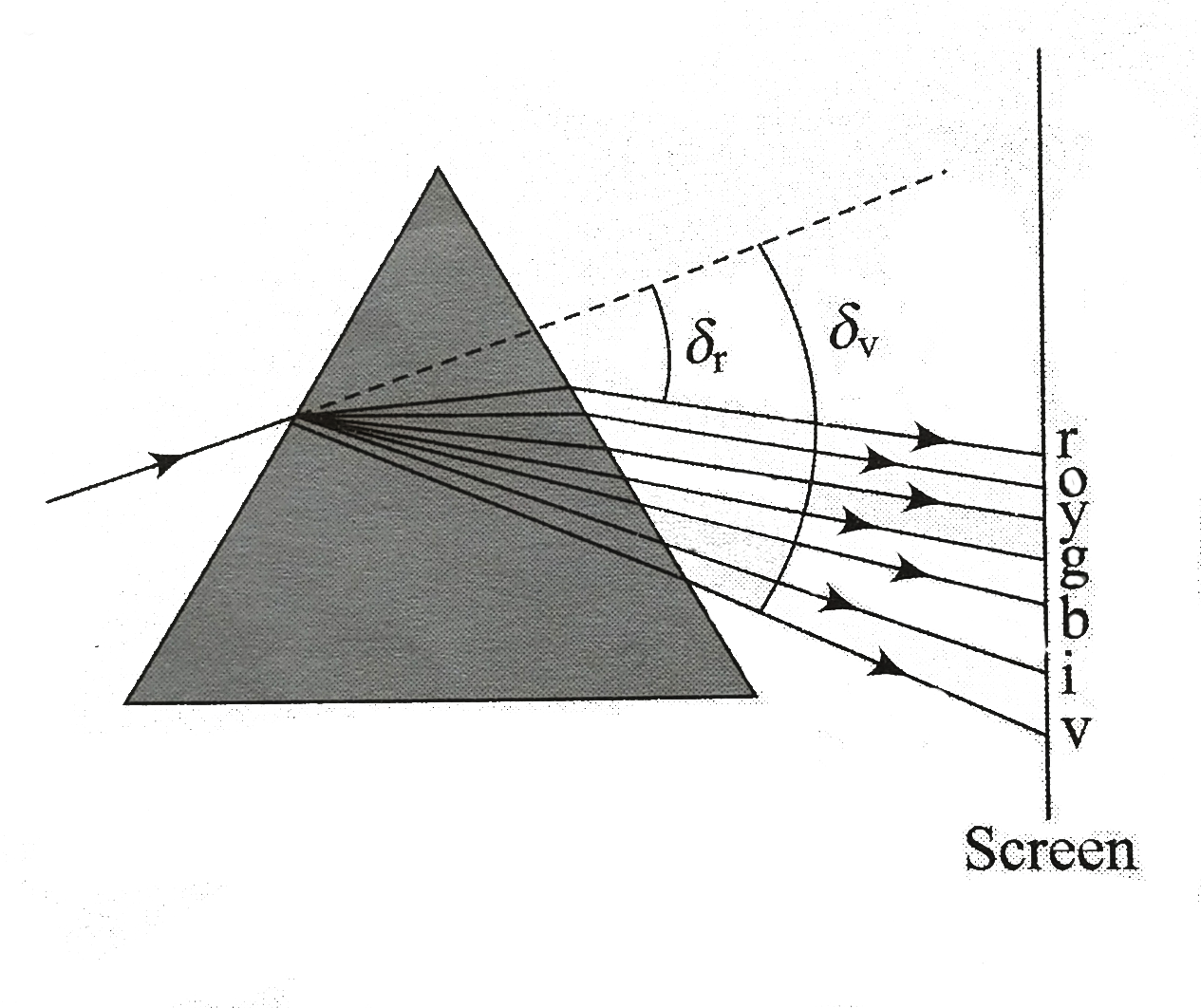
View SolutionA thin prism of angle A=6∘ produces a deviation d=3∘. Find the refractive index of the material of prism.
View SolutionThe refracting angle of a prism is A, and refractive index of the material of the prism is cot(A2). The angle of minimum deviation is
View SolutionThe angle of minimum deviation produced by an equilateral prism is 46∘ The refractive index of material of the prism.
View SolutionWhite light is passed through a prism of angle 5∘. If the refractive indices for red and blue colours are 1.641 and 1.659 respectively, calculate the angle of deviation between them, also calculate the dispersive power.
View SolutionIf the critical angle for the material of a prism is C, and the angle of the prism is A, then there will be no emergent ray when
View Solution
