If the path difference between the interfering waves is nλ, then the fringes obtained on the screen will be
dark
bright
coloured
white
The correct Answer is:B
To solve the problem, we need to analyze the relationship between the path difference of two interfering waves and the resulting interference pattern on the screen.
1. Understanding Path Difference:
The path difference between two interfering waves is given as nλ, where n is an integer and λ is the wavelength of the waves.
2. Relating Path Difference to Phase Difference:
The phase difference ϕ between the two waves can be calculated using the formula:
ϕ=2πλΔx
where Δx is the path difference. Substituting Δx=nλ:
ϕ=2πλ(nλ)=2nπ
3. Determining the Resultant Amplitude:
The resultant amplitude R at the point of interference can be calculated using the formula:
R=√A2+B2+2ABcosϕ
where A and B are the amplitudes of the two waves. Substituting ϕ=2nπ:
R=√A2+B2+2ABcos(2nπ)
4. Evaluating the Cosine Term:
The cosine of 2nπ is always equal to 1 (since cos(2nπ)=1):
R=√A2+B2+2AB⋅1=√A2+B2+2AB=√(A+B)2=A+B
5. Interpreting the Resultant Amplitude:
Since the resultant amplitude R is equal to A+B, this indicates that the amplitude is at its maximum.
6. Relating Amplitude to Intensity:
The intensity I of the interference pattern is proportional to the square of the amplitude:
I∝R2
Therefore, if the amplitude is maximum, the intensity is also maximum, resulting in a bright fringe on the screen.
Conclusion:
Since the path difference is nλ, the fringes obtained on the screen will be bright fringes.
The path difference between two interfering waves is given as nλ, where n is an integer and λ is the wavelength of the waves.
The phase difference ϕ between the two waves can be calculated using the formula:
ϕ=2πλΔx
where Δx is the path difference. Substituting Δx=nλ:
ϕ=2πλ(nλ)=2nπ
The resultant amplitude R at the point of interference can be calculated using the formula:
R=√A2+B2+2ABcosϕ
where A and B are the amplitudes of the two waves. Substituting ϕ=2nπ:
R=√A2+B2+2ABcos(2nπ)
The cosine of 2nπ is always equal to 1 (since cos(2nπ)=1):
R=√A2+B2+2AB⋅1=√A2+B2+2AB=√(A+B)2=A+B
Since the resultant amplitude R is equal to A+B, this indicates that the amplitude is at its maximum.
The intensity I of the interference pattern is proportional to the square of the amplitude:
I∝R2
Therefore, if the amplitude is maximum, the intensity is also maximum, resulting in a bright fringe on the screen.
Since the path difference is nλ, the fringes obtained on the screen will be bright fringes.
Topper's Solved these Questions
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - EFFICIENT
49 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
- RAY OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- REVISION TEST-15 JEE - 2020BOOK - VMC MODULES ENGLISHCHAPTER - REVISION TEST-15 JEE - 2020EXERCISE - PHYSICS
25 Videos
Similar Questions
The path difference between two interfering waves of equal intensities at a point on the screen is λ/4. The ratio of intensity at this point and that at the central fringe will be
View SolutionIn a double slit experiment, at a certain point on the screen the path difference between the two interfering waves is 13rd of a wavelength. The ratio of the intensity of light at that point to that at the centre of a bright fringe is:
View SolutionIn Young.s double slit experiment, what is the path difference between the two light waves forming 5th bright band (fringe) on the screen ?
View SolutionWaves emitted by two identical sources produces intensity of K unit at a point on screen where path difference between these waves is λ. Calculate the intensity at that point on screen at which path difference is λ4
View SolutionWhich of the following graphs best represent the variation of phase difference between he interferring waves in a double slit experiment with the distance from the central maximum?
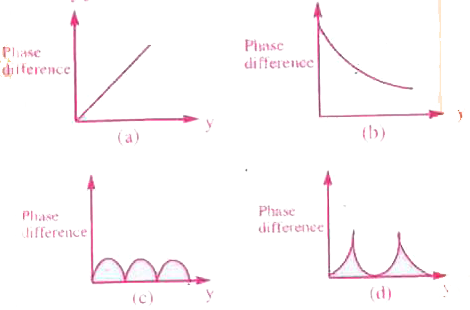
View SolutionIn Young's double-slit experiment, the path difference between two interfering waves at a point on the screen is 13.5 times the wavelength. The point is
View SolutionMaximum intensity in YDSE is I0. Find the intensity at a
point on the screen where
(a) The phase difference between the two interfering beams is π3.
(b) the path difference between them is λ4.
View SolutionMaximum intensity in YDSE is I0. Find the intensity at a
point on the screen where
(a) The phase difference between the two interfering beams is π3.
(b) the path difference between them is λ4.
View SolutionA : Light from two coherent sources is reaching the screen. If the path difference at a point on the screen for yellow light is 3λ/2, then the fringe at the point will be coloured.
R : Two coherent sources always have same phase relationship at any point on the screen.
View SolutionThe path difference between two interfering waves at a point on the screen is λ/6from central maximum. The ratio of intensity at this point and that at the central fringe will be
View Solution
