An object placed in front of a plane mirror is displaced by 0.4 m along a straight line at an angle of 30∘ to mirror plane. The change in the distance between the object and its image is:
0.20 m
0.40 m
0.25 m
0.80 m
The correct Answer is:B
To solve the problem of finding the change in distance between an object and its image after the object is displaced, we can follow these steps:
1. Understand the Initial Setup:
- Let the initial distance of the object from the mirror be u.
- The distance between the object and its image in a plane mirror is given by d=di−do=2u.
2. Displacement of the Object:
- The object is displaced by 0.4m at an angle of 30∘ to the mirror plane.
3. Calculate the Horizontal and Vertical Components of Displacement:
- The horizontal component of the displacement (along the mirror plane) can be calculated using:
Δx=0.4cos(30∘)
- The vertical component is not relevant for the distance between the object and its image.
4. Calculate the Horizontal Component:
- Using cos(30∘)=√32:
Δx=0.4×√32=0.4×0.866=0.3464m
5. New Distance Between Object and Image:
- The new distance between the object and its image after displacement becomes:
d′=2(u+Δx)=2u+2Δx
6. Change in Distance:
- The change in distance Δd is given by:
Δd=d′−d=(2u+2Δx)−2u=2Δx
- Substituting Δx:
Δd=2×0.3464≈0.6928m
7. Final Result:
- The total change in distance between the object and its image is approximately 0.6928m.
- Let the initial distance of the object from the mirror be u.
- The distance between the object and its image in a plane mirror is given by d=di−do=2u.
- The object is displaced by 0.4m at an angle of 30∘ to the mirror plane.
- The horizontal component of the displacement (along the mirror plane) can be calculated using:
Δx=0.4cos(30∘)
- The vertical component is not relevant for the distance between the object and its image.
- Using cos(30∘)=√32:
Δx=0.4×√32=0.4×0.866=0.3464m
- The new distance between the object and its image after displacement becomes:
d′=2(u+Δx)=2u+2Δx
- The change in distance Δd is given by:
Δd=d′−d=(2u+2Δx)−2u=2Δx
- Substituting Δx:
Δd=2×0.3464≈0.6928m
- The total change in distance between the object and its image is approximately 0.6928m.
Topper's Solved these Questions
- RAY OPTICS AND WAVE OPTICS Book:VMC MODULES ENGLISHChapter:RAY OPTICS AND WAVE OPTICS Exercise:IMPECCABLE
Explore 58 Videos
- RAY OPTICS AND WAVE OPTICS Book:VMC MODULES ENGLISHChapter:RAY OPTICS AND WAVE OPTICS Exercise:ENABLE
Explore 50 Videos
- RAY OPTICS Book:VMC MODULES ENGLISHChapter:RAY OPTICS Exercise:IN-CHAPTER EXERCISE-J
Explore 10 Videos
- REVISION TEST-15 JEE - 2020Book:VMC MODULES ENGLISHChapter:REVISION TEST-15 JEE - 2020Exercise:PHYSICS
Explore 25 Videos
Similar Questions
An object is placed in front of a convex mirror at a distance of 50cm. A plane mirror is introduced covering the lower half of the convex mirror. If the distance between the object and the plane mirror is 30cm, it is found that there is no parallax between the images formed by the two mirrors. What is the radius of curvature of the convex mirror?
View SolutionAn insect is sitting in front of a plane mirror at a distance 1 m from it.
a. Where is the image of the insect formed?
b.What is the distance between the insect and its image?
View Solution
Knowledge Check
An object is placed 100 cm from a plane mirror how far is the image from the object?
A50cmB100cmC200cmD300cm
The minimum distance between the object and its real image for concave mirror is
View SolutionAn object is at a distance of 0.5m in front of a plane mirror. Distance between the object and image is
View SolutionAn object is at a distance of 1.2m in front of a plane mirrorr. Distance between the object and image is
View SolutionAn object is initially at a distance of 100 cm from a plane mirror . It the mirror approaches the object at a speed of 5 cm/s . Then after 6 s the distance between the object and its image will be -
View SolutionA tank contains a transparent liquid of refractive index μ. The bottom of the tank is a plane mirror as shown. A person at P looks at an object O and its image in the mirror. The distance between the object and its image in the mirror as preceived by the person is
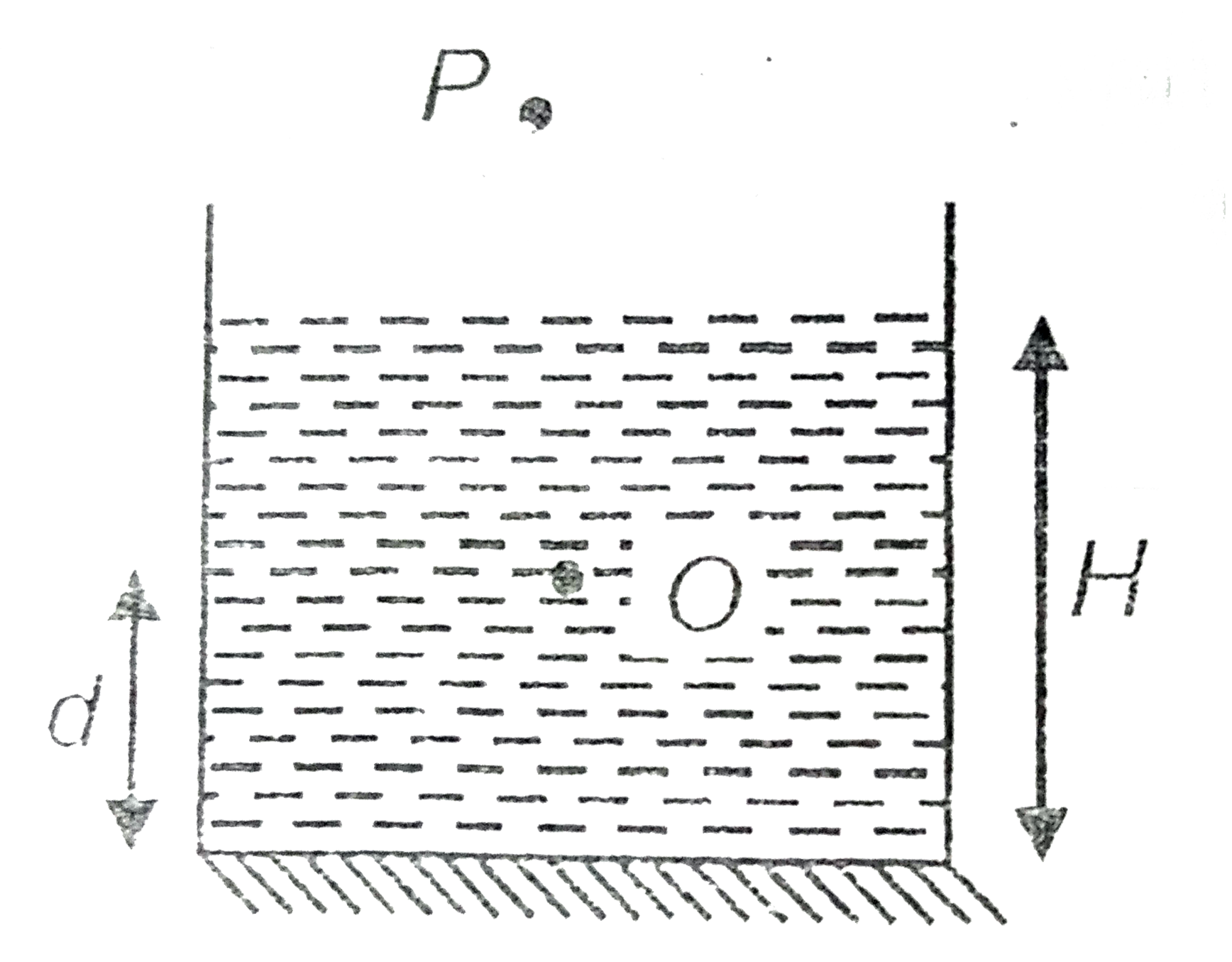
View SolutionAn object is at a distance 25 cm in front of a plane mirror. The mirror is shifted 5 cm away from the object. Find: (i) the new distance between theobject ad its image, and (ii) the distance between the two positions of the image.
View Solution
If an object moves towards a plane mirror with a speed v at an angle θ to the perpendicular to the plane of the mirror, find the relative velocity between the object and the image.
View Solution