In an irreversible process taking place at constant T and P and in which only pressure-volume work is being done, the change in Gibbs free energy (dG) and the change in entropy (dS) satisfy the criteria
(△S)VE=0,(△G)T,P=0
(△S)V,E=0,(△G)T,P>0
(△S)V,E<0,(△G)T,P<0
(△S)V,E>0,(△G)T,P<0
The correct Answer is:D
To solve the question regarding the relationship between the change in Gibbs free energy (dG) and the change in entropy (dS) for an irreversible process occurring at constant temperature (T) and pressure (P), we can follow these steps:
1. Understanding the Process:
- We are dealing with an irreversible process that occurs at constant temperature and pressure. In such processes, the system is not in equilibrium, and spontaneous changes occur.
2. Criteria for Spontaneity:
- For a process to be spontaneous at constant temperature and pressure, the change in Gibbs free energy (ΔG) must be negative. This is a fundamental criterion for spontaneity:
ΔG<0
3. Entropy Change:
- In an irreversible process, the change in entropy (ΔS) of the universe (system + surroundings) must be positive. This means that the entropy of the system may increase, but the total change in entropy must be greater than zero:
ΔS>0
4. Relationship Between ΔG and ΔS:
- The relationship between the change in Gibbs free energy and the change in entropy at constant temperature and pressure can be expressed using the equation:
ΔG=ΔH−TΔS
- For spontaneous processes, since ΔG < 0 and ΔS > 0, we can infer that the term TΔS must be greater than ΔH for ΔG to be negative.
5. Conclusion:
- Therefore, in an irreversible process at constant temperature and pressure, we conclude:
- ΔG < 0 (indicating spontaneity)
- ΔS > 0 (indicating an increase in entropy)
Summary:
In an irreversible process at constant temperature and pressure, the change in Gibbs free energy (ΔG) is less than zero, and the change in entropy (ΔS) is greater than zero.
---
- We are dealing with an irreversible process that occurs at constant temperature and pressure. In such processes, the system is not in equilibrium, and spontaneous changes occur.
- For a process to be spontaneous at constant temperature and pressure, the change in Gibbs free energy (ΔG) must be negative. This is a fundamental criterion for spontaneity:
ΔG<0
- In an irreversible process, the change in entropy (ΔS) of the universe (system + surroundings) must be positive. This means that the entropy of the system may increase, but the total change in entropy must be greater than zero:
ΔS>0
- The relationship between the change in Gibbs free energy and the change in entropy at constant temperature and pressure can be expressed using the equation:
ΔG=ΔH−TΔS
- For spontaneous processes, since ΔG < 0 and ΔS > 0, we can infer that the term TΔS must be greater than ΔH for ΔG to be negative.
- Therefore, in an irreversible process at constant temperature and pressure, we conclude:
- ΔG < 0 (indicating spontaneity)
- ΔS > 0 (indicating an increase in entropy)
In an irreversible process at constant temperature and pressure, the change in Gibbs free energy (ΔG) is less than zero, and the change in entropy (ΔS) is greater than zero.
Topper's Solved these Questions
- THERMODYNAMICS & THERMOCHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - THERMODYNAMICS & THERMOCHEMISTRYEXERCISE - Efficient
48 Videos
- THERMODYNAMICS & THERMOCHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - THERMODYNAMICS & THERMOCHEMISTRYEXERCISE - Impeccable
48 Videos
- THERMODYNAMICS & THERMOCHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - THERMODYNAMICS & THERMOCHEMISTRYEXERCISE - Impeccable
48 Videos
- THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE)
44 Videos
Similar Questions
As per second law of thermodynamics a process taken place spontaneously if and only if the entropy of the universe increases due to the process. Change in entropy is given by
ΔS=QrevT
What is the change in entropy of the universe due to the following reaction occuring at 27∘C ?
A+2B→C+2D,ΔH=+1.8kJmol−1
Molar entropy values of A, B, C and D are 1,2,3 and 4JK−1mol−1 respectively.
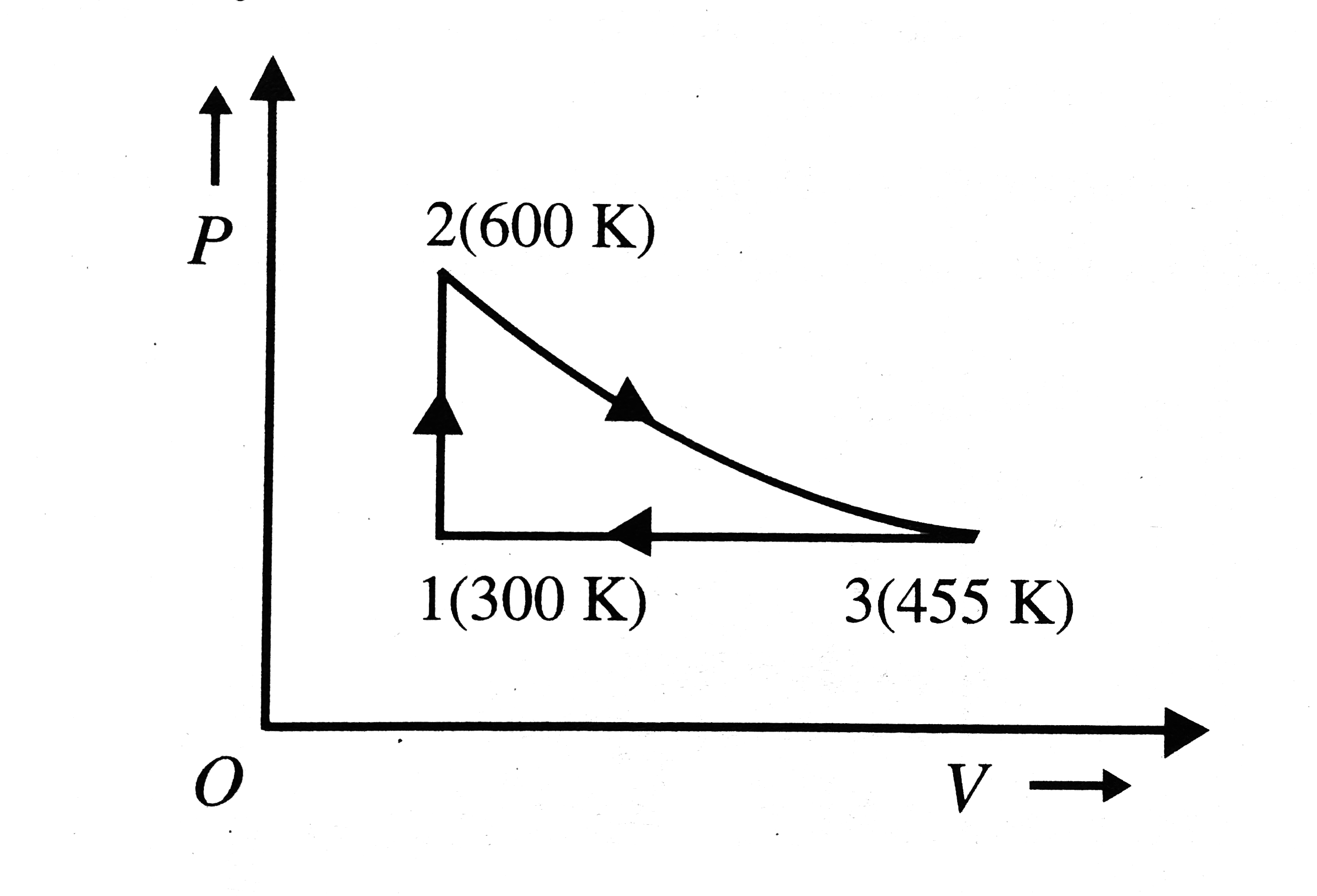
View SolutionA reversible heat engine carries 1 mole of an ideal monatomic gas around the cycle 1-2-3-1. Process 1-2 takes place at constant volume, process 2-3 is adiabatic, and process 3-1 takes place at constant pressure. Complete the values for the heat ΔQ, the change in internal energy ΔU, and the work done Δ, for each of the three processes and for the cycle as a whole.
View SolutionEntropy (S) is a thermodynamic variable like pressure P, volume V and temperature T. Entropy of a thermodynamic system is a measure of disorder of molecular motion. Greater is disorder , greater is entropy. Change in entropy of a thermodynamic system is the ratio of heat supplied to absolute temperature.In an adiabatic reversible process, entropy remains constant while in any irreversible process entropy increases. In nature the processes are irreversible , therefore entropy of universe is continuoulsy increasing.
After a long-long time, the energy available for work done will be :
View SolutionThe relationship between the free energy change (ΔG) and entropy change (ΔS) at constant temperature (T) si
View SolutionEntropy (S) is a thermodynamic variable like pressure P, volume V and temperature T. Entropy of a thermodynamic system is a measure of disorder of molecular motion. Greater is disorder , greater is entropy. Change in entropy of a thermodynamic system is the ratio of heat supplied to absolute temperature.In an adiabatic reversible process, entropy remains constant while in any irreversible process entropy increases. In nature the processes are irreversible , therefore entropy of universe is continuoulsy increasing.
The unit of entropy in S−I system is -
View SolutionEntropy (S) is a thermodynamic variable like pressure P, volume V and temperature T. Entropy of a thermodynamic system is a measure of disorder of molecular motion. Greater is disorder , greater is entropy. Change in entropy of a thermodynamic system is the ratio of heat supplied to absolute temperature.In an adiabatic reversible process, entropy remains constant while in any irreversible process entropy increases. In nature the processes are irreversible , therefore entropy of universe is continuoulsy increasing.
When milk is heated , its entropy :
View SolutionSelect correct statements:
S1 : For every chemical reaction at equilibrium , standard gibbs energy of reaction is zero
S2 : At constant temperature and pressure , chemical reactions are spontaneous in the direction of decreasing gibbs energy.
S3: Spontancity is related to change in entropy if universe.
View SolutionDependence of Spontaneity on Temperature:
For a process to be spontaneous , at constant temperature and pressure , there must be decrease in free energy of the system in the direction of the process , i.e. ΔGP.T<0.ΔGP.T=0 implies the equilibrium condition and ΔGP.T>0 corresponds to non- spontaneity.
Gibbs- Helmholtz equation relates the free energy change to the enthalpy and entropy changes of the process as : ΔGP.T=ΔH−TΔS ...(1)
The magnitude of ΔH does not change much with the change in temperature but the entropy factor TΔS change appreciably . Thus, spontaneity of a process depends very much on temperature.
For endothermic process, both ΔH and ΔS are positive . The energy factor, the first factor of equation, opposes the spontaneity whereas entorpy factor favours it. At low temperature the favourable factor TΔS will be small and may be less than ΔH,ΔG will have positive value indicated the nonspontaneity of the process. On raising temperature , the factor TΔS Increases appreciably and when it exceeds ΔH,ΔG would become negative and the process would be spontaneous .
For an expthermic process, both ΔH and ΔS would be negative . In this case the first factor of eq.1 favours the spontaneity whereas the second factor opposes it. At high temperature , when TΔS>ΔH,ΔG will have positive value, showing thereby the non-spontaneity fo the process . However , on decreasing temperature , the factor ,TΔS decreases rapidly and when TΔS<ΔH,ΔG becomes negative and the process occurs spontaneously. Thus , an exothermic process may be spontaneous at low temperature and non-spontaneous at high temperature.
The enthalpy change for a certain rection at 300 K is −15.0 K cal mol−1 . The entropy change under these conditions is −7.2 cal K−1mol−1 . The free energy change for the reaction and its spontaneous/ non-spontaneous character will be
View SolutionA diatomic gas is heated at constant pressure. If 105J of heat is given to the gas, find (a) the change in internal energy of the gas (b) the work done by the gas.
View SolutionDuring a process work equivalent to 400J is done on a system, which gives out of 125J of energy. The change in internal energy is
View Solution
