A current carrying conductor is in the form of a sine curve as shown, which carries current I. If the equation of this curve is Y=2sin(πxL) and a uniform magnetic field B exists in space.
The correct Answer is:xcosx−sinxx2
dydx=x.ddx(sinx)−sinx.1x2 Quotient Rule
=xcosx−sinxx2
Similar Questions
A coil carrying electric current is placed in uniform magnetic field
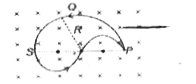
View SolutionA semicircular wire PQS of radius R is connected to a wire bent in the form of a sine curve to form a closed loop as shown in the figure. If the loop carries a current I and is placed in a uniform magnetic field B, then the total force acting on the sine curve is
View SolutionA current carrying loop in a uniform magnetic field will experience
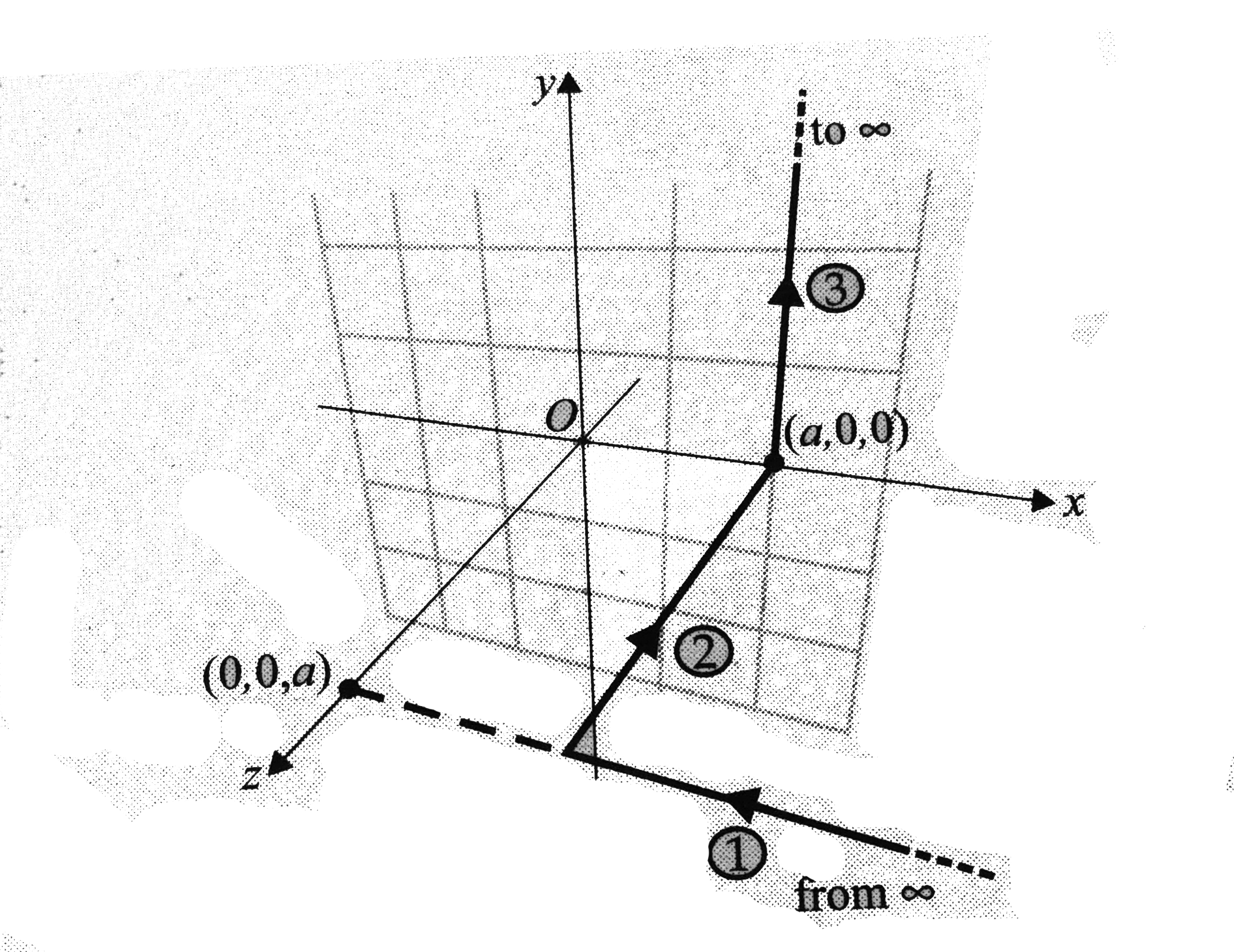
View SolutionAn infinite current carrying conductor is bent into three segment (1), (2) and (3) as shown in Fig. If it carries a current i, find the magnetic induction at the origin.
View Solutionthe force on current carrying wire placed in uniform magnetic field B as shown in figure is
View SolutionA wire carrying current l, bent in the form of a quarter circle is held at rest on a smooth table with two threads as shown. A uniform magnetic field exists in the region direction into the plane select the correct alternatives
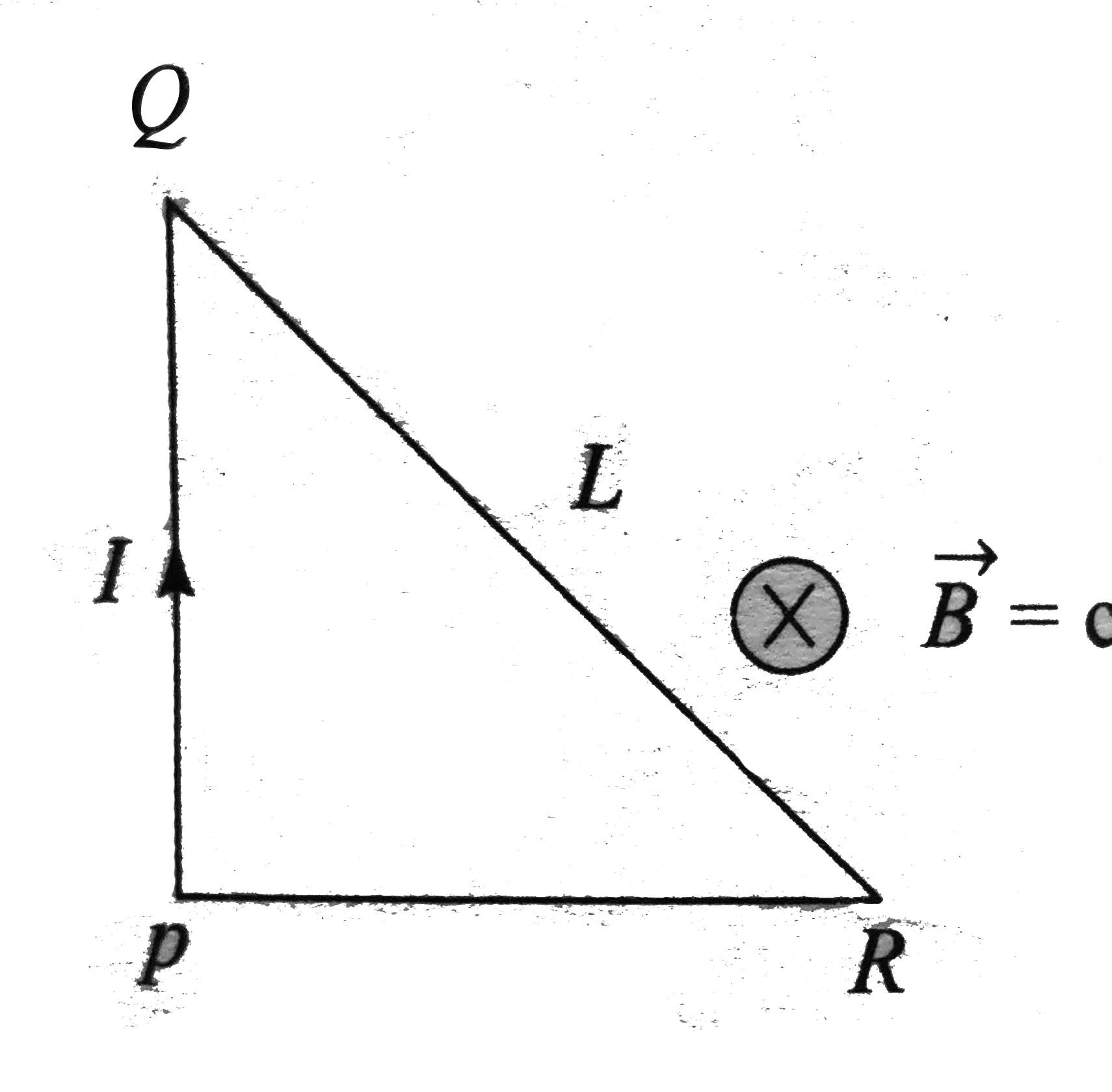
View SolutionThe rigid conducting thin wire frame carries an electric current I and this frame is inside a uniform magnetic field →B as shown in Fig. 1.146. Then,
View SolutionA current carrying wire AB of the length L is turned along a circle, as shown in figure. The magnetic field at the centre O.
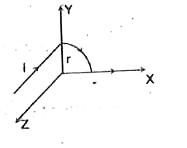
View SolutionShown in the figure is a conductor carrying a current i. the magnetic field at the origin is :
View SolutionA rectangular loop of dimensions (a×b) carries a current i. A uniform magnetic field →B=B0ˆi exists in space. Then :
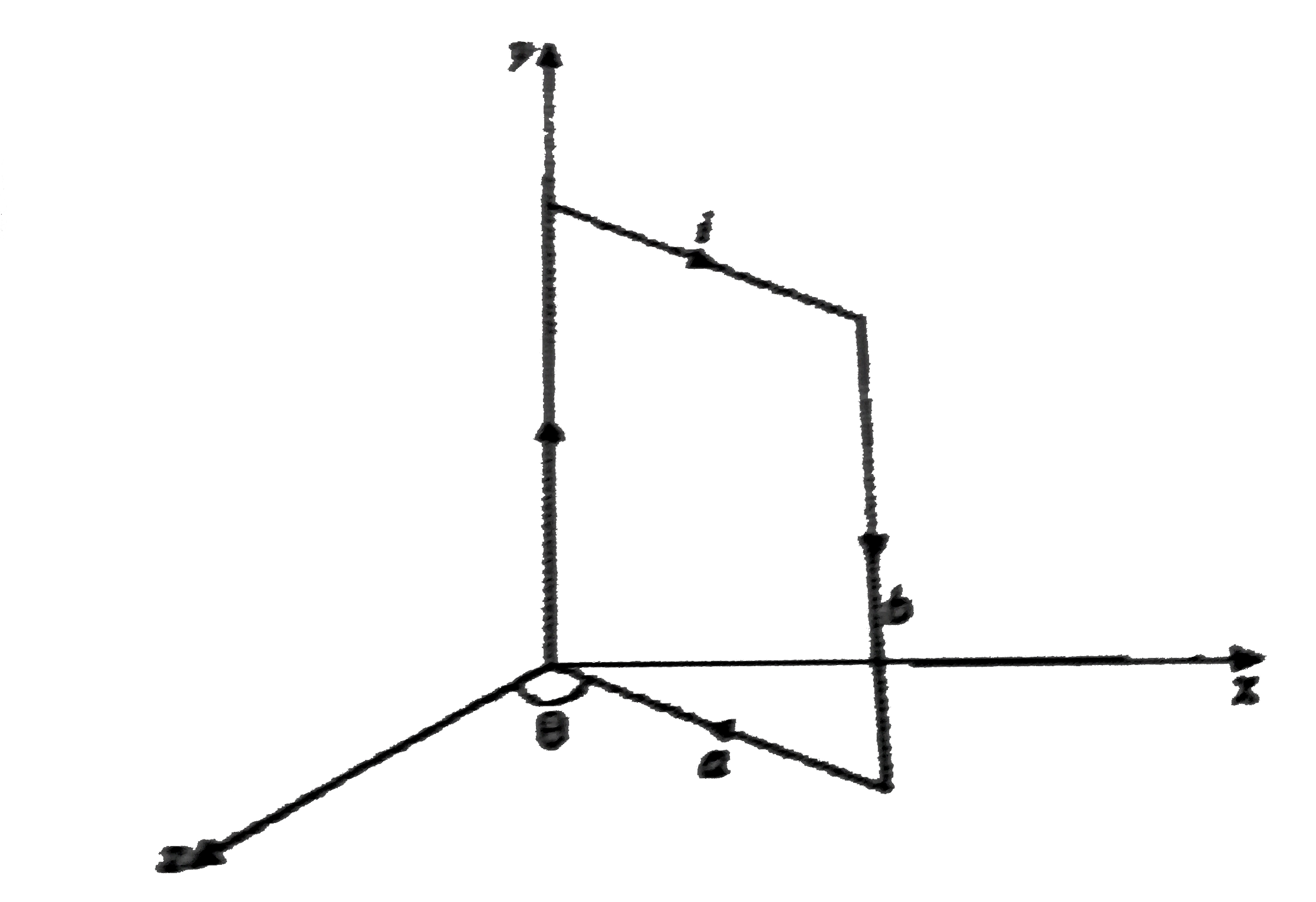View Solution